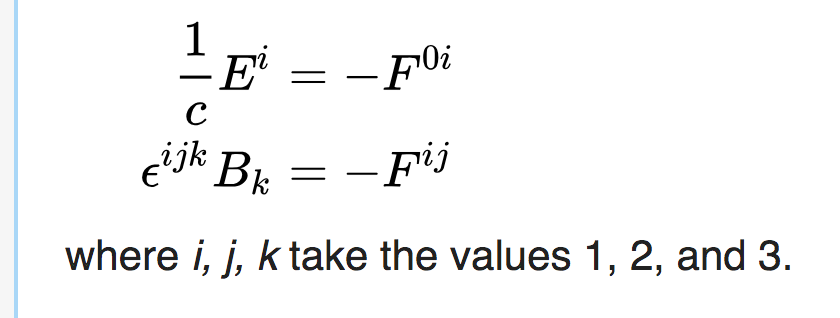Why is electric field $E^i = E_i$ instead of $E^i = - E_i$?

Question

Let us consider the Minkowski spacetim.

Generally, we know that when we lower or raise the index of the convariant or contravairant tensor, we need to use the metric $\eta^{\mu \nu}=\eta_{\mu \nu}=(+,-,-,-)$

Clearly, for the electromagnetic tensor $$ F_{0i}= - F^{0i} $$ However, if we define the electric field as $$ E_i \propto F_{0i} $$ as Wikipdia did

https://en.wikipedia.org/wiki/Electromagnetic_tensor#Relationship_with_the_classical_fields

we also get $$ E_i \propto F_{0i} =- F^{0i} $$ But from Wikipedia again, the same page says that $$ E^i \propto - F^{0i} $$

Thus, we get

$$ E^i = E_i \propto F_{0i} =- F^{0i} $$

My question is that why do $E^i = E_i$ instead of $E^i = - E_i$? Is there a reason we should not identify

$$E^i = \eta^{ia} E_{a} = - E_i?$$

This also relates to the fact whether we write the energy E as

E $\propto -(F_{0i} F^{0i}+...) =-(F_{0i} (- F_{0i})+...) = (F_{0i}^2+...)=((E_i)^2+...)$

E $=((E_i)^2+...)=((E^i)^2+...)=((E_i)(E^i)+...)$

Generally, we knew that

E $\propto((E_i)^2+(B_i)^2)=((E^i)^2+(B^i)^2)$

Question now: Generally, do we treat $E^i = E_i$ or $E^i = - E_i$ as a 1-vector, 1-(contra)vector or a 2-tensor?

Answer 1

Electric field is not a four vector. They are just three vectors which posses components along three spatial dimension. Their transformation to one form is defined by a $3\times 3$ identity matrix(Euclidean metric).$$E^i = \delta^{ia} E_{a} = E_i.$$ They are also components of elctromagnetic field tensor. Here $E^i$ depicts the $i^{th}$ component of electric field vector is same as the $-c F^{0i}$ th term of electromagnetic field tensor.

Answer 2

Why is $E^i = E_i$ instead of $E^i = - E_i$?

The fundamental reason would be that the electric and magnetic fields, $E$ and $B$ do not form four vectors. Rather they are three-dimensional vectors without a fourth component as explained here. The transformation between co- and contra- forms is identity transformation. This is due to different vectorial nature of the respective fields. The electric field is a polar vector (or true vector) because it changes the sign if coordinates are reversed, $\mathbf{r} \rightarrow \mathbf{-r}$. In contrast, the magnetic field given by

$$\mathbf{B}(\mathbf{r}) = \frac{1}{c} \int \dfrac{(\mathbf{r}-\mathbf{r'})\times \mathbf{J}(\mathbf{r})}{|\mathbf{r}-\mathbf{r'}|^3} \text dV'$$

remains unchanged against coordinate inversion since both $(r - r')$ and current density $J(r)$ change sign. The magnetic field is a pseudo-vector (or axial vector).

---

A detailed calculation of how it turns out to be the same in Minkowski space-time metric regardless of its form is shown here.

The signs of the components of the electromagnetic tensor $F^{\mu \nu}$ and $F_{\mu \nu}$ depend on the metric convention. However, the mixed tensor $F^\mu{}_\nu$ is independent of such a choice. Considering $c = 1$, we have

$$F^\mu{}_\nu=\left(\begin{array}{cccc}0&E_x&E_y&E_z\\E_x&0&B_z&-B_y\\E_y&-B_z&0&B_x\\E_z&B_y&-B_x&0\end{array}\right)$$

Here $\mu,\nu~\in~\{0,1,2,3\}$ and $i~\in~\{1,2,3\}$.

Defining $\eta_{\mu \nu} = \text{diag}(-1,+1,+1,+1) = \eta^{\mu \nu}$, we know $F_{\mu \nu} = \eta_{\mu \rho}F^\rho{}_{\nu}$ so we obtain $E_i = -F_{0i}$

Since, $F^{\mu \nu} = \eta^{\mu \rho}\eta^{\nu \lambda}F_{\rho \lambda}$, for $i \ne 0$ we obtain $$E^i = F^{0i} = \eta^{00}\eta^{i i}F_{0 i} = -F_{0i} = E_i$$

---

Now, it is fairly straight-forward to prove that if we use $\eta_{\mu \nu} = \text{diag}(+1,-1,-1,-1)$, the result will be $$E^i = -F^{0i} = - \eta^{00}\eta^{i i}F_{0i} = F_{0i} = E_i $$

---

In both the cases it turns out that $E_i = E^i$.

Answer 3

In Euclidean metric, such as standard 3D, the distinction between co- and contra is not useful and $E_i =E^i$. This can be confusing if the Minkowski metric is chosen as a space inversion. However, the alternative gives unintuitive minus signs (my opinion).

E is not a real 3D vector as its sign changes under time reversal. B also is not a true vector as its sign does not change under space inversion. It is called an axial vector. Another example of this is angular momentum.