0
$\begingroup$

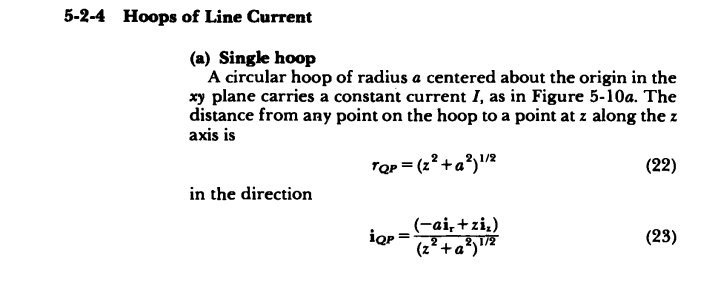
In the EMFT notes of MIT Course-ware, the derivation of the magnetic field due to a circular ring at its axis, using Biot-Savart's Law and the cylindrical coordinate system is done as follows,

enter image description here enter image description here enter image description here enter image description here

I am unable to understand how they calculate the $a_r$ vector component. Mainly the line, 'the radial vector changes direction as a function of $\phi$, being oppositely directed at $-\phi$, so that the total magnetic field due to the whole in the radial direction is zero.'

Why does the radial vector change direction isn't it always going to point outwards, thus in the positive direction? Can someone please explain this?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

There are actually two components of magnetic field due to an element on the ring. The radial itself means along the radius (radially outwards). It is perpendicular to axis of the ring for an element and it's direction depends on position of element on the ring. As you add the vectors the net will be zero. The other component is along the axis of the ring and they sum up to produce a net field along the axis of the ring.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I know. But why does the direction of the radial vector become negative as we move along the $\phi$ angle? I know that the field along the axis of the ring will remain. $\endgroup$
    – Mohammed Arshaan
    Commented May 13, 2019 at 13:01
  • $\begingroup$ The radial vector is along the radius of the ring. The radius vector changes direction at different points on ring $\endgroup$
    – Tojra
    Commented May 13, 2019 at 13:08
  • $\begingroup$ Can you give me a mathematical equation that can represent the change so that the math works out? $\endgroup$
    – Mohammed Arshaan
    Commented May 13, 2019 at 13:09
  • 1
    $\begingroup$ Suppose an element on the ring. The line joining that element to the point P on the axis makes angle $\theta $with the axis of the ring. (We have to determine field at the point P on the axis). The field due to element dB is perpendicular to that line. Then $\vec {dB}$ makes $\theta$ with vertical in the plane containing the line. So the radial field component is $dBcos \theta $and axial component is $dB sin \theta$. Note that$ \int dB cos \theta=0$ and $B_{net}=\int dB sin\theta$ $\endgroup$
    – Tojra
    Commented May 13, 2019 at 13:18
  • $\begingroup$ Refer to sketch a above $\endgroup$
    – R.W. Bird
    Commented Apr 24, 2020 at 17:42
0
$\begingroup$

Trying a simple answer to your question "Why does the radial vector change direction[,] isn't it always going to point outwards [...]?"

Yes, the radial component due to a current element on the ring always points outwards. But, you have to consider this "outward" direction with reference to coordinates in the $x$-$y$-plane. Consider the current element sitting on the positive side of the x-axis on the ring at point $(a,0,0)$. This will produce a radial component of the $B$-field at point $(0,0,z)$ in $-x$ direction. Whereas the current element sitting on the negative side of the $x$-axis at point $(-a,0,0)$ on the ring will produce a radial $B$-field component pointing in $+x$ direction. Both components have equal strengths and such cancel out. For every current element on the ring you will find a current element on the opposite side of the ring, and in any case the generated radial $B$-field components will cancel out for them.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.