What is the meaning of "matter" in physics?

Question

What is the meaning of matter in physics? By defining matter in terms of mass and mass in terms of matter in physics, are we not forming circular definitions? Please give a meaning of "matter" in Physics that circumvents this circularity.

Answer 1

What is the meaning of "matter" in physics?

It doesn't matter. Sometimes matter means "particles with rest mass". Sometimes matter means "anything that contributes to the stress-energy tensor". Sometimes matter means "anything made of fermions". And so on. There's no need to have one official definition of the word "matter", nothing about the physical theories depends on what we call the words.

Discussing this any further is just like worrying about whether a tomato is really a fruit or a vegetable. A cook doesn't care.

Answer 2

Please give a meaning of "matter" in Physics that circumvents this circularity.

In modern physics, mass is definitely not defined in terms of matter, and there is no circularity.

What we classically called mass was really a definition of its effects on and by other objects. We saw this as an intrinsic quality of an object, and definitional in that statement is that different types of matter had different masses - a 1m sphere of steel is more massive than a 1m sphere of water. We saw that as "obvious", different types of matter have different amounts of mass and that just makes sense.

Critically, the mass of an object defined its gravitational effect. That is, gravity was something caused by mass. This made mass "a thing", and objects with mass were "matter". Compare this to, for instance, a water wave. This clearly exists but is not of itself material, it's simply the water that was already there moving up and down. A body of water would cause a certain amount of gravity, and adding waves, which are "non-material", would not change that.

With the introduction of General Relativity in the early 20th century, this definition was seen to be incorrect. Earlier, Einstein concluded that E=mc^2, which means that mass is (although this terminology is very misleading) "another form of energy". Pondering this, a number of contemporary physicists helped develop GR, in which any and all energy causes gravity (though a mathematically complex system). So in GR, adding waves to water does increase its gravity, because the system has more energy. A shotput will have a certain gravity, and that will change if you heat it. Etc.

At this point, the link between mass and matter was broken. Mass was previously "that thing that causes gravity", but in GR, that was gone. Matter was previously "those things with mass", and while one could change that to be "rest mass" and still have a reasonable definition, we no longer needed it, there is no real need to have a definition of matter.

This is not a theoretical issue - it one "weights" an electron it will have higher "mass" if it's moving faster. Nothing in the electron changed, the change was what we thought we were actually measuring, not some intrinsic property of the object, but its total energy.

In QM, these definitions are further blurred. Particles, energy, fields, mass, all of it is "flexible" and not trivial to pin down. One can have a "mass-like concept" in QM which would be "the total internal energy of an object", but such a definition no longer serves a purpose. Another definition might be that matter is the class of particles called fermions, as opposed to bosons, but both cause gravity and have "mass like effects", because, under them, there's no real "mass".

This may sound confusing, but this true of most modern physics. Even simple things you think you understand, like "spinning", look very different today.

Answer 3

This response has been reorganized and had (a lot of) material added to it in response to a good point made in a comment. I have also appended some more in-depth information on mass and some other topics that I have a better understanding of now than I did when I first wrote this answer, and have added a third possible definition for matter. This is probably a lot more text than is necessary, but I figure "Why delete it?".

One good definition for mass is that it is a property of matter which determines it's motion according to certain equations. In classical mechanics, these are:

• Newton's Second Law: $F=ma$
• Newton's Law of Universal Gravitation $F_g=G\frac{m_1m_2}{r^2}$

(You can find explanations for these easily.)

Sometimes m in the first equation is called "inertial mass", and m in the second equation is called "gravitational mass", but the two are always proportional (i.e. equal with the right choice of the constant G)

Einstein's Theories of Special and General Relativity caused some reinterpretation of the concept of mass and introduced some new (more accurate) equations relating mass to motion. Νotably, it is necessary for inertial and gravitational mass to be equal for the General Theory of Relativity to be true.

For some definitions of mass, it might interest you to start looking at the Wikipedia page if you haven't already: https://en.wikipedia.org/wiki/Mass#Definitions

Given these mathematical definitions of mass, it becomes more reasonable to define matter as anything that has mass. However, since any collection of energy acts like it has mass to some extent in Relativity, it makes a bit more sense to define "matter" as "anything that has rest mass". "Rest mass" is mass as measured by an observer who is not moving relative to the object being measured. Many physicists think that "rest mass" should be considered the only kind of "mass", but it's also popular in explanations of Special Relativity to say that things gain mass when they move at higher speeds, because of the way the equations work. Particles like photons, that have no rest mass and thus always travel at the speed of light, are usually considered "massless", but they do have momentum (which they can transfer to and gain from other objects in collisions), react to gravity, and would suddenly act like they had mass if you stuck them inside a ball with mirrors on the inside so that they bounced around forever (though it is practically impossible to get any significant amount of mass this way, particularly since mirrors don't reflect totally efficiently; other difficult-to-verify facts are that black holes gain mass when you shine lights into them and that hotter things have more mass).

The idea that mass is "how much matter is in something" is an intuitive explanation to give people an idea of what sort of things have more mass than other things, or even have mass at all, based on the idea that people already have an intuitive understanding of what matter is. This was probably more important in the past because the vague idea of "matter" or "substance" has a long history in philosophy that goes back far before Newton's formulation of physics gave a definite mathematical notion of "mass". I don't know this kind of history, but I would guess that the explanation that mass is "how much matter is in something" would have been more popular among early physicists due to the fact that "mass" was a new concept and "matter" was an old one. It should also be noted that "weight" is also a much older concept than mass.

---

Another way matter is sometimes defined is that it has mass and takes up space. Particle physicists tend not to concern themselves too much with the macroscopic idea of "matter", at least not in the sense of needing to define it, but this idea of "taking up space" is related to one particle physics definition: "Fermions" follow the Pauli Exclusion Principle, which says that no two identical fermions can have all the same quantum numbers while they occupy the same quantum system (which corresponds roughly to our notion of "place"). This is why only two electrons can occupy the same orbital in an atom or molecule. "Bosons", on the other hand, can have any number of particles in the same place. The main example of a boson is the photon. Sometimes, matter is defined as fermions, or at least things made from fermions. This can, however, be rather problematic.

On larger scales most of what we think of as matter is made of atoms. Atoms tend to repel other atoms if they get too close. This property of ordinary matter leads to the "normal force" in classical physics, and is why particles in gases bounce off each other. Apparently, this is electrostatic repulsion that is only possible because of the Pauli Exclusion Principle. Apparently, if electrons did not obey this principle, any collection of adjacent atoms would merge into something with neutron-star-like density.* I'm not really sure how this works, but you might check out the following sources:

https://en.wikipedia.org/wiki/Electron_degeneracy_pressure
https://aip.scitation.org/doi/abs/10.1063/1.1705389
https://www.quora.com/Is-it-the-Pauli-exclusion-principle-or-electrostatic-forces-that-explain-why-I-do-not-fall-through-the-floor
http://www.pas.rochester.edu/~rajeev/phy246/lieb.pdf

One problem with this definition is that many combinations of fermions are not fermions. In fact, somewhere between 10% and 100% (by mass) of what is usually considered visible matter (as opposed to dark matter**) comes in the form of bosons (things that do not obey the Pauli Exclusion Principle) that are made of fermions. Protons, neutrons, and electrons are all fermions, but any collection of an even number of fermions acts like a boson whenever it acts like a single particle as a whole. (I'm not really sure how groups of particles act like single particles, but they do.)

For example, a neutral Helium-4 atom has two protons, two neutrons, and two electrons — an even number of fermions. This is why Helium-4 becomes a superfluid when it is cooled down to near absolute zero. Helium-3 has one less neutron than Helium-4, and is thus a fermion, when viewed as a single particle. Thus, Helium-3 does not become a superfluid until it reaches much colder temperatures than are necessary for Helium-4. At those temperatures, pairs of Helium-3 atoms come together in what's known as "Cooper pairs". Each of these Cooper pairs is made of an even number of fermions, and so acts like a boson.

Many other familiar atoms and molecules — such as most hydrogen, carbon, nitrogen, oxygen, water, glucose, methane, ammonia, carbon dioxide, neon, and argon — contain even numbers of fermions. (I'm ignoring a lot of solids because they're more complicated to deal to deal with. Many of them are also made of bosons.)

Given all this, it might seem odd that anyone would even consider this definition. The reason they sometimes do is that, when considering only elementary particles (particles that aren't known to be made of anything else), almost anything we might come up with to be "matter" is made of fermions,*** and it provides a convenient way of saying that light and various fields, such as electromagnetic fields, are not made of matter. (See the second "extra info" section below)

One potential way to save this definition is to only consider what elementary particles (see 2nd "extra info" section) something is made of rather than any particles that are actually combinations of particles. Another interesting fact is that, while even numbers of fermions can combine to make bosons, no number of bosons can combine to make a fermion unless there is at least one fermion involved as well. Thus, you could also define "matter" as "anything that is made of fermions on some level".

However, it's questionable whether any of this is a particularly good idea. I suspect that a large part of the reason this gained any credence is because it extends a notion that existed before Quantum Field Theory was developed. This notion was the idea that light, and probably mesons,**** were simply excitations in fields (that maybe sometimes looked like particles) and could be created and destroyed at will by just transferring energy; whereas protons, neutrons, and electrons were "really" particles of (possibly indestructable) matter. I think this notion comes from before quantum physics got started, when Maxwell explained how light could be waves in electromagnetic fields and people knew about atoms and were just starting to figure out the various parts they were made of. It was pretty thoroughly extinguished when Quantum Field Theory became established in the mid twentieth century and showed that all known particles can be described as excitations in fields that have values at every point in spacetime (at least in terms of the "wave functions" that probabilistically predict the outcomes of measurements). Any idea of particle-indestructibility was pretty far gone even before that when matter-antimatter annihilation and pair-production were discovered.

Given this understanding of particle physics, it might seem reasonable to say either that matter is a purely macroscopic phenomenon or that all types of particles, including photons, could count as matter. At least, this would make about as much sense as saying that matter was fermions, and still might exclude things like spacetime, energy (since that's something particles and parts of fields have, not a substance), and sound-waves (although I think there are quantum-particle-like descriptions of sound: look up "phonon" and maybe "quantum acoustics", but those are generally called "quasiparticles" rather than "real" particles).

*Neutron stars are, of course, also generally considered matter. They are not made of atoms (at least not mostly), but they are still "baryonic matter" in that they are made of protons and neutrons (and maybe also electrons). It's just that A: it's mostly neutrons because most of the electrons merged with the protons to form neutrons, and B: they're all grouped together like one enormous nucleus. The "quark matter" that may be in some of them does not have the quarks divided into baryons, but would still generally be considered "matter".

**Obviously, "dark matter" has the word "matter" in it. Since the only thing we "know" about dark matter is that it creates gravity (and therefore is usually supposed to have other properties that go with mass), this terminology does tend to go with the idea that matter = mass. There is an idea (which has been loosing favor) that "dark matter" is actually just something we don't understand about gravity, or maybe a fifth force, in which case people would probably find the label inappropriate, but if it were any kind of particle, and even if it was all black holes, people would probably consider the name pretty justified (although I can imagine an argument that black holes have mass but aren't made of "matter", since they're likely just stable configurations of spacetime from an outside perspective).

***Well, there is the issue of binding energy. For example, protons and neutrons are usually said to be "made of" quarks, which are fermions, but most of the mass comes from the binding energy holding the quarks together, which really isn't fermions, so you have to ask what "made of" means.

****Mesons are bosons "made of" two quarks each (one matter, one antimatter, whereas protons and neutrons are "baryons" made of three matter quarks each). Some mesons are involved in the force that holds protons and neutrons together in the nucleus, i.e., they "mediate" the "nuclear force" in the same way that photons "mediate" the electromagnetic force. They also all have mass.

---

The idea that "matter" means "fermions" is not particularly useful, for the reasons given above (and maybe others). In fact, having a distinction between matter and non-matter particles is kind of unnecessary in quantum theory. One situation where particle physicists do still talk about "matter", however, is in contrast to "antimatter". Matter and antimatter have the interesting property that when a particle of matter and a particle of antimatter meet, they can "annihilate" with each other to release energy. This energy must come in the form of another particle, usually a photon. This photon can be considered to neither matter nor antimatter. Alternatively, it might be considered to be either matter or antimatter or even both at once. This is similar to how zero is often considered neither positive nor negative, but you can also say that +0 and -0 are both valid representations of the same number.

Elementary particles are particles that are not known to be made of any smaller parts. All elementary fermions have antimatter equivalents. One type of elementary boson also comes in two forms (W+ and W-), which I believe behave like matter and antimatter, but there is no good way to decide which is matter and which is antimatter. The other 4 known boson-types are Z-bosons, photons, gluons, and Higgs bosons. Gluons come in 8 colors, but there's no good way to decide what is matter and what is antimatter, and the others all have the property of being neither matter nor antimatter, as far as I know.

If you look at the table of elementary particles in the 2nd "extra info section", you'll see that there is a strong correlation between particles having a matter-antimatter distinction and them having mass and being fermions. There are exceptions, though. The Z and Higgs bosons have mass but don't have this distinction (I don't think), and W boson is a non-fermion that I'm pretty sure has a matter-antimatter distinction, although I don't think people generally define which version is which, since it's not a stable particle either way and both appear regularly.

---

Extra Info About Mass

As an aside, the current theory of where mass comes from is two fold:

1. Everywhere in space is filled with something called the "Higgs Field". Elementary particles that have "intrinsic mass" sort of bump into this field constantly in a way that makes them seem to be going slower than the speed of light, which all particles naturally go at according to this theory, and this is what makes them need to be pushed to move around at different speeds relative to other things. It is also possible that some particles get their "intrinsic mass" from some other unknown source.

2. Most of the mass in atoms comes from a seemingly different process (which is technically the same process). According to Special Relativity, objects moving fast relative to you seem to have more mass because they seem to take more energy to push. Also, according to general relativity, how much spacetime curves (i.e., how much and what kind of gravity there is) depends on how much energy is in a part of spacetime (which is weird, since kinetic energy is different depending on the observer's velocity relative to the object, but maybe gravitational potential energy makes it less weird?). These effects do apply to the "intrinsic mass" of elementary particles like electrons and quarks (since that is energy), but most of the mass of atoms actually comes from what's called the "binding energy" of protons and neutrons, as well as of atomic nuclei as wholes.
   
   This is usually classified as "potential energy", but it can also be thought of (probably rather inaccurately) as the kinetic energy of the quarks inside protons and neutrons zipping around each other at almost the speed of light, shooting "virtual gluons" between each other that pull them together via the nuclear strong force and keep them from flying apart. Protons and neutrons as wholes also can be thought of as zipping around each other quite fast inside the nuclei of atoms more complex than simple hydrogen. They are held together by the "residual strong force" which can be thought of as being mediated by "virtual mesons" that protons and neutrons shoot at each other, similar to how quarks can be thought of as shooting "virtual gluons" at each other and electromagnetic forces can be thought of as mediated by "virtual photons".
   
   All this talk of particles zipping around each other, and especially of shooting "virtual particles" at each other to "mediate" forces binding them together, is rather imprecise and misleading. These are all just intuitive explanations people give of quantum mechanical phenomena. Just as electrons come in wave-like "orbitals" organized into "shells" around nuclei, protons and neutrons exist in similar wave-like states organized into "nuclear shells", which each have different binding energies. Protons and neutrons don't have shells, because each of the quarks they are made of are different and so the Pauli Exclusion Principle isn't an issue, but they still exist in a wave-like state that has a certain energy. The energies of electron orbitals, and even of macroscopic movements, all have energy, which therefore affects the effective "mass" of objects, but these energies are usually completely negligible relative to the binding energies of protons and neutrons, or even the smaller binding energies of atomic nuclei as wholes, which are the energy source for nuclear reactions.

(The larger binding energies of protons and neutrons as compared to the atomic nuclei they group into are the main reason why matter-antimatter annihilation releases so much more energy than nuclear reactions. This is called "total conversion" of "matter" "into energy". Some of that energy comes from the "intrinsic mass" of elementary particles being converted into photon energy, but, if any protons or neutrons are annihilated, most of the energy comes from the binding energy of those particles being released.)

---

Extra Info About Fermions, Bosons, and Quantum Theory

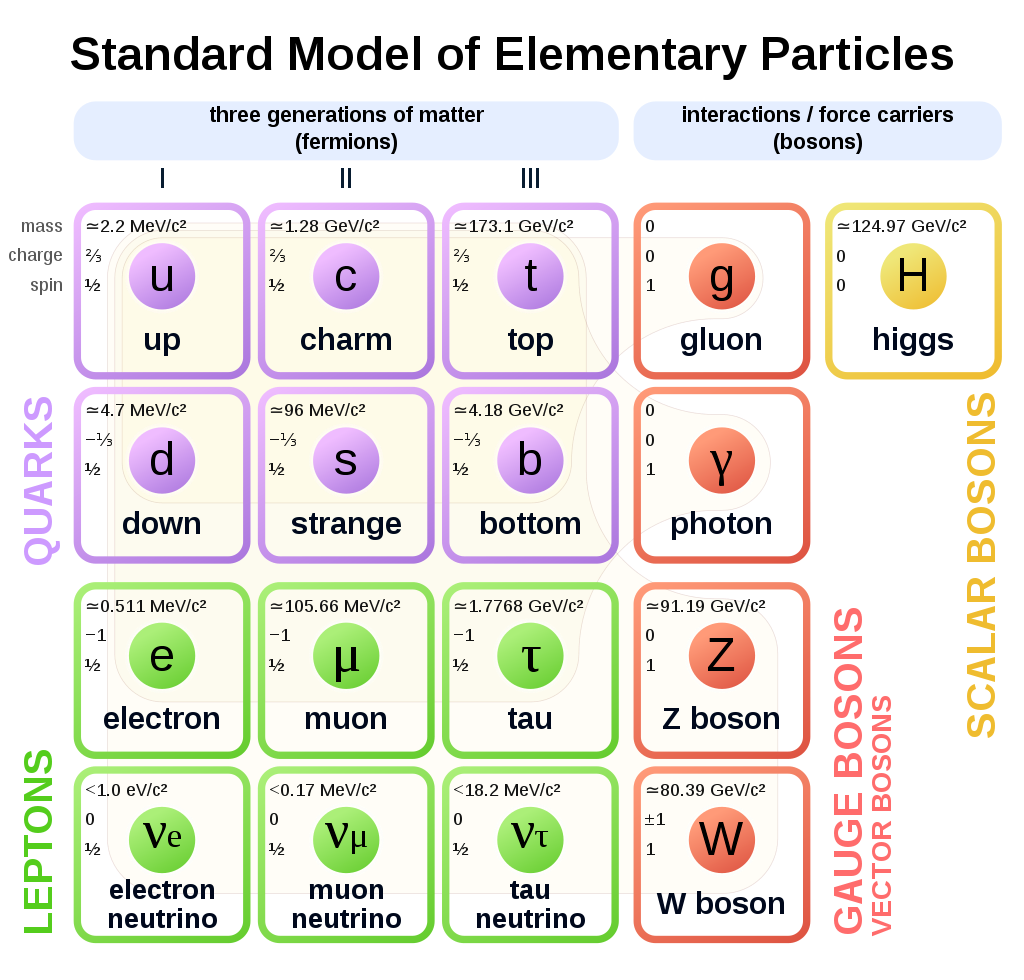
These are the elementary particles according to the standard model:

These are called "elementary particles", because they aren't known to be made of any smaller parts. You should recognize the electron; photons are what light is made of; and protons and neutrons are basically combinations of up and down quarks.

As you can see, all these particles have a quantum number called "spin" (also known as "quantum angular momentum"). Unlike macroscopic spinning objects, particles can only have spins which are multiples of 1/2 (in standard units) and they can only spin in two directions — positive and negative (often called "up" and "down", but I'll avoid that lest it cause confusion with the quarks). The sign of the spin (+ or -) is basically a matter of orientation, rather than anything fundamental to the particle.*

Any particle whose spin is an integer is a boson. Any integer whose spin is some odd number divided by two (e.g., 1/2, 3/2, 5/2, ...) is a fermion. You can put as many bosons as you want into the same quantum state/location (they just add up), but you can't do this with fermions. All the elementary fermions in the Standard Model have a spin of 1/2. Any combination of an odd number of these is a fermion, even if some are negative and some are positive. Likewise, any combination of an even number of these is a boson, even if some are positive and some are negative. (This is easy to prove: Each fermion is either adding 1/2 or subtracting it. The difference between the two states is 1, so no amount of flipping spins will change the sum to or away from being an integer.)

Examples:

Proton = 2 up quarks and 1 down quark. Two of these have positive spin and one has negative spin (or vice-versa). 1/2 + 1/2 - 1/2 = 1/2: Protons are fermions.

Neutron = 1 up quark and 2 down quarks. Spins are same as proton: 1/2 + 1/2 - 1/2 = 1/2: Neutrons are fermions.

Δ⁺ Baryon = 2 up quarks and 1 down quark, just like the proton. Unlike protons, all have spins in the same direction:. 1/2 + 1/2 + 1/2 = 3/2: Δ⁺ baryons are fermions.

π⁰ Meson (Neutral Pion) = either 1 up quark and 1 antimatter up quark or 1 down and one antimatter down quark. Spins facing opposite directions. 1/2 - 1/2 = 0: π⁰ Mesons are bosons.

ρ⁺ Meson = 1 up quark and 1 antimatter down quark. Both spins facing same direction. 1/2 + 1/2 = 1: ρ⁺ Mesons are bosons.

Alpha particle (⁴He nucleus) = 2 protons and 2 neutrons. Each has total spin 1/2. Two have + spin and two have - spin (at least in ground state). 1/2 + 1/2 - 1/2 - 1/2 = 0: Alpha particles are bosons.

Neutral Ground State Helium-4 Atom = One spin=0 nucleus and two electrons. Both electrons occupy the s1 shell and thus have opposite spins (according to the Pauli Exclusion Principle). 0 + 1/2 - 1/2 = 0: This type of atom is a boson.

Protium H₂ Molecule = 2 protons and 2 electrons, each spin=1/2. Both electrons are in the σ_1s orbital (the chemical bond) and thus have opposite spins. The spins of the two protons may either be pointing in the same direction (orthohydrogen) or in opposite directions (parahydrogen). In orthohydrogen: 1/2 + 1/2 + 1/2 - 1/2 = 1. In parahydrogen: 1/2 - 1/2 + 1/2 - 1/2 = 0. Either way, the spin is an integer, and so the particle is a boson.

In quantum field theories, these particles are actually the result of vibrations in various "fields", which have values everywhere and everywhen in spacetime. (This makes more sense if you know about Fourier series and particle wave-functions.) An electron is a manifestation of vibrations in the electron field, and the photon is a manifestation of vibrations in the electromagnetic field (which I suppose could also be called the "photon field"). One consequence of this wave-particle duality is that electromagnetic fields can more-or-less be modeled as the sum of an infinite number of "virtual photons" being exchanged between charged particles. The really accurate explanation is more complicated than that,** but this is the reason that people sometimes say that the electromagnetic force is "mediated" by photons. Similarly, the nuclear strong force is mediated by gluons and the nuclear weak force is mediated by W and Z bosons. If gravity can be explained by a quantum field theory, then it is mediated by spin-2 bosons called "gravitons". The so-called "nuclear force" or "residual strong force" that holds protons and neutrons to each other to form nuclei larger than Hydrogen-1 is "mediated" by π, ρ, and Ω mesons (mesons being bosons made of quark-antiquark pairs). (The animation linked below demonstrates the basic idea of how these particles can be transferred between the nucleons. This possibility is necessary for the quantum field theory to explain how energy is transferred between the nucleons to bind them together.)

https://en.wikipedia.org/wiki/Strong_interaction#/media/File:Nuclear_Force_anim_smaller.gif

*Actually, there is something related to spin-direction called "chirality", which is fundamental to particles, but it changes constantly (and is thus always undetermined) for charged leptons and quarks as part of their interactions with the Higgs field, which give them their "intrinsic" mass. This "chirality" (which is either "left-handed" or "right-handed") determines whether or not a particle can interact with the weak nuclear force. I'm not sure whether massless particles come in different chiralities. (I just know that their "helicity", i.e. spin-direction relative to motion, is purely dependent on their chirality). I know that only left-handed neutrinos and right-handed antineutrinos have been observed (since other kinds would only interact with gravity, i.e. be "dark matter", if they existed, which would explain why they've never been created by any process we know of) it's unclear whether or not the "intrinsic" mass of neutrinos comes from the Higgs mechanism (which I suppose would flip their chiralities back and forth). I'm very unsure about the chirality of the W and Z bosons, which I believe do derive their mass from the Higgs field but also only interact with particles of certain spins.

**Sometimes the photons turn turn into electron-positron pairs. Also, it's not so much an infinite number of particles as an infinite number of paths particles could take, which all add together to make a "probability amplitude", which is part of how things are defined in quantum physics, since the outcomes of measurements are not certain. Look up Feynman Path Integral.

Answer 4

Photons (electromagnetic fields) are usually massless, but I believe photons in a superconducting state have mass (symmetry spontaneous breaking). Mass does not mean whether a particle is "matter" or not. I believe that all particles in particle physics are "matters".