Angular collision equations help

Question

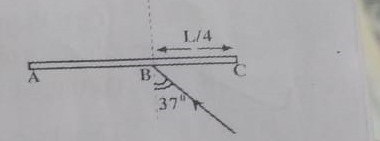
The situation is a particle of mass m striking a rod of the same mass and length $L$ at an angle with an initial velocity $v$ elastically.

Now to describe this situation I wrote the following equations:

$$mv\cos{\theta}=mv_1+mv_2$$

$$mv\cos{\theta}\cdot\frac{L}{4}=mv_1\cdot\frac{L}{4}+\frac{mL^{2}}{12}\cdot\omega$$

$$1=\frac{v_2+\omega\cdot\frac{L}{4}-v_1}{v\cos{\theta}}$$

Here I used linear momentum conservation, angular momentum conservation and coefficient of restitution respectively where $v_1$ is the final velocity of particle in the vertical direction and $v_2$ is the same for the centre of mass of the rod. And $\omega$ is the angular velocity of rod after collison.

Now my query is that since the rod is not hinged anywhere, am I right to conserve angular momentum about the centre of mass? Also I'm not sure whether the equation for coefficient of restitution should be written about the impact point or the centre. Moreover, is it true that the particle's component of velocity parallel to the rod remains unaffected?

Any help would be appreciated.

Answer 1

am I right to conserve angular momentum about the centre of mass?

In any inertial frame, linear and angular momentum will be conserved at all times. So you can pick any point fixed in an inertial frame that you want. The center of mass fits those limitations, so it is fine.

The point of collision, or the initial center of mass of the rod might also be interesting choices.

is it true that the particle's component of velocity parallel to the rod remains unaffected?

In the idealized world of physics problems where you ignore friction, things like planes and rods can only apply forces normal to their surface. Since the rod cannot apply any forces parallel to its length, the particle's momentum and velocity in that direction cannot change due to the collision. (We assume the collision is "quick" and the rod does not begin to rotate until the collision is complete).

I'm not sure whether the equation for coefficient of restitution should be written about the impact point or the centre.

The coefficient of restitution is a measure of the energy loss during a collision. You don't calculate it about a point at all. You use it to say that if the closing speed of the objects is $v$, the separation speed is $ve$. The actual location of the impact is not relevant.

Answer 2

Since the rod is not hinged you can apply conservation of angular momentum about the center of mass.

Yes the component along the rod will be unaffected since they collision occurs normal to the surface in question and since there are no forces along the rod such as friction that can affect the velocity.