2
$\begingroup$

I am reading the book by Gitman et al. 'self-adjoint extensions in quantum mechanics'.

In the book, they give a precise definition of the domain of the hamiltonian of an infinite square well.

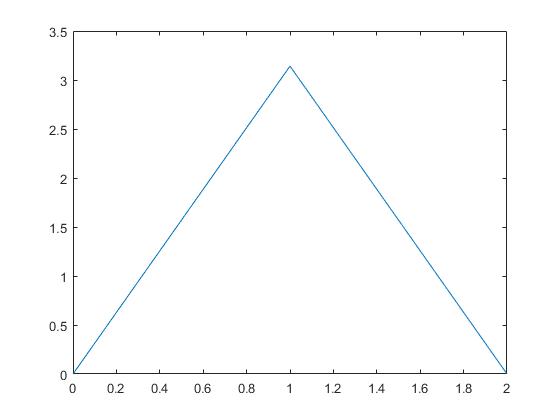
For me, the point is that, if a wave function belongs to the domain, then itself and its first derivative should be absolutely continuous. This means the tent function below is not in the domain---its first derivative is discontinuous.

This is somehow surprising to me. As I remember, we had excercises in which we were asked to expand the function in terms of the eigenstates of the well. We can then evolve the state in time. Everything seems okay.

So, what is the problem with this state? Why should we rule it out from quantum mechanics?

enter image description here

Improve this question
$\endgroup$
8
  • $\begingroup$ What's the energy of this state? $\endgroup$
    – NLambert
    Commented Apr 19, 2019 at 10:03
  • $\begingroup$ it is finite. But the second moment of the hamiltonian diverges $\endgroup$
    – Jiang-min Zhang
    Commented Apr 19, 2019 at 10:48
  • $\begingroup$ Can you quote precisely what they say in the book? Do they require this property to hold almost everywhere or sth like that? Otherwise, we are debating about your interpretation of what the book says, rather about what the book says. $\endgroup$
    – Norbert Schuch
    Commented Apr 19, 2019 at 10:55
  • 1
    $\begingroup$ As the function does not belong to the domain of the Hamiltonian operator, the energy variance is not defined in the said state. The expectation value could be defined in any cases (it is enough to decompose the state along the eigenvectors of the Hamiltonian and sum the series of the energies...to check it). $\endgroup$
    – Valter Moretti
    Commented Apr 19, 2019 at 12:49
  • 1
    $\begingroup$ However, all that has nothing to do with the time evolution of the state, which is always defined, even if the state does not beleng to the Hamiltonian domain, as it is implemented by a unitary operator. You should not rule out that state from QM! T $\endgroup$
    – Valter Moretti
    Commented Apr 19, 2019 at 12:51

1 Answer 1

Reset to default
-1
$\begingroup$

The simple answer to this problem is that the second partial derivative of one of the canonical variables appears in the Schrödinger equation when working with a continuous basis. In most problems this is the spatial coordinate as in $$ i\hbar\frac{\partial}{\partial t} \psi(x, t) = -\frac{\hbar^2}{2m}\frac{\partial^2}{\partial x^2}\psi(x,t) + \Phi(x)\psi(x,t) $$ for the case of one dimension, which is applicable to your example, but it can also be the electric field among other things.

Since quantum mechanical states are required to be normalizeable, as their squared norm is interpreted as a probability density, a discontinous first derivative leads to problems in the temporal evolution of the state. The second derivative of this function would then potentially blow up the norm of the state, which breaks the interpretation as a probability density.

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ It is completely false that discontinuities of the first spatial derivative lead to problems with time evolution. Time evolution has nothing to do with spatial regularity of the wavefunctions. Time evolution is implemented by the unitary evolutor whose domain is the whole Hilbert space. So also functions which nowhere admit derivative admit time evolution. $\endgroup$
    – Valter Moretti
    Commented Apr 19, 2019 at 12:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.