I'm currently studying Introductory Semiconductor Device Physics by Parker.
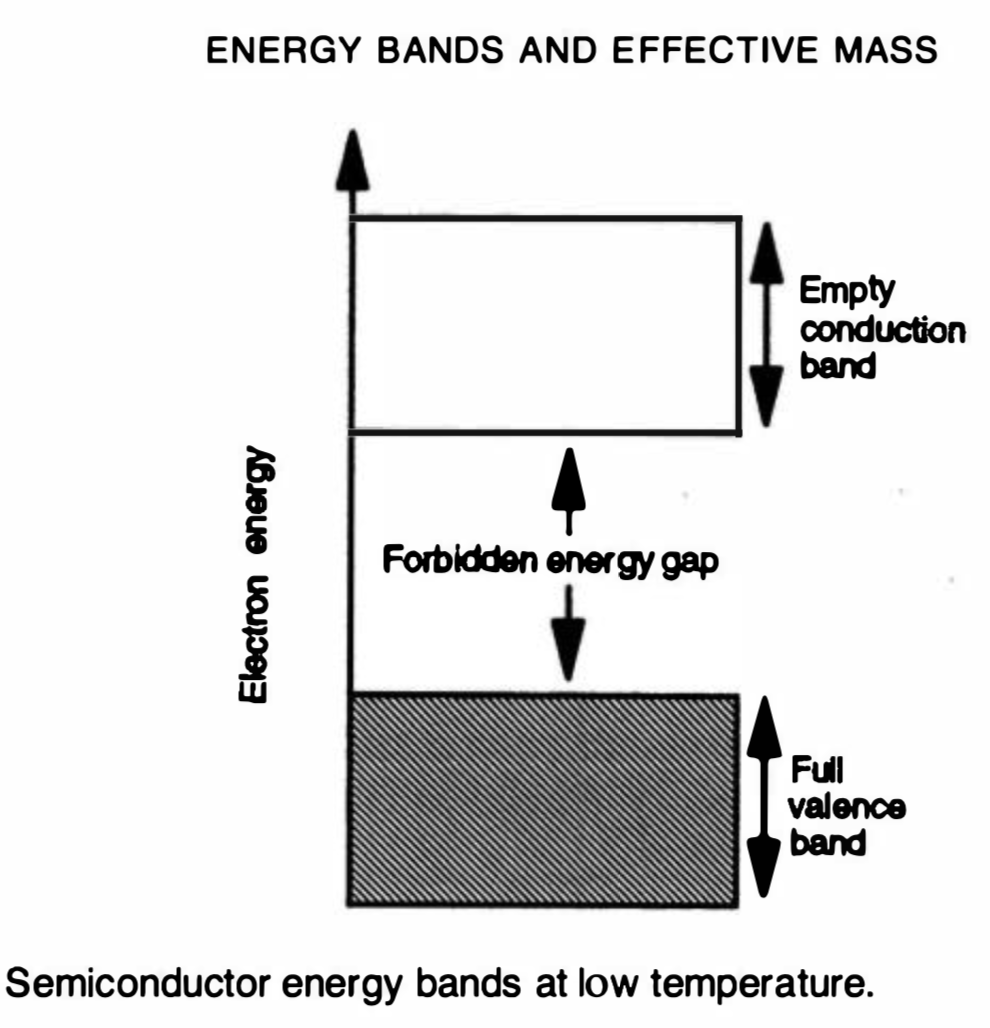
In band-theory, we know that if an electron is at the top of an energy band, then there are no allowed states immediately above it, since the forbidden band lies immediately above the top of an allowed band. See the following figure (from the same textbook) for illustration:
Given this, in the above figure, how can electrons travel from the valence band into the conduction band? It seems to me that it would have to somehow traverse the forbidden band, no?
I would greatly appreciate it if people could please take the time to clarify this.