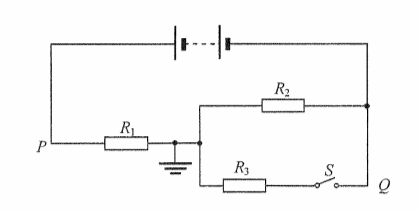
If the switch is still open, what will the electric potential at Q be, i.e. negative, positive or zero? Is there a potential difference across the grounded point and point Q, or R3, even though the branch is open? I suppose the current would be zero in that branch. Why would there be a voltage even though the branch is open?
2 Answers
The electric potential at $Q$ will be negative, why? I'll explain in a bit.
Of course there's a potential difference across $Q$ and ground point and $Q$ and $R_3$, which are different.
You're right there isn't current through the branch leading to $Q$, and there isn't "voltage" also based on $V=IR$. Voltage doesn't always means the potential difference or potential.
Electric potential is defined to be the magnitude of charge concentration in a particular region of space. Connecting a conductor to a terminal of a battery transfers the electric potential at that terminal to the tip of the conductor away from the battery terminal, thus in the study case above, the terminal of the switch (k) close to $Q$ has some fraction of the potential of the negative terminal of battery while the rest is expended and harnessed by the other branch.
Potential difference is defined as the energy expended by charges moving from a negative potential $x$ distance from positive potential region. If the switch is closed, the energy expended by the charges concentrated at $Q$ to move to the grounded point or $R_3$ is the potential difference between those points, and obviously it isn't zero.
In the lower part of your circuit you are dealing with resistors (including the connecting wires).
If a current $I$ passes through a resistor $R$ then there is a potential difference across the resistor $V=IR$.
No current passes through the lowest branch of the circuit which includes the switch because there is no conducting path in that branch.
So there is no potential difference between the earthed node and the left hand side of the switch which is therefore at zero potential.
There is no potential difference between node $Q$ and the right hand side of the switch so the potential of the right hand side of the switch will be the same as that of node $Q$.
There is a current through resistor $R_2$ going from left to right due to the battery in the circuit.
When passing through a resistor current flows from a higher potential (earth $= 0$) to a lower potential (at node $Q$).