0
$\begingroup$

I have seen this but couldn't understand so I wrote my own question.

We all have learnt in school that electric field lines never intersect. Same is the case for magnetic field lines. But I have a question:

1.What will be the resultant direction of electric field lines when a charge is placed near an infinite charged metallic sheet?

I tried to figure it out but could not. I think the field lines from the plate and those from the charge don't intersect but arrange themselves in some other way.

2. How will the resultant field lines be when a charge with uniform velocity v is introduced into a region of uniform magnetic field and the charge moves parallel to the direction of magnetic field. Won't the circular field lines produced by the charge intersect the magnetic field lines in that region?

3.What will happen when when the charge moves at an angle to the magnetic field in that region with velocity v?

I would be grateful if you could use illustrations rather than difficult mathematical expressions as I'm not accustomed to that.

P.S.- Though it seems like a homework question isn't one. Please don't down vote it on this ground.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ It's generally not a good idea to ask multiple questions within a single SE question. $\endgroup$
    – user4552
    Commented Mar 1, 2019 at 13:22
  • $\begingroup$ For question 1: Have you learned about the method of images? $\endgroup$
    – The Photon
    Commented Mar 1, 2019 at 16:49

1 Answer 1

Reset to default
1
$\begingroup$

  1. There exist a field interaction law as you know: like attract and unlike repels. The field line of a lone charged particle propagates radially outward as you must have learnt in school, but when brought into another electric field, the field line of the particle changes depending on the charge of the other field. In the case of a charged particle in front of a metal plate with the same charge, this should happen. 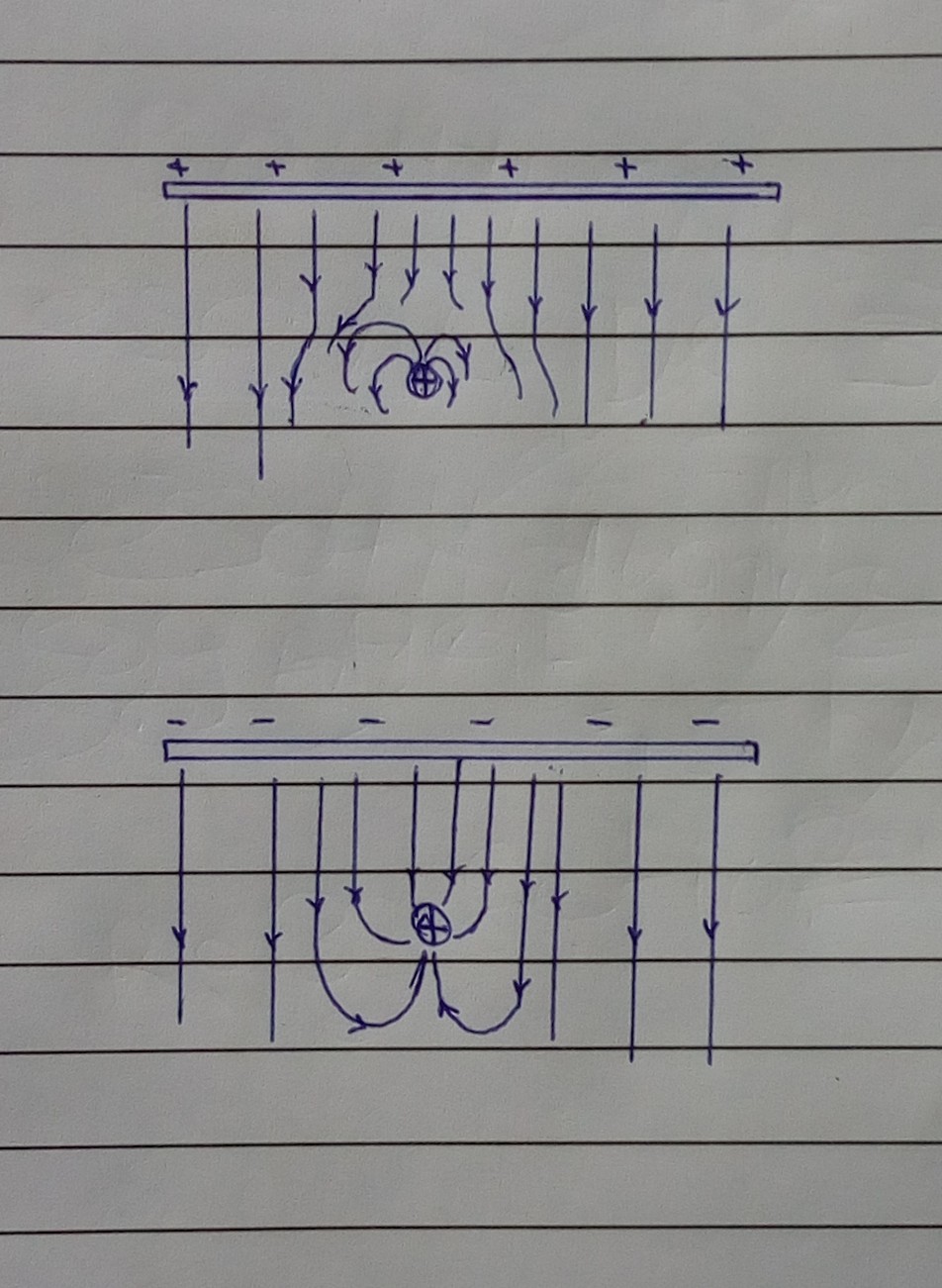enter image description here The degree of deflection between the two field line is determined by the field strength of each.

  2. First, point charges don't produce circular field line, it's radial. And the law states that zero force is exerted on a charge moving parallel to a magnetic field line.

  3. When the angle is relative to the field line, the charge experiences a force which is a fraction of the force it'll experience if it was fully perpendicular to the field. You want to know how much? $$ F=qvBcos\alpha$$

Improve this answer
$\endgroup$
2
  • $\begingroup$ aren't radial and circular the same thing? $\endgroup$
    – user8718165
    Commented Mar 1, 2019 at 14:36
  • $\begingroup$ Oh no. Radial means pointing outward in all directions, especially in the case of a sphere. Circular means moving around a center in an orbit at a distance from that center. $\endgroup$
    – TechDroid
    Commented Mar 1, 2019 at 14:42

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.