I often hear about the wave-particle duality, and how particles exhibit properties of both particles and waves. However, I wonder, is this actually a duality? At the most fundamental level, we 'know' that everything is made up out of particles, whether those are photons, electrons, or maybe even strings. That light for example, also shows wave-like properties, why does that even matter? Don't we know that everything is made up of particles? In other words, wasn't Young wrong and Newton right, instead of them both being right?
-
15$\begingroup$ "we 'know' that everything is made up out of particles, whether those are photons, electrons, or maybe even strings." Actually, we also know that those particles are properly described by a mathematical framework--path integrals--in which the wave properties are of fundamental importance. $\endgroup$– dmckee --- ex-moderator kittenCommented Dec 7, 2012 at 22:20
-
1$\begingroup$ I suggest you to read [dx.doi.org/10.1209/0295-5075/1/4/004 ] (Grangier, P., Roger, G., & Aspect, A. (1986). Experimental Evidence for a Photon Anticorrelation Effect on a Beam Splitter: A New Light on Single-Photon Interferences. Europhysics Letters (EPL), 1(4), 173–179.) and then to try to change your mind. This is clear experiment showing that light is neither a particle nor a wave field: it's both a particle and a wave. Best regards $\endgroup$– FraSchelleCommented Dec 9, 2012 at 20:42
-
3$\begingroup$ Wow, I am getting answers from both ends of the spectrum, don't know which ones are correct. $\endgroup$– user14445Commented Dec 11, 2012 at 13:28
-
1$\begingroup$ Unfortunately there are some myths and misconceptions in quantum mechanics that self-propagate in the literature. Unfortunately, you must chose. E.g., will you trust what says a poster who is known for running a personal blog and whose contributions to quantum mechanics are zero? Or will you trust what an author, who is known for developing a modern formulation of quantum mechanics, says in one of the best textbooks in quantum mechanics (a modern textbook which corrects what is said in older textbooks? $\endgroup$– juanrgaCommented Dec 11, 2012 at 15:45
-
5$\begingroup$ @user14445 I think Lubos gives a comprehensive exposition of what the duality means in terms of quantum mechanics. As I stress in my complementary answer, if you keep in mind that "wave" is a probability wave, not an amplitude wave the different terminologies stop being confusing. $\endgroup$– anna vCommented Dec 17, 2012 at 15:55
12 Answers
Duality is the relationship between two entities that are claimed to be fundamentally equally important or legitimate as features of the underlying object.
The precise definition of a "duality" depends on the context. For example, in string theory, a duality relates two seemingly inequivalent descriptions of a physical system whose physical consequences, when studied absolutely exactly, are absolutely identical.
The wave-particle duality (or dualism) isn't far from this "extreme" form of duality. It indeed says that the objects such as photons (and electromagnetic waves composed of them) and electrons exhibit both wave and particle properties and they are equally natural, possible, and important.
In fact, we may say that there are two equivalent descriptions of particles – in the position basis and the momentum basis. The former corresponds to the particle paradigm, the latter corresponds to the wave paradigm because waves with well-defined wavelengths are represented by simple objects.
It's certainly not true that Young was wrong and Newton was right. Up to the 20th century, it seemed obvious that Young was more right than Newton because light indisputably exhibits wave properties, as seen in Young's experiments and interference and diffraction phenomena in general. The same wave phenomena apply to electrons that are also behaving as waves in many contexts.
In fact, the state-of-the-art "theory of almost everything" is called quantum field theory and it's based on fields as fundamental objects while particles are just their quantized excitations. A field may have waves on it and quantum mechanics just says that for a fixed frequency $f$, the energy carried in the wave must be a multiple of $E=hf$. The integer counting the multiple is interpreted as the number of particles but the objects are more fundamentally waves.
One may also adopt a perspective or description in which particles look more elementary and the wave phenomena are just a secondary property of them.
None of these two approaches is wrong; none of them is "qualitatively more accurate" than the other. They're really equally valid and equally legitimate – and mathematically equivalent, when described correctly – which is why the word "duality" or "complementarity" is so appropriate.
-
15$\begingroup$ The wave-particle duality is an old misconception which is avoided in modern textbooks and papers. $\endgroup$– juanrgaCommented Dec 10, 2012 at 10:46
-
7$\begingroup$ Fields are believed to be fundamental only in the old-fashioned approach, which is open to several objections: among others the fields are unobservable. As Weinberg explains in his recent textbook, the old approach "is certainly a way of getting rapidly into the subject, but it seems to me that it leaves the reflective reader with too many unanswered questions". The modern picture is developed in Weinberg textbook where "here particles come first -- they are introduced in Chapter 2"... $\endgroup$– juanrgaCommented Dec 10, 2012 at 11:19
-
3$\begingroup$ Fields are not fundamental. Weinberg introduces them in Chapter 5 and only as a technical tool (as said before the fields are unobservable) valid for certain kind of interactions and dynamical regimes. Particles are much more fundamental than fields and that is the reason why particles are used in generalized theories beyond the scope of field theory. The equivalence or duality that you pretend is only in your imagination, not in nature :-) $\endgroup$– juanrgaCommented Dec 10, 2012 at 11:21
-
4$\begingroup$ @user14445 it is not only me who disagrees with his answer, but all the references that I have cited: Klein, Ballentine, Weinberg, Mandl & Shaw, CERN,... $\endgroup$– juanrgaCommented Dec 11, 2012 at 15:35
-
4$\begingroup$ @Danu Why do you attribute to me the references from other authors? As Weinberg mentions in his book what we measure at laboratory are particles. Fields are only tools. There is no problem with curved spacetimes. Only some people that pretends to obtain the concept of particle from a QFT on curved spacetime. $\endgroup$– juanrgaCommented Mar 3, 2014 at 19:52
Effectively, as the CERN website emphasizes
The theories and discoveries of thousands of physicists over the past century have resulted in a remarkable insight into the fundamental structure of matter: everything in the universe is found to be made from twelve basic building blocks called fundamental particles, governed by four fundamental forces.
It must be emphasized that they refer to quantum particles. A quantum particle is not a Newtonian particle. A quantum particle is not a wave. A quantum particle never behaves as a wave and this is the reason why the discipline that studies quantum particles such as electrons, quarks, or photons is named "particle physics" not "wave physics".
Your question about the wave-particle duality is well answered in the Klein site:
true wave-particle duality does not exist.
The site also reveals interesting historical details on how the incorrect beliefs on duality and complementarity were based in early misunderstandings of quantum theory plus some technological limitations of the apparatus used in early double-slit interference experiments.
Are "particles" really "waves"? In the early experiments, the diffraction patterns were detected holistically by means of a photographic plate, which could not detect individual particles. As a result, the notion grew that particle and wave properties were mutually incompatible, or complementary, in the sense that different measurement apparatuses would be required to observe them. That idea, however, was only an unfortunate generalization from a technological limitation. Today it is possible to detect the arrival of individual electrons, and to see the diffraction pattern emerge as a statistical pattern made up of many small spots (Tonomura et al., 1989).
Today we know that wave-particle duality does not exist and modern literature avoids the term:
The miraculous ”wave-particle duality” continues to flourish in popular texts and elementary text books. However, the rate of appearance of this term in scientific works has been decreasing in recent years (the same is true for Bohr’s notion of complementarity).
In fact, if a wave-particle duality existed or played a fundamental role it would be found in modern textbooks. A critic in the comments appeal to quantum field theory, but the fact is that you cannot find the term "wave-particle duality" in the indices of recent quantum field theory textbooks such as Weinberg (Volume I) or in classics as that by Mandl & Shaw. Why? Because, there is no "wave-particle duality" in nature.
You can also check the CERN scientific glossary and verify that there is none entry or mention to "wave-particle duality". Why? Because, there is no "wave-particle duality" in nature.
Some people believes that the wavefunctions used in some formulations of QM are real waves, but this is a mistake. A wave is a physical system which carries energy and momentum. A wavefunction is a mathematical function which cannot be observed. Wavefunctions are only an approximated way to represent the states of true quantum objects in certain formulations of QM. The quantum state of an open system cannot be represented by a wavefunction. It is not a mere question of semantics.
As the Klein site cited above clearly explains, all the quantum phenomena including interference patterns can be explained without any wave-particle duality.
One would also analyse experiments such as that of the double slit with electrons. As stated above, today it is possible to detect the arrival of individual electrons, and to see the diffraction pattern emerge as a statistical pattern made up of many small spots. To obtain the statistical interference pattern you need to repeat the experiment during a period of time and superpose the results of each one of the individual runs in a final statistical figure

The statistical interference pattern observed corresponds to a statistical distribution of positions of different particles at different time. There is no wave-behaviour for a single electron:
The manifestations of wave-like behavior are statistical in nature and always emerge from the collective outcome of many electron events. In the present experiment nothing wave-like is discernible in the arrival of single electrons at the observation plane. It is only after the arrival of perhaps tens of thousands of electrons that a pattern interpretable as wave-like interference emerges.
Notice that the author correctly write "wave-like", because no real wave is detected in the experiment, only a statistical pattern is observed in the detector.
@annaV wrote an excellent remark about our modern understanding of this experiment. I would add that recent advances in quantum theory allow us to compute the trajectory of each particle in the experiment. The result of the theoretical simulation of the particle followed by each particle in a double slit experiment is
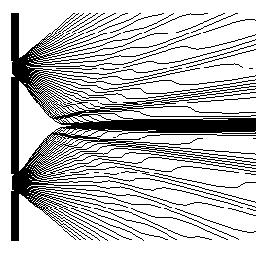
which predicts exactly the observed behaviour and the exact interference pattern in the double slit experiment.
Unfortunately, the development of quantum mechanics has been plagued with myths and misconceptions. I would recommend Ballentine textbook for a rigorous and advanced treatment of quantum mechanics without old misconceptions such as "wave-particle duality":
This approach replaces the heuristic but inconclusive arguments based upon analogy and wave–particle duality, which so frustrate the serious student.
Quantum Mechanics a Modern Development is considered one of the best textbooks today.
-
10$\begingroup$ The mathematics of path integrals and quantum field theories has wave equations embedded in it, and particle experimenters regularly measure the interference between terms in the perturbative expansion as a tool for probing physics. The wave nature remains every bit as real as the particle nature. $\endgroup$ Commented Dec 8, 2012 at 19:36
-
15$\begingroup$ I agree with dmckee, the wave nature is an indispensable part of the physics. The observed behaviour of matter can only be explained through physical concepts such as spreading, diffraction and interference, all of which are associated with waves, not particles. In fact, I would go so far as to say that the real physics is mostly that of waves! The discrete particle nature only really becomes apparent during the measurement process. $\endgroup$ Commented Dec 8, 2012 at 19:54
-
7$\begingroup$ @MarkMitchison: As stated in my answer there is no waves in QFT. I also disagree on that quantum fields are fundamental. In fact, Weinberg correctly notices in his textbook that what one measures in the lab are particles not fields. He introduces particles first, in chapter 2, and next fields in chapter 5. The fields are not fundamental and, in fact, it is possible to eliminate them completely from the theory. $\endgroup$– juanrgaCommented Dec 9, 2012 at 14:27
-
8$\begingroup$ Sorry, but you are still just arguing on semantics. The wave might be considered (by you) just a calculational tool not a physical wave, but it's still a "wave", insofar as that word has meaning (it's the only word we have!). As I have already stated, particles are associated to measurement only. If you think that physics is just about predicting the outcomes of measurements, that's fine. Personally I take the view that physics is about understanding, and for that you require fields. If Weinberg is your main reference I recommend supplementing it with Zee for quite a different view. $\endgroup$ Commented Dec 9, 2012 at 18:44
-
8$\begingroup$ @MarkMitchison The spacetime curvature is not real, but a mere "geometric analogy" as Weinberg calls it. This is why you can reformulate the theory in flat spacetime and describe the same physical phenomena without any curvature. One would differentiate a physical system from formal elements specific to a given model of it. $\endgroup$– juanrgaCommented Dec 11, 2012 at 16:11
I think you will be less confused by the answers if you keep clearly in mind that wave equations are specific differential equations which apply to many classical systems which have been studied for over two centuries in great detail as they applied to light and sound and fluids.
It so happened that the differential equations which first described the observed quantized behavior of the microcosm , like the Schroedinger equation, are also wave equations. That is why one talks of wave functions. But, and it is something that has to be emphasized time and time again, what the quantum mechanical solutions describe are not waves in the size of the "particle" in $(x,y,z,t)$ but the probability of finding a "particle" at $(x,y,z,t)$ or with a four vector $(p_x,p_y,p_z,E)\;.$
The terminology "particle" which is useful in classical physics as for example in the molecules of an ideal gas, is what creates the confusion here. We should be calling them "elementary entities" which can be described as probability waves for some manifestations, as in the two slit image in Juanrga's reply here, and sometimes as particles of classical behavior, i.e having specific coordinates and specific four vectors describing their motion, for other behaviors.
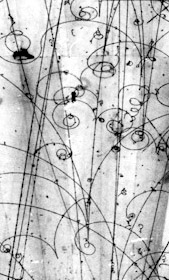
These electron positron pairs appear at specific $(x,y,z,t)$ with specific four vectors in this bubble chamber photo.
Look, the firing of sequential electrons one at a time in the double-slit experimental set-up does indeed reveal single electron detection events on the detector plate; and it is also true that after many such events a pattern emerges that is consistent with an interference pattern. Simply saying that the interference pattern results from the statistical pattern of many detection events does not explain at all why that pattern happens to be one that is consistent with wave interference! The single detection events are indeed consistent with the particle nature of the electron, but the wave interference pattern after many such single events are accumulated is consistent with the wave nature of the electron. Rather than dismiss the wave nature of the electron, what has been described actually demonstrates quite clearly the wave-particle duality that some have attempted to deny as real. The interference pattern must be explained exclusively in terms of particle physics if one wants to deny the wave nature of the electron and I have not seen that yet. On the other hand, I have not yet heard an explanation for how a "probability wave" can exhibit actual physical interference if it is only a mathematical abstraction. So the wave aspect of wave particle duality also needs to be further explained or understood.
-
$\begingroup$ I absolutely agree with your statement. Wave nature cannot be dismissed as we are really observing the interference pattern although each dot is a single electron collapse of the wave function. However, no one seems to explain why a mathematical abstract can cause physically interference. This means the waves we talk about in QM are really some form of real energy wave exchanging the energy fluctuations at a much smaller scale then quantum realm. $\endgroup$ Commented Oct 27 at 15:19
Whilst everything is made up of particles, they are not your typical "billiard ball" particles because they have a phase.
The consequence of this is that they demonstrate examples of interference when adequately set up. For example:
In the double slit experiment, particles hit the screen according to interference patterns instead of simple scatterings
In an atom, electrons are bound to specific orbitals which correspond to its resonating frequencies
and many more.
-
1$\begingroup$ Ballentine textbook, cited in my answer, devotes several sections to show how the identification of a single particle with a wave-packet or with a wavefunction gives contradictions and experimental discrepancies. $\endgroup$– juanrgaCommented Dec 10, 2012 at 10:38
-
$\begingroup$ @juanrga which sections exactly please? $\endgroup$– RevoCommented Nov 29, 2013 at 2:23
-
2
In other words, wasn't Young wrong and Newton right, instead of them both being right?
Localization defines what most physicists would think of as particles ie. yes, Newton's aether - ridding nature of its inert stage. But 20th century physics still hinges on the inert stage and cannot deny that waves are at the heart of the SM. But if we can modify the mathematics, then do we get rid of waves (like someone at CERN says)? Still NO. The duality is a deep principle for a quantum world, even if the nature of waves still needs to be sorted out in quantum information theory.
Recall that Heisenberg's uncertainty principle can be derived by taking de Broglie's rule for waves-matter (wavelengths limit resolution). This use of mass is more physical than the classical one, where it is really just a parameter. (Ironically, as you know, it was Newton (and Descartes and Galileo) who initiated the confusion of the inert stage). Now we are taught to think of light waves in a 'vacuum' a la Maxwell, but this would have Newton turning in his grave. We need to think of the background spacetime emerging from the em fields. This is the modern point of view (but no one seems to understand it yet). Then waves and particles describe two distinct properties of spacetimes - one local (events) and one nonlocal (interference etc). We assume that new theories require both types of information. This is all an oversimplification but see how Newton is only right for 20th century ideas, and not beyond. So Young is still wrong in the context of the old aether, but the continuity of ideas from classical optics to QM and QFT cannot be forgotten as we pull apart the idea of wave functions. Note also that the historical experiments were very careful to demonstrate that both waves and particles are aspects of underlying nature - and our weak understanding.
Where is de Broglie now then. The uncertainty principle in string theory uses deep mathematical dualities (STU). In principle it comes from a modified de Broglie principle (I don't know a good ref sorry). This goes far beyond the original WPD, but I think highlights the importance of WPD. An event is not just a point of classical spacetime (because this is unphysical in a theory with uncertainty) so WPD is in some sense the best idea we have for building spacetime states from both local and non local information.
Let me first show you this example, we find a lot of in the net :
![]()
Here, the detectors are the faces of the box where the cylinder is. Most of the time this example is used to illustrate the duality wave particule duality. And as we can see the detectors can detect a circle (in the yellow detector) or a square (in the blue detector). But the cylinder is not a square, not a circle, and not more the both in same time. It is just a cylinder.
So in reality there is no really wave particule duality.
What we can say is more like this: In physics we try to find the equation which rule the behaviour of things, and for that we use mathematics, and in all cases we say (and we must say if it's an approximation) all goes like if (here is the point explain below) it is this or that.
Explenation :
Consider just a simple thing we can do in mechanic. If you want to know what happend to an object if you throw it in the air. If you consider their is just its weight as force and not more, you will find that its path is a parabola. And if you make a movie of your object in the air, and you look its position image by image, you will conclude "all goes like if the path of my object is a parabola." But in reality it's wrong, there are frictions due to the air, and the earth spin round then there are some inertia force etc... Then with the time we get more and more precision in our calculus, and if you know different equations for your path and if you have a very good detector (instead of your eyes) you will have the possibility to say "oh yeah it's like all goes like this solution of this equation try to represent.
Now let's back about duality. We have some equations which explained more or less good what happend in quantum mechanic. And for some solutions of some equations we can say in the Young experiment that all goes like if the "particules" are waves, and for the photoelectric effect we can say all goes like if the "particules" are particules. But in reality we don't know what exactly they are. And like we see with the cylinder, we can conclude that it should be possible that "particules" are not particules or waves and not more the both in same time.
When Einstein try to explain the photoelectric phenomena, didn't represent photons as particules, but like a density of energy spread in a very small space drive by (here it's difficult for me to translate from french, I will ask a friend and change later if it's wrong) an electromagnetic wave which is the light. And the photon is just represent as the energy it carries.
As a conclusion, what we can say is the wave particule duality is not really real, sometime to solve a problem it will be easier to consider that the "particule" is a wave because the equations work better with, and for some other problems we will consider them as particules for the same reasons. But we don't know what exactly they are, and they are define in mathematics has probability density, and in the reality all goes like if it is the case, but in reality (without measure, because like you can see in my example of the cyclinder, the measure transform the reality as something really different, here two dimensions objects for one in three dimension) it must be something really different of that, we may never see, or never imagine because our brain is too limited.
-
$\begingroup$ How can interference pattern be explained? $\endgroup$ Commented Oct 27 at 15:25
Your perception of reality is based on your IQ developed in everyday world. Don't apply it to understand Quantum world.
All of denizens of Quantum realm are something we haven't yet understood fully. They are neither particles nor waves... they are something else. Our everyday languages don't have words to name these kind of things.
Young's double-slit experiment says that they are waves (Double-slit experiment can also be performed with atoms, electrons etc., not just light). Compton Scattering & Photoelectric Effect say that they are particles. Combining results of all valid experiments, they posses properties of both wave & particle at the same time. Common sense can deny that, but its true.
The modern version of Young's double-slit experiment:
In case you don't know, when light from same source are passed through two parallel slits, an interference pattern like barcode is formed on second screen. Its like water wave interference.
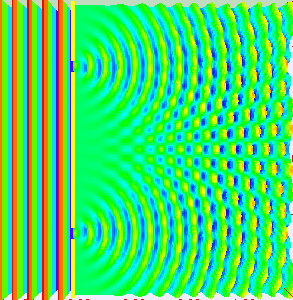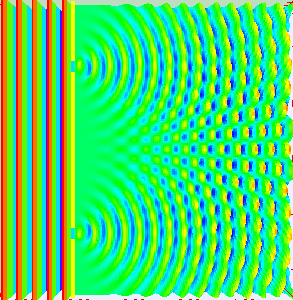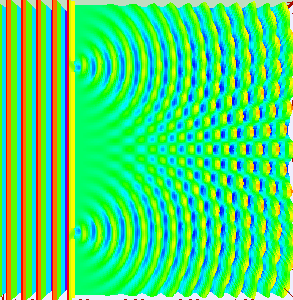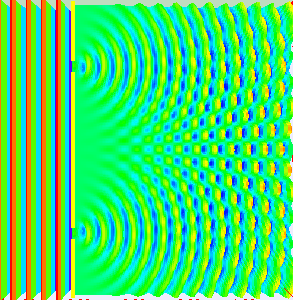

In modern version of the experiment, sensitive detectors are placed on many places of second screen to count arrival of photons. The results are interesting: Its same as that of original Young's result. White band gets very high number of photons & black band gets almost no photon. But, the problem is: Interference is a property of waves. How can it be with particle model? There's no co-ordination between photons. They are fully alone. How can a photon knows where its fellow photon would land? Well, solution to this is somewhat tricky. See below.
To visualize the concept of duality more clearly, look at the modern explanation of Young's double-slit experiment with the Schrödinger Equation:
Light reveals itself either as a stream of particles or as a wave. We don't see both sides of the coin at the same time. So, when we observe light as a stream of particles, there is no wave in existence to inform those particles about how to behave & vice versa. To solve the problem, Erwin Schrödinger proposed an idea (Physicists launched at it initially, but it became game-changer of whole physics). He imagined an abstract mathematical wave that spread through space, encountering obstacles and being reflected and transmitted, just like a water wave spreading on a pond. In places where the height of the wave was large, the probability of finding a particle was highest, and in locations where it was small, the probability was lowest.
With probability wave described by Schrödinger Equation, one can see this extraordinary property of photon: Since the photon can either be transmitted from slit 1 or slit 2, the Schrödinger equation must permit the existence of two waves, one corresponding to the photon going through slit 1 and another corresponding to the photon going through slit 2. Nothing surprising here. However, if two waves are permitted to exist, a superposition of them is also permitted to exist. For waves at sea such a combination is nothing out of the ordinary. But, here the combination corresponds to something extraordinary: The photon being transmitted from both slits simultaneously!
The same is true for any other denizen of Quantum world. It means that an atom, electron etc can exist at more than one places at once & do multiple things at once (the fundamental of upcoming quantum computer). If you see particle model this way (which is 100% correct), your common sense won't reject wave model.
-
3$\begingroup$ The image of the two slit experiment that Juanrga shows in his answer has been taken according to the wikipedia article with particle detectors at the slits en.wikipedia.org/wiki/… .quote "And in 2012, researchers finally succeeded in correctly identifying the path each particle had taken without any adverse effects at all on the interference pattern generated by the particles." This shows that the interference is pure probability, and the particle passes through one slit whole. $\endgroup$– anna vCommented Dec 9, 2012 at 21:20
-
$\begingroup$ I would add that there is novel theoretical techniques based in Bohmian mechanics that allow the computation of the trajectory of each particle in the double slit experiment. $\endgroup$– juanrgaCommented Dec 9, 2012 at 22:23
-
$\begingroup$ @anna Interesting.. Looks like I am running little outdated. I am happy to know Quantum Decoherence isn't an issue. $\endgroup$ Commented Dec 10, 2012 at 4:53
-
$\begingroup$ @juanrga I have also computed this in my paper single edge certainty $\endgroup$ Commented Aug 1, 2016 at 0:08
-
1$\begingroup$ @annav The weak measurements in that paper are fully consistent with standard QM and rely on post-selection in order to build up the map of “average paths taken.” This can only be done for an ensemble, not any individual measurement by definition. There’s still no single path an individual particle takes so long as there’s no measurement. Weak measurements included. $\endgroup$ Commented Mar 1, 2019 at 19:52
I would IMHO say that, speaking about photons (and then generalizing): Newton showed light behaved like particle, while Huygens showed light behaved like wave. Both are/were surely right, since they just showed it! What is understood by "wave behavior" or "particle behavior" might be questioned, but I assume everyone here roughly agrees on what I am referring to.
Quantum mechanics (QM) just ended the fight by unifying the picture and showing that both descriptions are pertinent to states of matter! QM shows that a particle is described by a wave-function (everyone here would agree on this), and thus by a wave. Although the wave-function is surely not the conventional classical wave, it surely manifests wave properties, like phase and interference; these are just what all we guys here agree on referring them to the "particle behavior".
On what above, I believes that everyone agrees. The thing some of us might have different opinions is statements such "when the particle behavior is detected, the wave behavior is cannot be displayed" and vice versa. This exclusion principle is somehow evanescent and needs to precisely define what we mean by particle or wave behavior. Usually, the definitions on which the experiments are based are: "particles" are detected at a defined position, while waves interfere". It has been displayed that when you detect "which-way" the particle has gone by (particle behavior), interference disappears. As soon as you erase this information, interference appears. If we limit the exclusion principle to this, I again believe that everyone here agrees upon it.
I prefer to answer it this way. The particle x or the wave x can be a particle and it can be a wave but not measured at the same time. The duality I believe refers to the mathematics. The math is consistent in both cases. The mathematics has no description for when this entity is to be a particle or a wave if they are measured at the same time. I don't believe any experiment has measured the particle nature and the wave nature at the same time. As for example in the double slit experiment you don't see the photo multiplier tube clicking a photon at the same time you see an interference pattern in the foreground do you? Duality exists but there is nothing that dictates that duality can not follow the law of excluded middle.
It would be quite wrong to insist there is no such phenomenon as wave-particle duality without first defining exactly what one means by the term. There is no doubt that particles of all sorts, including large molecules consisting of very many atoms, exhibit interference behaviours, notwithstanding the fact that fundamental particles always appear point-like when detected. A search of the internet shows that the term wave-particle duality, and associated terms such as 'matter waves', are in widespread use within recently published text books and research papers.
What would be equally wrong is to interpret the term as meaning that the wave function associated with a particle is somehow a smeared out version of the particle itself. The wave functions are complex mathematical entities that can be used to model various probabilities associated with the behaviour of the particles they are associated with.
Anybody who claims there is no wave-particle duality does not do justice to the mystery of quantum mechanics. One cannot sweep wave-particle duality under the rug. Nevertheless, the often repeated statement that an electron or a photon can be have like a wave or a particle depending on it being observed is incorrect.
Wave-particle duality can be tersely summarized as follows. Electrons and photons, quarks etc. are point particles, yet their behavior is governed by waves or wave functions. In turn, wave functions are not observable, yet their consequences are strikingly evident.

