Harmonic oscillator position expectation value

Question

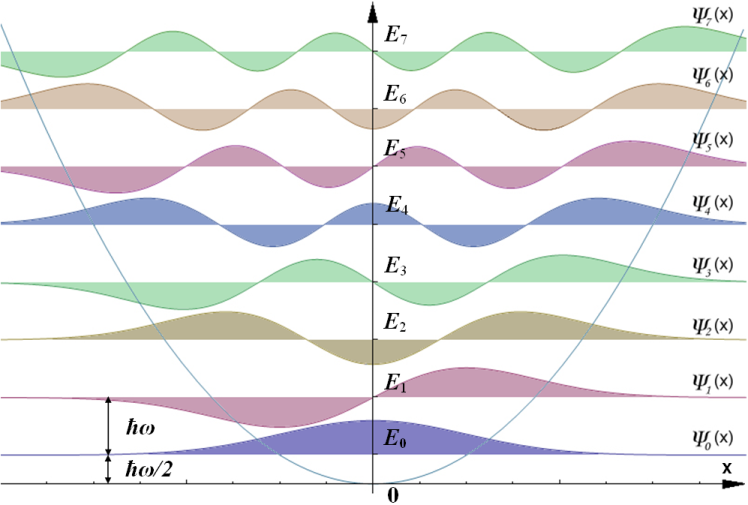
I'm trying to get the expected value as a function of time for the position, of a harmonic oscillator hamiltonian and a state vector $|\psi\rangle=a|0\rangle+b|2\rangle$.

I have $$|\psi(t)\rangle=ae^{-\frac{i\omega t}{2}}|0\rangle+be^{-\frac{5i\omega t}{2}}|2\rangle$$ and $$\langle x(t)\rangle=\langle\psi(t)|x|\psi(t)\rangle.$$

By using creation and annihilation operators, $x=\sqrt{\frac{\hbar}{2m\omega}}(a+a^{\dagger})$ where $a^{\dagger}$ is the creation operator and $a$ the annihilation operator.

From here, it's easy to see that $\langle x(t)\rangle$ because $a|0\rangle=0$, $a^{\dagger}|0\rangle=|1\rangle \propto a|2\rangle$ and $a^{\dagger}|2\rangle \propto |3\rangle$ and all the dot products with the bra $\langle\psi|$ will be zero.

But how can this make sense? if the expected value of the position is 0 for all time t... wouldn't the oscillator be standing still? I was expecting to get a sine or cosine function

Answer 1

Congratulations! You found out that the time dependence of the harmonic oscillator's eigenstates do not resemble the classical oscillator. If you want a non-zero expectation value you should prepare the system in a superposition of adjacent eigenstates, like $$ |\psi\rangle = c_0 |0\rangle + c_1|1\rangle. $$ That's a consequence of $x$ depending on $a + a^\dagger$.

Either way, if you want the state that truely resembles the classical oscillator you should look at the coherent states. There are many ways to define them, one example that makes clear their resemblance to the classical oscillator is to translate by a finite distance $d$ the ground state: $$ |\psi\rangle = \exp \left (-\frac{i p d}{\hbar} \right )|0\rangle. $$ Using the Heisenberg picture, where the time-dependent operator $x$ is $$ x(t) = x(0) \cos \omega t+\frac{p(0)}{m\omega} \sin \omega t $$ and $|\psi\rangle$ is fixed on time, you can prove that the expectation value of $x(t)$ evolves just like a classical oscillator of amplitude $d$: $$ \langle x(t)\rangle = \langle \psi |x(t)|\psi\rangle = d \cos \omega t. $$

Answer 2

The expectation value is zero because there is a symmetry between $x$ and $-x$. If you look at the form of the eigenfunctions below, you'll see that both $\psi_0$ and $\psi_2$ are symmetric about the $y$-axis. Intuitively, this means that if you take the expectation value of either of them, or their sum (their sum will have non-trivial time evolution, but you can convince yourself that the symmetry will be conserved - there's no reason for it to prefer one side over the other), the expectation value of $x$ will be zero.

In general, eigenstates of the harmonic oscillator do not tend to have the oscillatory behavior that one might expect from classical mechanics. However, this feature is present for coherent states.

Answer 3

First recall that the $\psi_n(x)$ are time independent solutions so there is no reason to suspect that $\langle x\rangle$ ought to behave like a classical oscillator since clearly $\langle n\vert x\vert n\rangle=0$. Now it could happen that your state is not an energy eigenstate so that the probability density $\vert \Psi(x,t)\vert^2$ is time-dependent, but that doesn't imply that $\langle x\rangle$ would be time-dependent as well: imagine an ice cream scoop symmetrically melting: the mass distribution might change in time but the average position of the ice cream might remain constant.

As others have indicated, coherent states, which are specific linear combinations $\psi_n(x)$ containing all $n$ values, have average $\langle x\rangle$ that goes like a cosine: see an answer to this question for details.

In your specific case $\langle x\rangle=0$ by symmetry. Since $\psi_n(x)$ is an even function for all even $n$'s and an odd function for all odd $n$'s, you basically have \begin{align} \langle x\rangle &= \int dx \left(a^*\psi_0(x)+b^*e^{2i\omega t}\psi_2(x)\right) x \left(a \psi_0(x)+b e^{-2i\omega t}\psi_2(x)\right)\, ,\\ &= aa^*\int dx \psi_0(x)^2 x + (a^* be^{-2i\omega t}+ab^*e^{2i\omega t}) \int dx \psi_0(x)\psi_2(x)\\ & \qquad +bb^* \int dx \psi_2(x)^2 x \tag{1} \end{align} In (1), every function under an integral is odd, since the products $\psi_0(x)^2, \psi_2(x)^2$ and $\psi_0(x)\psi_2(x)$ are even but multiplied by $x$.

One has to be a bit careful about the limits here, since they are $\pm\infty$, but the exponential factor $e^{-\lambda x^2/2}$ that enters in $$ \psi_n(x)=H_n(\sqrt{\lambda}x)e^{-\lambda x^2/2} $$ will make the integrals converge and you are left with an odd function integrated between symmetric limits, which yields $0$ by parity.