Resolve The Envelope Detector Circuit

Question

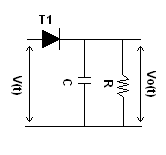
I was working on a little project and I was wondering if the envelope detector circuit could be resolved analytically. Let me explain.

The circuit is the one shown above, I know the values of $C$, $R$ and $V(t)$ which is the input signal. My $V(t)$ is a smooth function and I know all of its derivatives. The thing I would like to find is the function of time $V_d(t)$ which is the voltage drop across the diode assuming the Shockley equation to be true at every instant in time.

$$ I(t) = I_s \Big(\exp \Big(\frac{V_d(t)}{V_t}\Big) - 1 \Big)$$

Another thing that I assume is that Kirchhoff Voltage law is true for every $t$. I've found a pretty nasty differential, first order, equation, which is:

$$ \exp\Big(\frac{V_d(t)}{V_t}\Big) = \lg \Big[\frac{1}{I_s} \Big( \frac{V - V_t}{R} + C\frac{d}{dt} (V - V_d) \Big) + 1\Big] $$

Can this circuit be solved? Does the result has any limitation other than the ones mentioned above?

EDIT: I've realized that the question is meaning less without knowing the $V_t$, in this case it is: $$V_t(t) = A(1 + m \cos (2 \pi f_1 t + \phi_1))cos(2\pi f_2 t+ \phi_2)$$

Answer 1

The differential equation can be written in the form :

$\frac{d{{V}_{s}}}{dt}=-\frac{{{V}_{s}}(t)}{RC}+\frac{Is}{C}\left( {{e}^{(V(t)-Vs(t))/{{V}_{d}}}}-1 \right)$

I can be wrong but I do not think she admits an exact analytical solution.

But this differential equation can be solved numerically very easily (with Python for example)