What is the theory behind imaging a precision pinhole?

Question

In my efforts to characterise a 0.67 NA microscope objective (working distance of about 15mm, effective focal length of 25mm), I have placed a 20 micron precision pinhole at the focal plane of the objective, and back illuminated the pinhole with a 767nm laser. The light coming out of the objective is focused onto the imaging plane (camera plane) with a F=1 metre lens.

By my understanding, the image field $U_i$ should be a convolution of the Point Spread Function (PSF) $h$ and the object field $U_o$, i.e.

$$ U_i(x_i,y_i) \propto \int_{-\infty}^\infty\int_{-\infty}^\infty h(x_i-\xi, y_i-\eta) U_o(\xi,\eta)\, d\xi d\eta\, , $$

where the proportional sign is for good measure as there are some coefficients in front, but that does not affect the profile. The image intensity profile is given by

$$ I_i(x_i, y_i) = |U_i(x_i, y_i)|^2\,. $$

In my case the microscope objective has a circular aperture, and that means that the PSF $h$ is given by the airy function, i.e.,

$$ h(x,y) = \frac{J_1 \left( \frac{2\pi}{\lambda} NA \sqrt{x^2 + y^2}\right)}{\sqrt{x^2 + y^2}} \, , $$

where $J_1$ is the Bessel function of the first kind, order one.

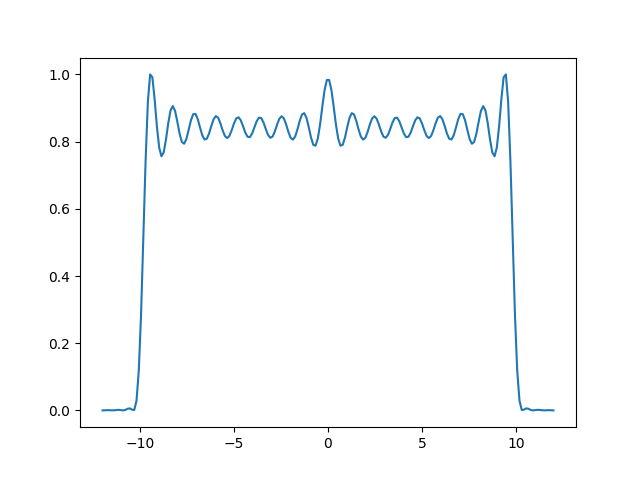
I have plotted out the intensity profile (gauss quad integral) that I expect for the current pinhole and wavelength, taking into account the magnification of the system, and I got the following plot.

However, this differs from my measurements taken in experiment:

The size of the image is approximately 20 microns (pixel size = 3.75/40 microns). The number of peaks somewhat represent what is shown from the plot above. What concerns me more is how the intensity profiles goes to zero between peaks, unlike what the theory predicted.

Is my understanding of the current theory incorrect?

Answer 1

Do note that there is a difference between coherent and incoherent imaging (see Introduction to Fourier Optics by J. Goodman, Chapter 6).

In coherent imaging, the imaging system is linear in amplitude, and the image amplitude follows your first formula. In incoherent imaging, the imaging is linear in intensity, and the image intensity looks like a small modificiation of your first formula:

$$ I_i(u,v) \propto \iint_{-\infty}^{\infty} |h(u-\xi,v-\eta)|^2 I_o (\xi,\eta) d\xi d\eta $$

This is certainly different from what you would get by using the coherent imaging formula and squaring the amplitude to get the intensity. Also, in your expression for the Airy pattern, the argument of the Bessel function and the denominator should be the same (see Wikipedia article on "Airy disk").

Regarding NA, the illumination making through the pinhole has to fill the entire aperture of the objective - otherwise you are working with an effectively lower NA.

---

Since your main goal is to characterize your high NA objective, I would like to offer some advice based on personal experience...

When characterizing the imaging system, I wouldn't dismiss the issue of coherence (as first raised by @fiddlehead) easily. Both temporal and spatial coherence can show up as dirty interference patterns that will ruin your image. I had this experience when trying to measure the modulation transfer function of a high NA objective (NA = 0.8) using USAF target.

Even for temporally incoherent source (e.g. LED), spatial coherence can still matter. It helps to place a diffuser between the sample and the illumination to scramble the light modes. In my experience, it's hard to get rid of false fringes without diffusers.

Lastly, I would advise that using a 20 um pinhole for characterizing your NA 0.67 objective is not helpful at all. At this NA, you will be sensitive to

1. spherical aberration from any window thickness between your sample and your objective

2. coma from tilt. You probably want less than 0.1 degree.

and the pinhole exercise tells you nothing about these two aberrations.

I strongly recommend getting a point light source and measuring the PSF directly, and with imaging condition as close to the realistic condition (e.g. if you have a vacuum window in your future setup, please buy a flat window with same thickness and use it in your imaging setup).

Also see this paper for using SNOM fiber tip as a point light source. You can ask vendors like K-Tek Nanotechnology to buy one or two fibers instead of buying a whole bundle for \$$$$.

• "High numerical aperture (NA=0.92) objective lens for imaging and addressing of cold atoms" Opt. Lett. 42. 1043-1046 (2017) (preprint)

Answer 2

This looks like an interference pattern caused by the laser's coherence when passing through the pinhole (see e.g. here). Can you try illuminating the pinhole with an incoherent source, instead, such as an LED? I believe that should produce a flatter profile similar to the one in your model.