$ e^+e^- \longrightarrow \mu^+\mu^-$ probability density function of $\theta$ strange trend

Question

I'm considering the process: $$ e^+e^- \longrightarrow \mu^+\mu^-$$ The cross section in the center-of-mass frame: \begin{equation} \left( \frac{d \sigma}{d \Omega} \right)_{CoM}= \frac{\alpha^2}{4 s} \sqrt{1-\frac{4m^2_{\mu}}{s}} \left( 1+\frac{4m^2_{\mu}}{s} + \left( 1-\frac{4m^2_{\mu}}{s} \right)\cos^2\theta\right) \end{equation} Let's fix the parameter $\sqrt{s}=0.212$ GeV, I take $m_e=0$ for semplicity, but I' am not in the case of the ultra-high-energy limit so $m_{\mu} \neq 0$.

I want the probability density function of the observable $\theta$. $$ \Pr [\theta_1 \le \theta \le \theta_2] = \int_{\theta_{1}}^{\theta_{2}} f(x) \, dx $$ $$f(x)= \frac{C}{\sigma_{total}}\left( \frac{d \sigma}{d x} \right)_{CoM} \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,C \Rightarrow \int_{0}^{\pi}f(x)=1$$ $$f(x)=\frac{2s}{\pi(4m_{\mu}^2+3s)}\left( 1+ \frac{4m^2_{\mu}}{s}+\left( 1-\frac{4m^2_{\mu}}{s} \right)\cos^2x \right)$$ Below are two graphs of the PDF by changing the range on the y axis.

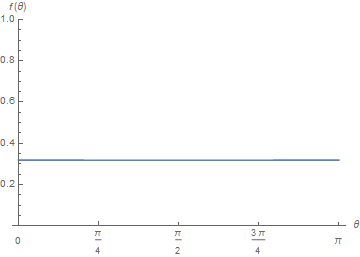
I'm not at all sure that the result makes sense.

In fact it would imply that there are no privileged directions. The variability of the cross section is practically independent of the angle and therefore is at the end constant.

I do not think it could be that way but if it is not so I do not know where my mistake is.

Answer 1

The integral should be done over $d\Omega$.

Here is the differential cross section for the process.

$$ \frac{d\sigma}{d\Omega} =\frac{\alpha^2}{16E^2} \left(1+\cos^2\theta+\frac{m^2+M^2}{E^2}\sin^2\theta+\frac{m^2M^2}{E^4}\cos^2\theta\right) $$

For $m=0$ we have

$$ \frac{d\sigma}{d\Omega} =\frac{\alpha^2}{16E^2} \left(1+\cos^2\theta+\frac{M^2}{E^2}\sin^2\theta\right) $$

Let

$$ I(\xi)=2\pi\int_0^\xi\frac{d\sigma}{d\Omega}\,\sin\theta\,d\theta, \quad0\le\xi\le\pi $$

The cumulative distribution function is

$$ F(\theta)=\frac{I(\theta)}{I(\pi)}, \quad0\le\theta\le\pi $$

Hence

$$ P(\theta_1\le\theta\le\theta_2)=F(\theta_2)-F(\theta_1) $$

The probability density is

$$ f(\theta)=\frac{dF(\theta)}{d\theta} =\frac{2\pi}{I(\pi)} \left(\frac{d\sigma}{d\Omega}\right) \sin\theta,\quad0\le\theta\le\pi $$

For $E=M$ this works out to

$$ f(\theta)=\frac{1}{2}\sin\theta $$

Here is a graph of $f(\theta)$.