Growth of black holes and the no-hair theorem

Question

Let's assume there is a black hole, and there are two starships (A and B), hovering above the black hole at the same distance from the event horizon, but on the opposite sides of the black hole. According to the "no-hair" theorem, both starships should measure exactly the same gravitational force from the black hole. The situation is depicted in the picture.

Now let's throw a very massive object C from the direction where the starship A is positioned to the black hole. As the object C is falling, the starship A should measure a greater gravitational force, because it is closer to the massive object C. Now the situtation looks like in this picture:

According to the theory of general relativity, an outside observer should never see an object cross the event horizon - instead the object will freeze outside the event horizon. I know that the object will become more and more redshifted, and finally it will fade to black. But it should still exert a gravitational force. If it is so, the spaceship A will continue to detect a greater gravitational force, even after it stops receiving any light from the object C.

If this is right, how can a black hole ever grow and how can it consume anything? And if it somehow consumes the falling object C, how can the "no-hair" theorem be true, as you can still measure presence of C? And if my reasoning is not correct, where have I made a mistake?

I know there are similar questions to mine, but as far as I could find none answers exactly my question.

Answer 1

The mistake seems to be that intuition developed in the context of one approximate model is being extrapolated to a situation that breaks that approximation.

The "approximate model" that I'm talking about is the model in which the spacetime metric is fixed, as it would be for an eternal black hole in an otherwise empty universe, and none of the other objects being considered have any effect on the metric. This can be a useful approximation with those other objects have negligible mass compared to the mass of the black hole. The statement "the object will freeze outside the event horizon" would be appropriate (asymptotically) in this approximation.

However, the question describes a situation in which the mass of object C is not negligible. To determine what the spacetime metric would be in this situation, in principle, we would need to solve the Einstein field equation in a dynamic scenario with two masses, the one labelled BH and the one labelled C. This equation is non-linear; there is no generally useful sense in which two single-mass solutions can be "added" to obtain a two-mass solution. (I wrote "added" in scare quotes because I don't even know what that would mean here.) So the picture of object C approaching the original (say, spherical) event horizon of BH arbitrarily closely is not correct, because the event horizon will change while that is happening.

Solving the Einstein field equation in such a two-mass scenario is very difficult, with numerical calculations on powerful computers being the leading approach today. However, for the sake of illustrating the dynamic nature of the event horizon, a crude back-of-the-envelope exercise might be sufficient.

Consider an eternal Schwarzschild black hole with mass $M$, let $r$ denote the usual radial coordinate, and let $R=2GM$ denote the Schwarzschild radius (in units with $c=1$). The innermost circular orbit is at $r=3R/2$, and something orbiting at that radius must be moving at the speed of light. Anything that is initially moving in a tangential direction inside that radius will necessarily spiral into the black hole. Now, here's the crude back-of-the-envelope exercise. Suppose that objects BH and C both have mass $M$, so that they would both have event horizons at $R=2GM$ if they existed in isolation — but suppose that they are both present, with "coordinate distance" $< R$ between their would-be event horizons. (For the sake of this crude exercise, we can pretend that these statements are well-defined.) Then the midpoint between them has "coordinate distance" $<R/2$ from both of the two would-be individual event horizons, which means that a flash of light emitted from that point would not be able to escape along any direction at all, because it is closer than the innermost circular orbit with respect to both of the individual "black holes", even though it is not inside the naive event horizon of either one of the individual "black holes." The conclusion of this crude exercise is that the real event horizon is not a pair of spherical surfaces centered on the two masses, because the real event horizon also encloses the midpoint between the two masses before their naive event horizons touch each other.

Again, this was a very crude back-of-the-envelope exercise, but it's sufficient for showing that an event horizon is generally not a static thing: it changes shape during the process. This is confirmed by numerical calculations and is illustrated in figures 1 and 15 from "Revisiting Event Horizon Finders," https://arxiv.org/abs/0809.2628, which are reproduced here for convenience:

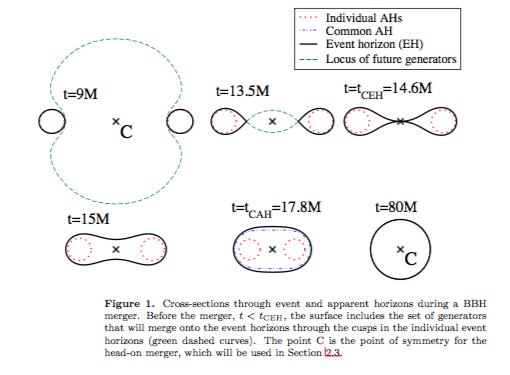
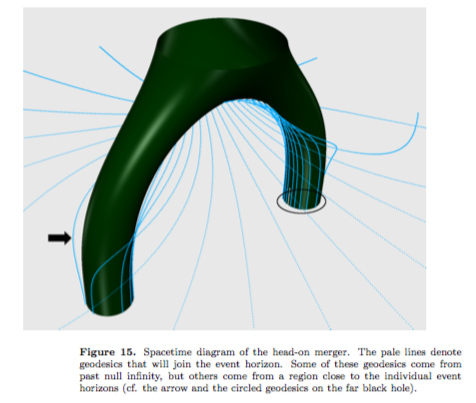
After two black holes merge, the system quickly settles into a nominal configuration with a traditionally-shaped event horizon, producing gravitational waves in the process. The No-Hair "Theorem" only says that the final result should be a nominal black hole with a traditionally-shaped event horizon.

The original question isn't about a merger of two black holes; it's about what happens when an object C with significant mass (not necessarily a black hole itself) falls into a black hole BH. But the reasoning and the conclusion is qualitatively similar: when object C is close enough to the original BH, that little bit of extra gravity will extend the region from which light cannot escape, which defines the event horizon. The event horizon will change shape during the process, finally settling back down to a nominal shape, emitting gravitational radiation in the process. Working this out explicitly is exceedingly difficult, but this reasoning is enough to resolve the paradoxes posed in the OP. The No-Hair "Theorem" applies only to the final state, not to the process; and the picture of the infalling object freezing outside the original event horizon is not correct, because the event horizon will change dynamically, becoming momentarily non-spherical, for precisely the reason stated in the OP: the infalling object adds to the overall gravitational effect, which affects not only the observer at A, but also any light that is being emitted from the object.