Orientation of the linearly polarized light comming from circularly polarized light that went through a quarter waveplate

Question

If circularly polarized (CP) light enters a quarter waveplate (QWP), it is converted to linearly polarized (LP) light. But what is the orientation of the resulting LP light? Does it depend on the phase of the entering CP light or its handedness?

This question arises from a practical work. Fluorescence emitted by chiral molecules can be circularly polarized to a certain degree. The goal is to measure the amount of each polarization in the emitted light by rotating one of each polarizers.

Let's say that a "mixture" of CP light is emitted from a chiral molecule (monochromatic). About 25% of the light emitted is L-CP and the other 75% is R-CP. After passing through the quarter waveplate, the light should be ellipticaly polarized (EP). But what is the orientation of this polarization (ellipse tilted at which angle)? How can I predict at which angle a LP filter will block most of the light?

I hope that I was clear in my explanations. Maybe some of my assumptions are wrong!

Answer 1

In both cases the light is linearly polarized 45° off of the fast axis of the QWP. The handedness decides if it will rotate + or - 45° from the fast axis. If you are looking the direction the light is going, it will rotate the opposite direction as the handedness. Jones calculus can show this, which can be added if needed.

Answer 2

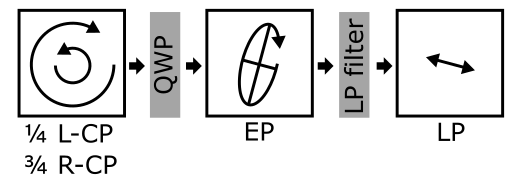
If you send circular polarized light into a QWP the output is always linear, and the axis of the linearly polarized light is determined by the orientation of the waveplate and the handedness of the light. It does not depend on the overall phase of the input. In particular it will be polarized on one of the diagonals that split the two axes (fast & slow) of the waveplate, and which diagonal depends on what handedness light you put in.

To see this a bit more mathematically, think about how you describe circularly polarized light mathematically and what a QWP does. If I decompose my light along the two axes of the waveplate, then it is $\vec{E}=E_0(\sin(\omega t),\sin(\omega t \pm \pi/2),0)$, where the $\pm$ is one sign for each handedness. The QWP will add some uninteresting net phase to these to oscillating components and a difference of $\pi/2$ (if it's a zeroth order QWP, else $\pi/2+2n\pi$) to one of the axes (depending on which axis is fast). If you consider both handedness cases on the input, you get:

$$ \vec{E}_{LH}=E_0(\sin(\omega t),\sin(\omega t - \pi/2),0)\rightarrow QWP\rightarrow E_0(\sin(\omega t),\sin(\omega t-\pi/2+\pi/2),0)=E_0(\sin(\omega t),\sin(\omega t),0) $$ $$ \vec{E}_{RH}=E_0(\sin(\omega t),\sin(\omega t + \pi/2),0)\rightarrow QWP\rightarrow E_0(\sin(\omega t),\sin(\omega t + \pi),0)=E_0(\sin(\omega t),-\sin(\omega t),0) $$ And as you can see both inputs are linear but rotated 90 degrees from each other.

All of this is linear optics, so in general you can treat each polarization independently, figuring out how they would each individually be transformed through each waveplate, and then add them back up at the end. Also, note that the light before the QWP in your example is also elliptically polarized. For exact calculations, and in general for more complicated systems, using Jones matrices is a good approach, as suggested by Alfred Centauri in the comments, although I'm not sure such a heavy-handed approach is necessary, you can probably just do it by hand like I just did.

For a measurement, taking data points with the polarizer will tell you the relative magnitude of the ellipticity but not the handedness of it, but you should be able to figure out the handedness e.g. by measuring with and without the QWP you suggested at an appropriate angle and seeing the direction in which the ellipticity deflects.

Answer 3

If the input is a mixture (in the quantum-mechanical sense) of left and right circularly polarized light, then the output is a mixture of light polarized linearly along two perpendicular axes (which is not the same as elliptically polarized light). If you pass it through a linear polarizer with the correct alignment, you'll get as much light out as there was left-circularly (or, depending on the orientation, right-circularly) polarized light in the first place. What you've created by combining the quarter-wave plate and linear polarizer with correct relative alignment is simply a circular polarizer, and it sounds like that's what you really want.

The easiest way to understand polarizations is as points on a sphere (which is known as the Bloch sphere or Poincaré sphere). If you take the north and south poles to be left and right circular polarization, then the equatorial points are the linear polarizations (antipodal equatorial points are perpendicular linear polarizations) and all other points on (the surface of) the sphere are elliptical polarizations. Any reversible/non-dissipative process can only rigidly rotate this sphere. A quarter-wave plate is reversible (you can undo the effect with another one) and it converts circular to linear polarization; that means it rotates the Bloch sphere by 90° around some axis through the equator. Which axis (and therefore which two equatorial points it rotates the poles to) depends on the QWP, not on the incoming light. (You can rotate the axis by rotating the QWP.) You can use this model to visualize what the QWP will do to any incoming polarization, once you know what it does to the circular polarizations.