No-S-$B^+$ Theorem like Results

Question

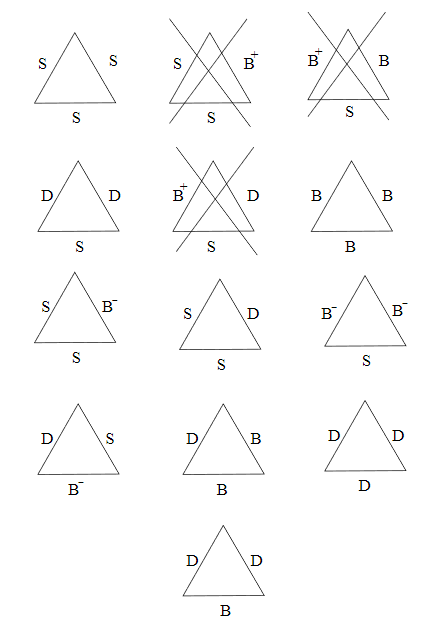
Classification of multipartite quantum state is an interesting topic in quantum information and there have been many accomplishments in the field. For example, according to the result of Thapliyal, for tripartite pure state case, there can't be a tripartite pure state with a pair of subsystems in separable marginal state and another pair in PPT (Positive Partial Trace) bound entangle state. This result can be summed up as the following figure. S stands for 'Separable', $B^\pm$ is for 'Bound entangled PPT(NPT)' and D is for 'Distillable'.

$B^+$ Theorem.">

However, is there any progress in ruling out other categories in the catalog given above? What do we know more about tripartite pure states in 2018?

Answer 1

Hayashi and Chen did exhaustive classification of tripartite pure states. Let the following letters denote S : separable, P : PPT-Entangled, R :non-PPT reduction and N : Non-reduction states for each marginal bipartite state. Then, there can be essentially 8 cases: SSS, SSN, SNN, PNN, RRR, RRN, RNN, NNN. These are all physically possible quantum states, hence the classification is complete.