Sjruru,
The wheel is indeed a great analogy for this.
Imagine a single wheel with centre O that rolls along the ground. Take two opposite points on the wheel (on the top and on the bottom) and call them T and P respectively.
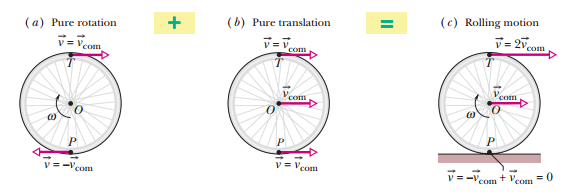
Have a look at diagram (a), where the wheel is rotating purely about the origin O. At any given moment, at point P will have velocity -v and T will have velocity +v (with velocity tangent to the circumference of the wheel).
In (b), when the wheel is purely moving forwards (translating) at velocity +v, it follows that all points of the wheel have velocity +v too.
Therefore, as (c) is a wheel both rotating and moving forwards at the same velocity (v), it is a combination of the processes occurring in both (a) and (b). It is where at any given moment, P (in contact with the ground) will have an instantaneous velocity of zero (since it is both rotating at -v and translating at +v, which cancels out to 0). Therefore
 = 0.
= 0.
Point O travels forward at velocity +v, since it is not rotating, but purely translating.
Finally, point T, however, is not only rotating forward at velocity +v, but it is also translating forwards at +v too. v + v = 2v, so at any given instant T has a velocity of +2v. Therefore
 = 2.
= 2.
Now, replace the wheel with the Earth, and a point (Q) underneath the floor is where the Sun lies. Both the wheel situation and the Earth's orbit is similar because both the wheel and the Earth are rotating and translating at the same time. Also, we know the Earth rotates clockwise if the viewer stands below the Earth's orbit, watching it translate towards the right.
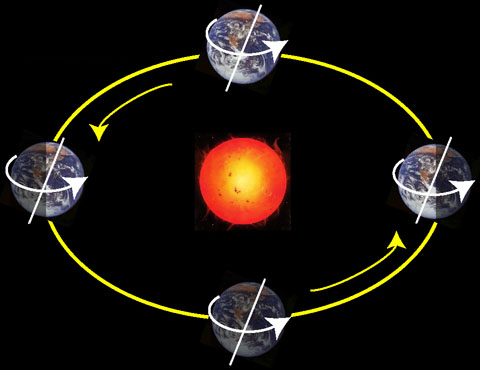
Therefore, in the same way, the outer side of the Earth (facing away from the Sun) would travel faster than the inner side (facing towards the Sun.)
Without knowing the velocity of the pure translation of the Earth, according to Yakov Perelman in your extract, the magnitude of the velocity of the rotation of the Earth (at point P and T) is around v = 0.5km/s. Since the pure translation only adds to the velocities of P and T equally (say translation velocity = t km/s)
Therefore,
Total velocity of P (inner) = t - v km/s
Total velocity of T (outer) = t + v km/s
The difference between the two sides of the Earth (T - P) = 2*v km/s = 2*0.5 km/s = 1 km/s.
And of course, the inner side (P) would be day time, and the outer side (T) would be the outer side.
It is quite something to realise that at night time we are actually flying across the solar system at twice the speed as our daytime counterparts.
Great question, and hope this helps!
Yours, Eugene.



