1
$\begingroup$

I have a bachelor's in physics & its recently struck me that I do not understand, semantically, what phenomenon allows us to measure the speed of light through air in a small room with a laser and a spinning mirror. I understand "the how," which is to say I know that measuring the angle reflected by a beam (fired at a fixed distance) against a mirror (rotating at high angular velocity) allows us to measure the speed of that beam. What i do not understand is why this experimental method is possible, and therefore satisfactory to claim measurement of a finite speed.

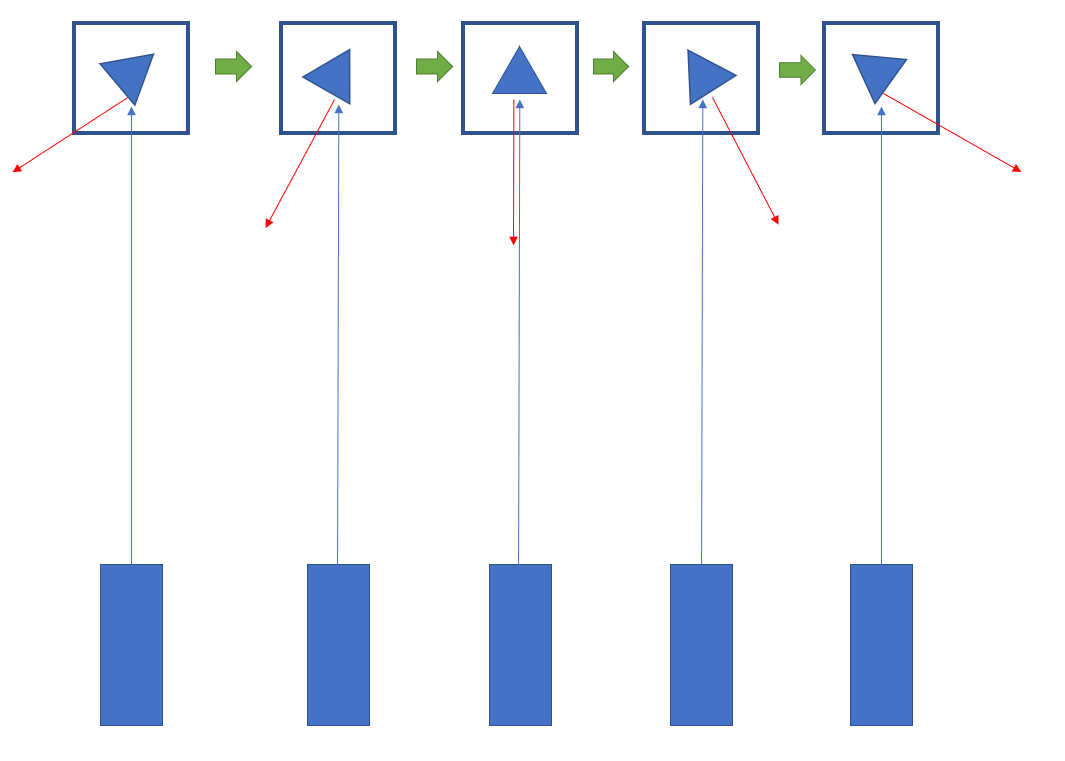
For a typical Fizeau–Foucault method setup: If the beam is continuous & mirror perfectly flat, shouldn't light always get reflected at whatever angle is governed by normal geometry?...as a result, I would imagine a wedge of light having fixed-width, & depending only on the spatial coordinates of the mirror, not on t.

To help try and make my question clearer, I made a diagram. The red arrows are how I would expect light to be reflected off of a spinning flat mirror: enter image description here

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Wikipedia has a good explanation of the experimental setup. $\endgroup$
    – niels nielsen
    Commented Dec 7, 2018 at 8:45

2 Answers 2

Reset to default
1
$\begingroup$

The rotating mirror is hit twice, on the way to and returning from another distant mirror, but at different angles, such that the return light forms an image slightly offset from the source. When the rotating mirror is static, the outgoing and return angle are the same and the image coincides with the source

Improve this answer
$\endgroup$
4
  • $\begingroup$ > "When the rotating mirror is static, the outgoing and return angle are the same" <== how is this angle-ratio related to the speed of the beam though? $\endgroup$
    – Rob Truxal
    Commented Dec 13, 2018 at 5:00
  • 1
    $\begingroup$ @RobTruxal the beam has to cover a long distance between the 2 bounces, the time it takes is proportional to the angle change of the mirror in between $\endgroup$
    – Manu de Hanoi
    Commented Dec 13, 2018 at 5:28
  • 1
    $\begingroup$ ...ok wow actually that makes total sense now; you'd just need to know the angular velocity of the mirror very-very precisely, and it would need to not change very much. $\endgroup$
    – Rob Truxal
    Commented Dec 13, 2018 at 5:38
  • $\begingroup$ ...or you'd need a very long round trip $\endgroup$
    – Manu de Hanoi
    Commented Dec 13, 2018 at 5:47
1
$\begingroup$

Think of the beam from the rotating mirror like a beacon from a lighthouse. This beam is sent to a distant mirror. As it sweeps across the distant mirror only a small portion of the beam will be reflect directly back to the rotating mirror. My daughter and I actually did this experiment in our garage. I ended up adding additional mirrors which caused the returning beam to be even more precise. What I mean by precise is the return beam had a very narrow passage back to the rotating mirror. What I have described in this answer is how a rotating mirror and a distant mirror can create a movement of light that is measurable. I am not sure if it matches the details of fizeau’s experience, but I believe it addresses your question.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Anyone trying this should remember that the actual movement of the mirror is 1/2 the reflected angle. If you rotate a mirror say 5 degrees the reflected beam will move 10 degrees. $\endgroup$
    – Lambda
    Commented Jan 31, 2021 at 16:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.