Spacing of resonant modes in a (laser) cavity

Question

I don't see why the frequency spacing between resonant cavity modes should decrease with higher frequency. Here's what I have:

For a laser cavity of length $L$, from the condition of constructive interference during a roundtrip

$$e^{-i 2\omega \frac{L}{c}}=e^{-i 2 \pi q}$$

we get the possible resonance frequencies inside the cavity as

$$\omega=q \cdot 2\pi \cdot \frac{c}{2L}$$

with an integer $q$

The resonance frequencies (axial modes) are thus equidistantly spaced in the frequency domain

$$\Delta \omega=2\pi \cdot \frac{c}{2L}$$

However, in Siegman's Lasers on Page 499, this frequency distribution is illustrated and described as

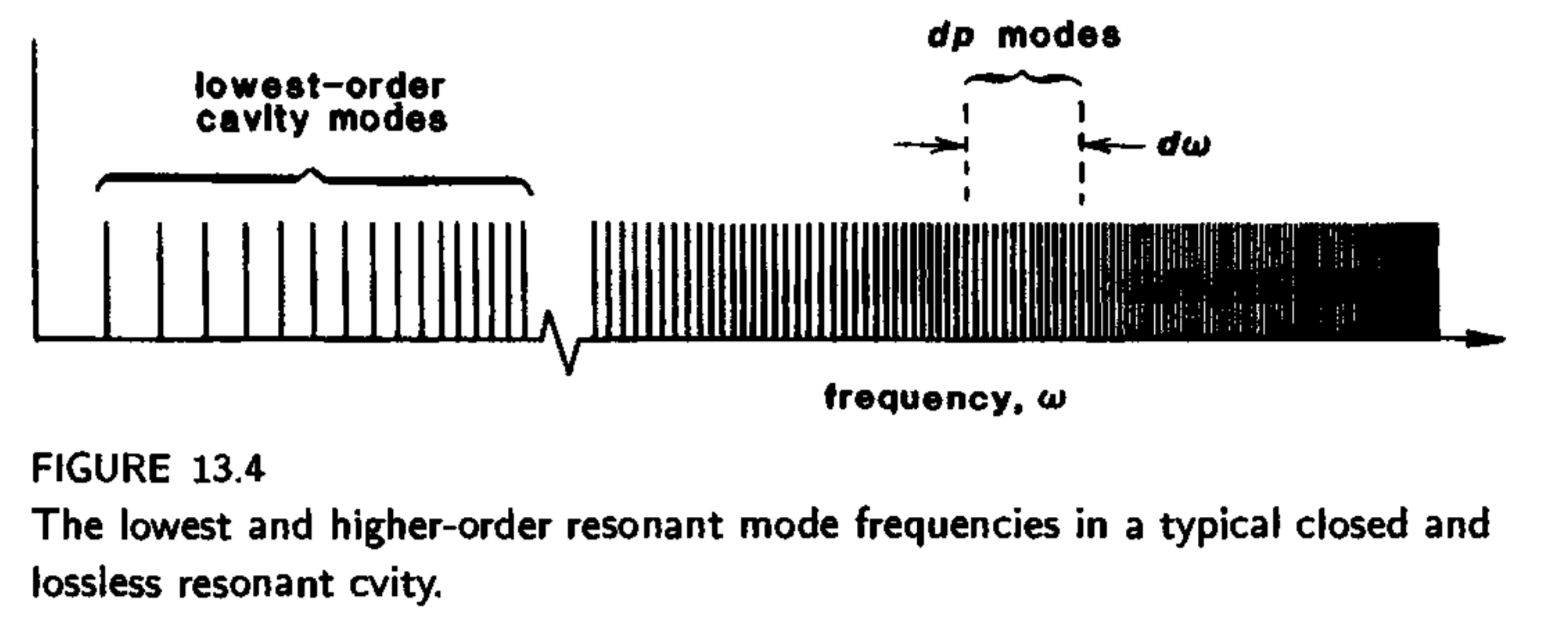
Frequency Distribution of Resonant Cavity Modes

Suppose we consider some arbitrary shaped (...) cavity having closed and completely reflecting walls. Let us then calculate all the theoretically possible lowest and higher-order electromagnetic modes in this cavity; and plot the resonant frequencies (...) as tic marks in the frequency scale.

As we go to higher frequencies, where the cavity dimensions become large compared to the resonance wavelengths, these resonant modes become more and more closely spaced along the frequency axis.

No formula is given for this decrease in spacing. I could not find any derivation in the chapters that preceded this illustration and argument. What am I missing?

Answer 1

I don't believe the section referenced is referring to the modes of a linear (1D) optical resonator but, rather, the modes of a 'volume' resonator analogous to the acoustic resonances in my home theater (sigh).

In such an acoustic 3D resonator, the modes are distinguished by three numbers, $n_x,\,n_y,\,n_z$ indicating the order of the mode in each of the three dimensions and the frequency of the mode is proportional to

$$f_{n_xn_yn_z} \propto \sqrt{\left(\frac{n_x}{L_x}\right)^2 + \left(\frac{n_y}{L_y}\right)^2 + \left(\frac{n_z}{L_z}\right)^2}$$

and so the frequency spacing does decrease with increasing order.

Answer 2

It’s because the cavity resonances occur when the length is an integer multiple of wavelength. Since $\omega=2\pi c/\lambda$, then you see the spacing decrease as 1/$\lambda$ in frequency.