0
$\begingroup$

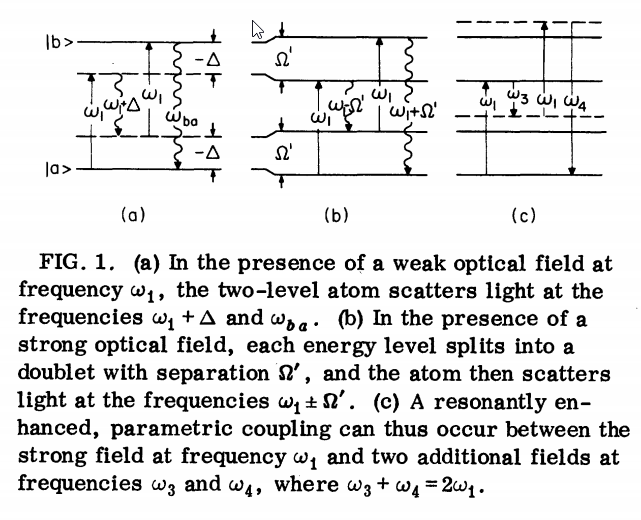
In this paper it says that for a two-level system excited by two fields:

$$ V_{ab} = -\mu_{ba} E_1 e^{i \omega_1 t}+ E_3 e^{-i \omega_3 t}$$

"In steady state the off-diagonal density-matrix element $\rho_{ba}$, exhibits harmonic oscillations at an infinite number of frequencies of the form $n\omega_1 \pm m \omega_2$, where n and m are integers." If the strong field $E_1$ is treated correctly to all orders while the weak probe field $E_3$ is treated to only first order, then $\rho_{ba}$ oscillates at three dominant frequencies: $\omega_1, \omega_3,$ and $2\omega_1-\omega_3$

enter image description here

Any ideas how I can show explicitly how this system can be expressed as an infinite number of frequencies? This paper cites a previous paper, where it works out $\rho_{ba}(\omega_1), \rho_{ba}(\omega_3),$ and $\rho_{ba}(2\omega_1 - \omega_3)$ by fourier transforming (which I discuss in this question). Within this previous paper it's not clear to me where it uses this assumption that $E_3\ll E_1$ to obtain these three special frequencies.

Additionally, I'm hoping to understand what happens in the case when $E_1$ and $E_2$ are both strong.

Improve this question
$\endgroup$
3
  • $\begingroup$ Isn't the Fourier transform, by definition, a way of representing a time series signal as the sum of an infinite number of frequencies? Or am I missing something specific in your first part of your question? $\endgroup$
    – honeste_vivere
    Commented Sep 12, 2018 at 14:49
  • $\begingroup$ The form of $n \omega_1 \pm m \omega_2 $ is not trivial. I'm interested in finding the fourier amplitudes associated with these frequencies, and how, to first order in the probe, these amplitudes can be approximated to be these three dominant frequencies. $\endgroup$
    – Steven Sagona
    Commented Sep 12, 2018 at 17:25
  • $\begingroup$ The emergence of $n\omega_1 \pm m\omega_2$ can be understood as a limit of multi-order time-dependent perturbation theory (see Chapter 3 of "Nonlinear Optics") by Boyd. $\endgroup$
    – wcc
    Commented Sep 17, 2018 at 3:09

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.