What if looked inside a moving spaceship, from Earth?

Question

So we all know about time dilation, and how Alice travelling in a spaceship relative relative to Bob ages more slowly than him.

When Alice gets back to Earth, she has biologically aged time $t$, while Bob has biologically aged $t + \delta t$.
But this is fine since Alice has just made a quantitative gain lifespan, while she will still qualitatively live the same life as Bob. I.e., she was able to perform the same tasks that Bob did in time $t$.

So, what if Bob had a telescope and looked into Alice's spaceship (which has windows)? Would he see Alice going on with her slow-mo life?
And what would Alice see if she looked down at Bob?

Answer 1

From the time they separate to when they meet, Alice has had less time than Bob. She has had less time to do stuff, like ageing, writing a diary or watch movies.

As to what would twins see if they looked at each other, the answer depends on the specifics of the trajectory that Alice takes. Let’s assume that Alice only accelerates to turn around once she is far enough and she wants to come back.

Each twin carries with them a laser with an internal closck that is set to flash once every 24 hours. We can use these lasers to think about what each twin sees the other twin doing. This makes sense: activities performed by each twin will go in rythm with their clock, and hence with the laser.

Look at the diagram below.

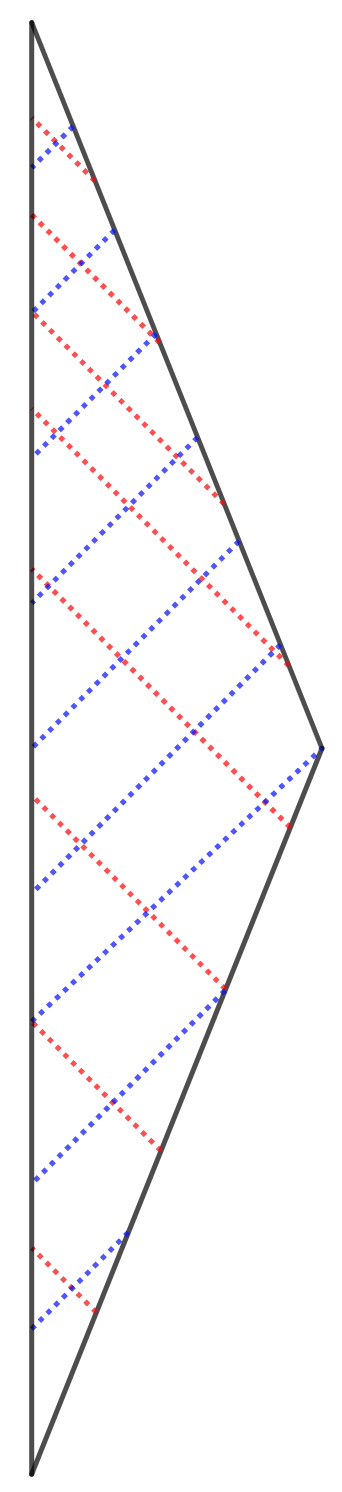
This diagram is drawn in the reference frame of Bob. The vertical black segment represents 10 days of his life. The croocked black line is Alice's worldline, who is also inertial for most of the experiment, except where the line is kinked, which is the instant in which she turns on her thrusters to reverse directions.

The blue dotted lines indicate the propagation of the Bob's laser's flashes. You can see 9 of them, because the laser's flash on day 0 and 10 coincide with the start and end of the experiment.

The red dotted lines indicate the the propagation of Alice's laser's flashes. I did the maths to make sure that each flash is emitted after 24h as measured from Alice's clock. You can see 8 of them. The ones on day 0 coincides and 9 coincide with the start and end of the experiment.

If you focus on Alices's worldline, you can understand what she sees Bob doing. During her first leg, her laser flashes 5 times, while she receives only 4 of Bob's laser's flashes, including the one at the start of the experiment. Indeed, her first leg lasts 4.5 days for her, and during this time she only sees Bob doing 3 days worth of stuff. If she would be able to watch him, she would indeed see him going in slow-mo. During her second leg, which also lasts 4.5 days from her perspective, she receives 7 of Bob's flashes, the last one being emitted at the moment she reaches him. Thus, if she were to observe him during this time, she would see him do 7 full days of activity. She would see him moving faster than usual.

What does Bob see? You can stare at the picture to convince yourself of the following. He receives in total 8 flashes from Alice, 5 from her first leg (including the one on day 0), and 5 from her second leg (including the one on the last day). But he receives the first 5 over the course of 6 of his days, and the second 5 in just under 4 days. Thus he sees Alice living 4 days in slow-mo, and 5 days accellerated.

Qualitatively, the same thing is happening for both of them: they first see their twin moving too slow, and then too fast. Quantitatively, the situation is slightly asymetrical, because in the end of the day, between their two meetings, Alice has lived 9 days while Bob has lived 10.

Answer 2

From the time they separate to when they meet, Alice has had less time than Bob. She has had less time to do stuff, like ageing, writing a diary or watch movies.

As to what would twins see if they looked at each other, the answer depends on the specifics of the trajectory that Alice takes.

Let’s assume that Alice only accelerates to turn around once she is far enough and she wants to come back. While she is coasting at constant velocity in interstellar space, Bob would see her moving in slo mo.

(To see this, consider Alice having a computer program that emits a laser flash towards Bob every second. Bon can observe that. You know that every second on the spaceship is more than a second long as seen from Bob’s point of view, by time dilation. So he receives a flash every 1.2 seconda for example. But say that computer is also playing music, and Alice is dancing to that music. If she is keeping rhythm, she is synchronised with the laser flash, so if Bob could see her, he would actually see her moving in slow mo!)

But by special relativity, Bob is moving at constant speed in Alice’s frame, so she would see him moving in slo mo.

If that sounds paradoxical, and that is why this is called the Twin Paradox. The reason its not contradictory, and why it’s not actually a paradox, is that when Alice turns on her thrusters to accelerate herself to come back to Earth, she will slow down even more from Bob’s point of view. And she would see Bob speed up.