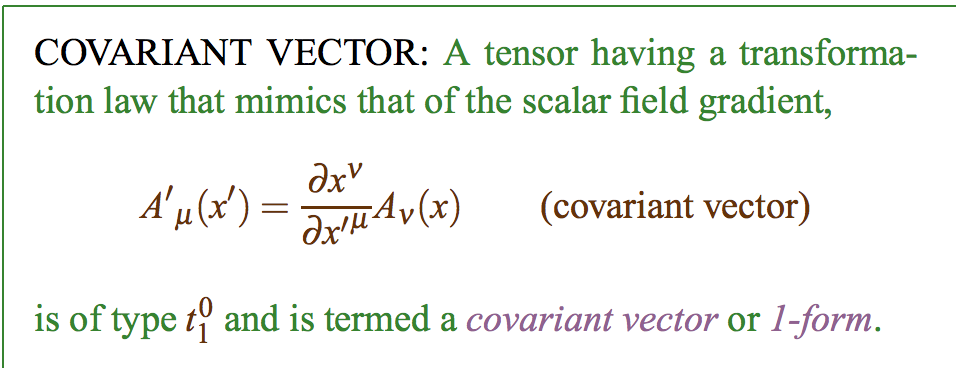
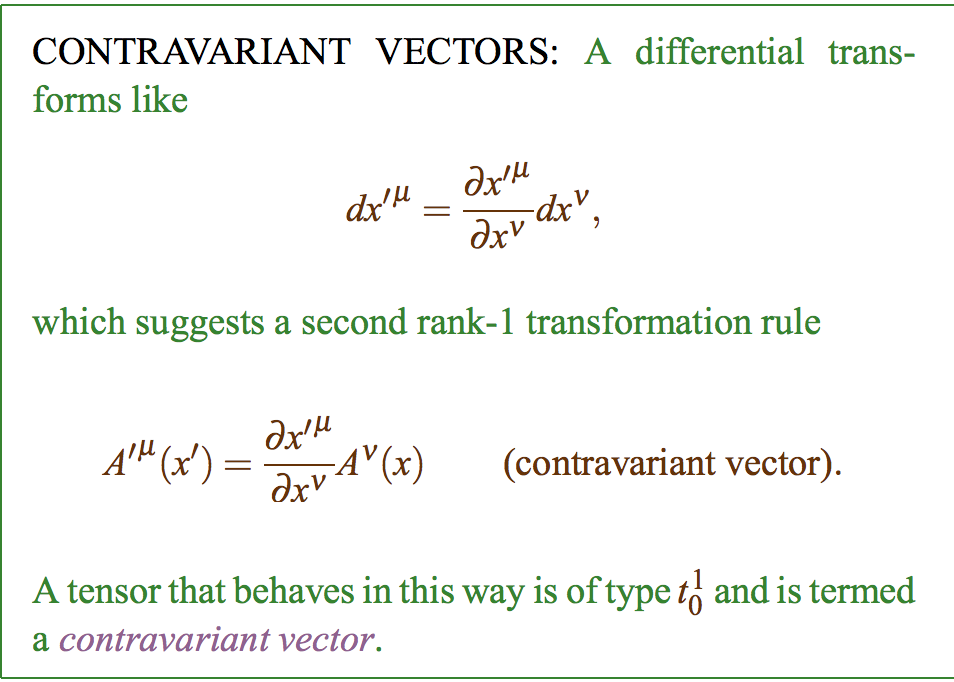
sorry is there any good intuition behind the following definitions. I am having trouble understanding these. Or is it recommended to just continue reading and accept these definitions for now?
Update: I think I roughly understand now, I took a look at covariant and contravariant transformations first. But any help is still appreciated :)
If you change to coordinates to where the tick marks (units) are spaced twice as wide:
Contravariant vector, such as displacement, will then be measured to be half as many tick marks. This is opposite (contra) to the unit vectors which doubled in length.
Covariant vector, such as a gradient, will then seem to be twice as steep per tick mark. This is the same (co) as the unit vectors, which doubled in length.
This is myself answering this question 2 years later. I still don't have a complete understanding of this, but when studying manifolds and tangent vectors this is much clearer. The coordinate changes correspond to different local homeomorphisms to R^n. And the coordinate changes then sure that the vector (or covector) on the manifold is consistent throughout different local coordinates. Read Nakahara, Geometry Topology and Physics's chapter on Manifolds for a clearer understanding
