1
$\begingroup$

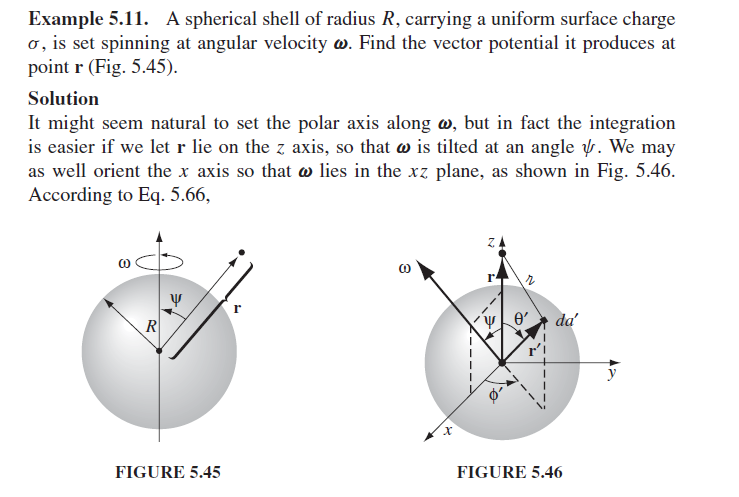
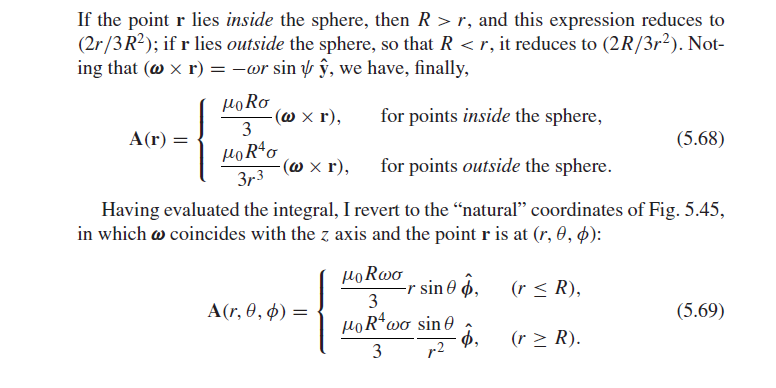
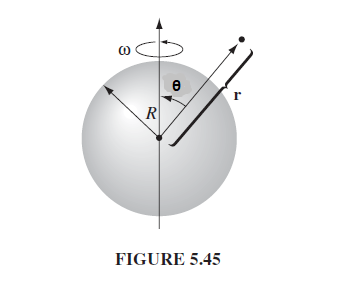
I have done all the calculation in finding the vector potential leading up to the equation 5.68. But then Prof. Griffiths goes back to the original figure and mentions that the coordinate of the point $\mathbf{r}$ is $(r, \theta,\phi)$. And now I have a problem here. Because, we all know, that in spherical coordinate $\theta$ is the angle from the $\mathbf{z}$ axis and it remains constant even when the point rotates around the $\mathbf{z}$ axis. In the original figure $\theta$ actually would be $\psi$. But he doesn't use $\psi$ in the ultimate equation 5.69 rather goes on with $\theta$ which I am unable to get. Or is he just generalizing the result where the angle is $\theta$ instead of $\psi$?

What am I missing here in my reasoning?

Below are the relevant figures and equations.

enter image description here enter image description here

EDIT: I think the first figure (5.45) should have been the following to match up with his explanations towards the end.

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ The images are not visible to me. $\endgroup$
    – Farcher
    Commented Aug 8, 2018 at 8:39
  • $\begingroup$ Really @Farcher? It seems okay to me. Anyway here are the links to the image. i.sstatic.net/e4rym.png & i.sstatic.net/l3jhW.png. $\endgroup$
    – Global
    Commented Aug 8, 2018 at 8:44
  • $\begingroup$ Thank you. It seems to be a function of the application used to view your page. On the iPad it works in Firefox and Safari but not when using the Stack Exchange app. $\endgroup$
    – Farcher
    Commented Aug 8, 2018 at 8:51

2 Answers 2

Reset to default
1
$\begingroup$

Griffiths explains it well: he defines $\psi$ as the angle between the radius vector of the point of interest $\mathbf r$ and the angular velocity vector $\omega$ solely for the purpose of the calculation in the special tilted coordinate system. The vector potential value expressed in that system depends on this angle.

The change from $\psi$ to $\theta$ is made because he wants to express the result in the original frame, where the coordinates are usually denoted $r,\theta,\phi$; he could use symbol $\psi$ instead of $\theta$, but that would be confusing for the users of the formula, because the angle from the polar axis is usually denoted $\theta$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ To your second paragraph: Yes, the coordinates are usually denoted by $(r, \theta, \phi)$ but he has taken it as $\psi$ in the original coordinate system too. Things would have been confusion-less had he taken that as $\theta$ in the figure 5.45. $\endgroup$
    – Global
    Commented Aug 8, 2018 at 10:42
  • 1
    $\begingroup$ Ah yes, I suppose you're right. It is a minor point though, it does not seem very confusing to me. $\endgroup$
    – Ján Lalinský
    Commented Aug 8, 2018 at 10:55
  • $\begingroup$ You can suggest an improvement to the author, perhaps he will improve it in the next edition. Check the errata first, though. See reed.edu/physics/faculty/griffiths.html $\endgroup$
    – Ján Lalinský
    Commented Aug 8, 2018 at 11:03
0
$\begingroup$

The $\theta$ is probably a typo due to the standard notation to call that angle $\theta$, but you are right that from the picture it should be $\psi$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.