It is well known that when water flows through a tube you can make it flow faster by making the tube narrow.
Now consider what happens when a group of people are moving and the space becomes narrower. The opposite of what happens with water happens here. People start to move slower.
I was wondering if there is any fluid that shows this kind of behaviour and what would cause that.
An incompressible (i.e. constant density, like water under most cirumstances) fluid has to satisfy the continuity equation $\nabla V = 0$, where $V$ is the velocity of the fluid.
This means that because the same amount of mass per unit of time goes in at one end as goes out the other end and the volume per unit of mass stays constant, the velocity of the fluid has to increase as the cross-sectional area of the tube decreases along the flow direction.
A compressible fluid on the other hand can change in density and therefore does not obey the same rules. If you take for example a supersonic gas flow like in a rocket nozzle or a jet fighter exhaust, the fluid will counterintuitively flow slower as the cross-sectional area decreases, and faster as the cross-sectional area of the flow increases.
(Table from Introduction to compressible flow by Eric Pardyjak, University of Utah)
A classic example is a laval nozzle, where the flow behind the critical cross-section (the narrowest part in the middle) is supersonic and will go faster (note the increasing V in the diagram) as the nozzle gets wider.
(image taken from https://commons.wikimedia.org/wiki/File:Nozzle_de_Laval_diagram.png, public domain)
Now consider what happens when a group of people are moving and the space becomes narrower. The opposite of what happens with water happens here. People start to move slower.
Do they? Consider a large room full of people which must exit through an unobstructed hallway. The people inside the room will be moving slowly as they wait to enter the hallway. Once inside the hallway, their movement will be unobstructed. Velocity is highest in the narrowest space.
I think your confusion may stem from an inconsistent notion of "fast". One sense of fast is flow rate: filling a bucket or emptying a room as quickly as possible. Another is flow velocity, which would be relevant trying to squirt water a maximal distance.
Usually the two are at odds, for example with a sprinkler where you want to squirt water far but also squirt a lot of it, there's an optimal orifice size that gets the flow velocity high enough for good range without introducing too much friction. The optimal size will depend on the water pressure available and the friction in the distribution system leading up to the sprinkler: the pipes, valves, etc.
The main limit to what you are looking for is mass flow. Assuming steady state flow, mass in equals mass out. Thus, if you decrease the cross sectional area, you must increase the mass flow per unit area. Typically that means increasing the velocity.
One way around this is to consider your people example. People follow the rules above: the people flowing into an area must equal the people flowing out of it. However, if you impinge the flow of people, they move slowly. This slows the movement in the wide area even more. See any traffic jam for an example of this.
The other way around it would be a substantial change in density. If you include phase changes, this sort of thing can happen. In a typical power plant water cycle, the boiler heats water into steam which goes through the turbines. That steam is then cooled down and condensed into water, and the water is pumped through pipes back to the boiler. As a general rule, the cross sectional area the pipes carrying the steam is far higher than the cross sectional area of the pipes carrying the water. So this lines up with what you ask. However, the dominating effect is the cooling process. The pipes getting smaller is more of a side effect.
A fascinating place where you might see what you really want to see is in degenerate matter, like the stuff a white dwarf is made out of. The more mass you have, the smaller white dwarf matter gets (because its gravity pulls it tighter together). So if you had a flow of this stuff, then impinged it to cause it to all clump together, it would get more dense. This matter could then flow through that small tube slower.
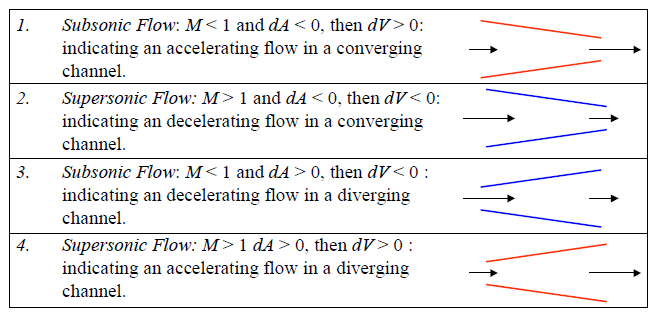
If pressure difference driving the flow is constant, then it is not obvious that introducing a constriction in the flow will necessarily increase the flow speed there (compared to the flow speed before the constriction was introduced). Flow driven by a constant pressure difference occurs for example when water flows through a pipe attached to an overhead tank (at least over a time scale in which the water level in the tank doesn't change significantly).
Say the flow rate $Q$ depends on pressure drop $\Delta p$ according to the following relation: $Q=B(\Delta p)^n$, in which $B$ is an empirical constant and $n>0$. The magnitude of $A$ depends on the geometry of the pipe (among other factors), and in particular on whether a constriction is present or not. Let $B_0$ be its value when there is no constriction, and $B_c$ its value when the constriction is present. Since constriction increases resistance to flow we must have $B_c\leq B_0$.
Let $A_0$ and $A_c$ be the cross-sectional area of the unconstricted and constricted portion of pipe respectively ($A_c\leq A_0$). When there is no constriction, the average flow speed $v_0=Q_0/A_0=(B_0/A_0)(\Delta p)^n$, and when there is constriction the average flow speed is $v_c=Q_c/A_c=(B_c/A_c)(\Delta p)^n$, assuming that the pressure difference across the pipe is the same in both cases. Therefore: $$\frac{v_c}{v_0}=\frac{B_c}{A_c}\frac{A_0}{B_0}$$
Now we know that when the area of the constriction becomes zero, there can be no flow, i.e $v_c=0$ when $A_c=0$. For this to happen without a jump, we must have the ratio $B_c/A_c\to0$ as $A_c\to0$, which means that asymptotically $B_c/A_c\sim A_c^m$ as $A_c\to0$, where $m>0$. Therefore we must have the following asymptotic behaviour: $$\frac{v_c}{v_0}\sim A_c^m\frac{A_0}{B_0},\quad m>0\quad (A_c\to0)$$
Therefore, for a given $A_0,B_0$, there is a particular value of the area of constriction $A_c$ below which the flow speed actually reduces compared to the case before the constriction was introduced. This argument doesn't assume a compressible flow.
It is well known that when water flows through a tube you can make it flow faster by making the tube narrow.
No it isn't. A tap is a tube with a section which can be made narrower or wider. Does water flow faster when you turn a tap off?
If you have a constant-volume flow of liquid through a tube, regardless of back pressure, then a narrower tube will require that liquid to flow faster. But this requires a pump (or other source) to force water down at a constant rate. If the liquid instead is flowing with constant pressure (a more normal situation) then the narrower tube will let less liquid through. Higher pressure will result in more flow, but it will still be reduced compared to a wider tube.
And this is exactly the same with people.
Your question only arises from you having a belief in how fluids flow which is incorrect. The situation you ask for does not require any special fluids - water will do fine.
Are there any fluids that flow slower in a constricted region compared to water?
Is any fluid that show this kind of behaviour and what would cause that?
A rheopectic fluid, such as printers ink, show a time-dependent increase in viscosity (time-dependent viscosity); the longer the fluid undergoes shearing force, the higher its viscosity and if shaken they solidify.
A non-newtonian fluid such as corn starch and water becomes thicker under stress. Some non-newtonian fluids get thicker and some become thinner, see the links for other fluids outside the scope of your question.
Shear thickening behavior occurs when a colloidal suspension transitions from a stable state to a state of flocculation. A large portion of the properties of these systems are due to the surface chemistry of particles in dispersion, known as colloids.
A non-Newtonian fluid is a fluid whose flow properties are not described by a single constant value of viscosity. Many polymer solutions and molten polymers are non-Newtonian fluids, as are many commonly found substances such as ketchup, custard, toothpaste, starch suspensions, maizena, honey, paint, blood, and shampoo.
In a Newtonian fluid, the relation between the shear stress and the strain rate is linear, the constant of proportionality being the coefficient of viscosity. In a non-Newtonian fluid, the relation between the shear stress and the strain rate is nonlinear, and can even be time-dependent. Therefore a constant coefficient of viscosity cannot be defined.
