What makes it a good idea to use RMS rather than peak values of current and voltage when we talk about or compute with AC signals.
-
5$\begingroup$ I don't know why the question was downvoted, it seems a perfectly reasonable question to me. $\endgroup$– John RennieCommented Oct 26, 2012 at 16:04
-
2$\begingroup$ @JohnRennie Check the edit history: the original text was confusing, but think that this was the question that anikumar had in mind. $\endgroup$– dmckee --- ex-moderator kittenCommented Oct 26, 2012 at 18:37
-
5$\begingroup$ Thank you for editing my question , Actually this is the question in my mind but I've some communication problem how to ask it $\endgroup$– anilkumarCommented Oct 29, 2012 at 4:48
-
3$\begingroup$ @JohnRennie because it's a "grubby" engineering question, as Gell-Mann would say. $\endgroup$– John McAndrewCommented Nov 1, 2012 at 22:31
3 Answers
Attempts to find an average value of AC would directly provide you the answer zero... Hence, RMS values are used. They help to find the effective value of AC (voltage or current).
This RMS is a mathematical quantity (used in many math fields) used to compare both alternating and direct currents (or voltage). In other words (as an example), the RMS value of AC (current) is the direct current which when passed through a resistor for a given period of time would produce the same heat as that produced by alternating current when passed through the same resistor for the same time.
Practically, we use the RMS value for all kinds of AC appliances. The same is applicable to alternating voltage also. We're taking the RMS because AC is a variable quantity (consecutive positives and negatives). Hence, we require a mean value of their squares thereby taking the square root of sum of their squares...
Peak value is $I_0^2$ is the square of sum of different values. Hence, taking an average value (mean) $I_0^2/2$ and then determining the square root $I_0/\sqrt{2}$ would give the RMS.
It's example time: (I think you didn't ask for the derivation of RMS)
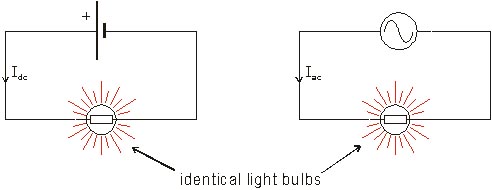
Consider that both the bulbs are giving out equal-level of brightness. So, They're losing the same amount of heat (regardless the fact of AC or DC). In order to relate both, we have nothing to use better than the RMS value. The direct voltage for the bulb is 115 V while the alternating voltage is 170 V. Both give the same power output. Hence, $V_{rms}=V_{dc}=\frac{V_{ac}}{\sqrt{2}}=115 V$ (But Guys, Actual RMS is 120 V). As I can't find a good image, I used the same approximating 120 to 115 V.
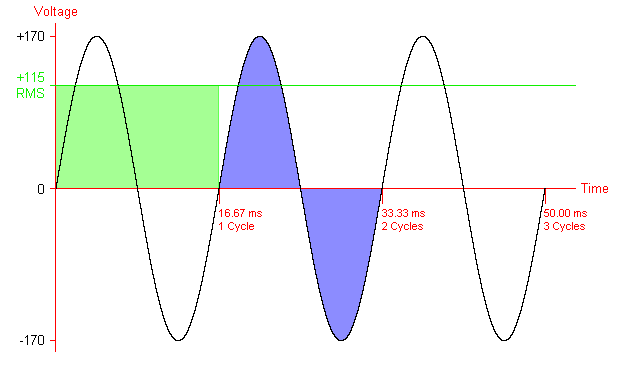
To further clarify your doubt regarding the peak value, It's simply similar to finding the distance between two points $(x_1,y_1)$ and $(x_2,y_2)$ in Cartesian system represented as, (sum of squares & then "root") $$d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}$$
-
1$\begingroup$ I've another question for you , in which cases average value of A.C is useful $\endgroup$ Commented Nov 1, 2012 at 11:17
-
1$\begingroup$ yes I know that RMS value is not the average value, But the average value of Ac sine wave is V(peak) x 0.637 not zero $\endgroup$ Commented Nov 2, 2012 at 4:26
-
1$\begingroup$ Another simple way to look at it, geometrically, is that by squaring V, in effect you flip the negative voltage to positive ((-V)^2 = V^2), then find the average magnitude of these square values. By taking the square root, you get back to an unsquared value that averages the magnitudes, regardless of sign. $\endgroup$– iSeekerCommented Sep 3, 2019 at 17:44
-
1$\begingroup$ I can't believe this answer has so many upvotes. The squaring has NOTHING to do with the negative values. The distance formula has NO RELATION to this problem. It is all about POWER. Alfred Centauri's answer is correct. In simple terms, P=V^2/R. We are equating AC and DC POWER. You have to square the voltage. Search "Why use RMS instead of the average voltage of half the cycle for power" at electronics.stackexchange.com for my answer with a drawing. $\endgroup$ Commented Jan 13, 2022 at 4:25
-
1$\begingroup$ “Attempts to find an average value of AC would directly provide you the answer zero... Hence, RMS values are used.” // That the average is zero doesn’t imply nor suggest to use RMS values. We could easily use peak values instead. $\endgroup$ Commented Jan 27, 2022 at 12:09
What makes it a good idea to use RMS rather than peak values
The rms value, not the peak value, is the equivalent DC value that gives the same average power.
Recall that power is the product of voltage and current:
$p(t) = v(t) \cdot i(t)$
For a resistor, we have:
$p(t) = R[i(t)]^2$
To find the average power, we must take the time average of both sides:
$p_{avg} = R \dfrac{\int_{T_1}^{T_2} {[i(t)]}^2\, dt}{T_2-T_1} $
You'll recognize the fraction on the right hand side as the mean of the square of $i(t)$.
Denoting $i_{rms}$ (the root of the mean of the square) as:
$i_{rms} = \sqrt{\dfrac{\int_{T_1}^{T_2} {[i(t)]}^2\, dt}{T_2-T_1}}$
we have:
$p_{avg} = R[i_{rms}]^2$
For DC, we have:
$p = R I^2$
So, we see that the rms value of the time varying current produces the same average power, for a given resistor, as a constant current of that value.
This is what makes the rms value "a good idea".
In many applications we are interested in the power. For example your electricity bill is based on the power you consume. For a DC source the power is:
$$ W = VI = \frac{V^2}{R} $$
and for an AC source (assuming a resistive load so the voltage and current stay in phase):
$$ W = V_{rms}I_{rms} = \frac{V_{rms}^2}{R} $$
So using the RMS values makes the power easy to calculate. The RMS values are, in a sense, the equivalent to the values in a DC circuit.
-
$\begingroup$ In case of resistive load ,is the formula W=VI applicable at any instant of voltage and current value? $\endgroup$– user184271Commented Mar 2, 2018 at 2:04
-
$\begingroup$ @harambe yes. The power I'm calculating is the average power over a cycle, but the instantaneous power is just $W=IV$ where $I$ and $V$ are the instantaneous values of current and voltage. $\endgroup$ Commented Mar 2, 2018 at 6:00
