Blackbody radiations

Question

The energy absorbed per unit time by the body at an absolute temperature $T_1$ and kept at a surrounding of higher temperature $T_2$ is $$J=\epsilon\sigma A T_2^4.$$

What my question is that aren't there many objects in the surrounding emitting radiations at temperature $T_2$ and applying Stefan-Boltzmann law for all of them wouldn't the radiations absorbed be greater than J(above)?

Kinda like if you have a blackbody at the center of the spherical shell then shouldn't the shell focus all the radiations on the blackbody effectively heating it beyond the temperature of shell,

$$ s_1 - s_2 = \tfrac{1}{2}t^2 \mu g \left( -\tfrac{1}{2} + \frac{M+m}{M} - \frac{m}{2M} \right) $$

Answer 1

if you have a blackbody at the center of the spherical shell then shouldn't the shell focus all the radiations on the blackbody effectively heating it beyond the temperature of shell

That's not what happens.

To see why, first consider the 2nd Law of Thermodynamics. Your outer shell would be raising, via a thermal process, the temperature of the center body to one higher than the outer shell's own temperature. Some engine could then do work by moving heat from the center body back to the outer shell, creating work in violation of the 2nd law.

To look at it at a more microscopic level, you have to be careful with calculating the geometry. Its not true that all the blackbody radiation from the outer shell is "focused" on the inner body. First, there's no "focusing": the radiation moves in straight lines. Second, not every ray of radiation from the outer shell hits the inner body: Some skim past it and hit the outer shell in some other point.

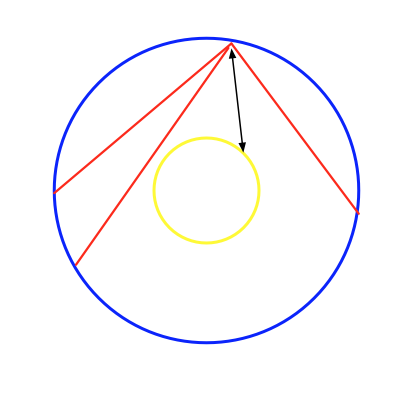
Red shows rays that go from outer to outer without hitting innner; black shows ones that go from outer to inner, but also vice-versa. In fact, there are exactly as many rays that go from the inner shell to the outer as there are from the outer to the inner. Once you precisely calculate both areas and solid angles, you'll find that, at equal temperatures, equal energy goes in both directions. Equal temperature is the result.

Answer 2

A spherical perfect black body $B_1$ of temperature $T_1$ completely surrounded by another perfect black body $B_2$ of temperature $T_2$ emits $J_e = \sigma A T_1^4$ and absorbs $J_a = 4 \pi \sigma A T_2^4$. For imperfect black bodies, denoting their absorptivities by $0 \lt \alpha_i \lt 1$ and emissivities by $0\lt\epsilon_i\lt 1$ then we get $J_e = \epsilon_1 \sigma A T_1^4$ and $J_a = \alpha_1 \epsilon_2 \sigma A T_2^4$. If $B_2$ incompletely surrounds $B_1$, that is, if it extend a solid angle of $\Omega \lt 4 \pi$ then $J_a = \Omega \sigma A T_2^4$.