Can the continuity equation and Bernoulli contradict each other?

Question

Please bear with me - I'm a lapsed mathematician and I'm self-studying these concepts.

A question asks the following:

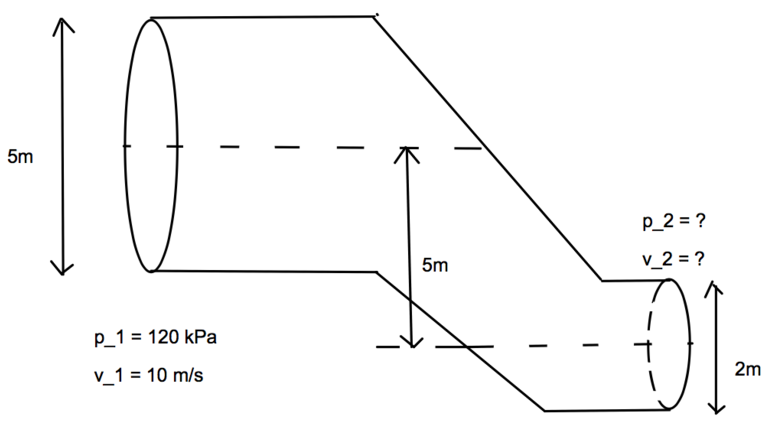
Water flows in a pipe of diameter 5 m at a velocity of 10 m/s. It then flows down into a smaller pipe of diameter 2 m. The height between the centre of pipe sections is 5 m. The density is assumed to be uniform over the cross sections. The gauge pressure at Boundary 1 is 120 kPa. Calculate the velocity at the smaller pipe section.

There is no reason I can see to assume mass flow continuity doesn't apply, and using $v_1A_1 = v_2A_2$ one obtains $v_2 = 62.5$ m/s. However, using the Bernoulli equation while assuming atmospheric pressure at the smaller section, one gets $$ \frac{p_1}{\rho} + \frac{1}{2}v_1^2 + + gz_1 = \frac{p_2}{\rho}+\frac{1}{2}v_2^2 + gz_2 $$ $$ 120+ 50 + 5g = 0 + \frac{1}{2}v_2^2 + 0 $$ $$ v_2 = 20.93, $$ and in fact this is what the textbook answer gives. I am quite confused as to why mass continuity applies in other situations, even with changes in pressure, but doesn't seem to apply here.

---

My question is: is this textbook question then ill-posed? I feel as though by providing too much information about the pipe section without checking the calculations, the question is bound to create a contradiction. The 5m/2m diameters don't actually make it into the final answer.

EDIT: I have added below the only answer I'm satisfied with that makes sense of the data. Please correct me as you see fit.

Answer 1

We assume water is incompressible so $\rho = $ constant $ = 1000 \text{ kg m}^{-3}$. Using mass continuity, and assuming both pipes are full, $$ v_1 A_1 = v_2A_2 $$ $$ 10 \cdot \pi(2.5)^2 = v_2 \cdot \pi(1)^2 $$ $$ v_2 = 62.5 \text{ ms}^{-1} $$ However, we may check the resulting gauge pressure at the second boundary using Bernoulli and find $$ 120 + \frac{1}{2}(10)^2 + 5g = \frac{p_2}{1000} + \frac{1}{2}(62.5)^2 + 0 $$ $$ 170 + 5g = \frac{p_2}{1000} + 1953.125 $$ $$ p_2 = -1734 \text{ kPa} $$ This is impossible without cavitation occurring. We may proceed in one of two ways: either the assumption that both pipes run full is erroneous and the larger top pipe must run partially full, or the air bubbles formed from cavitation, being lighter than water, will rise to the top pipe. Both of these effectively have the same result: the top pipe can only be partially full.

We assume cavitation occurs at the hard low barrier of $-101$ kPa (gauge) at the second boundary. Then $$ 120 + \frac{1}{2}(10)^2 + 5g = -101 + \frac{1}{2}(v_2)^2 + 0 $$ $$ v_2 = 25.3 \text{ ms}^{-1} $$ Returning to mass continuity, assuming the lower pipe is full, $10A_1 = 25.3 \cdot \pi(1)^2$ and hence $A_1 = 7.95 \text{ m}^2$. With a bit of geometry, this translates to a depth of $2.12$ m in the top pipe - just under half-full.