I am trying to calculate the centripetal force for an enlongated object like a fan blade. Where do I measure to to get the distance from center. Would I measure from the center to the end of the blade? Also what if I had a mass such as a hammer in circular motion since this is an uneven distribution of mass where would I measure to from the center of rotation to get the distance from center?
$\begingroup$
$\endgroup$
1
-
2$\begingroup$ What do you mean by "the centripetal force"? Different parts of the system experience different forces. So there is no "the" centripetal force, in general. $\endgroup$– nasuCommented Jan 19, 2021 at 15:20
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
2
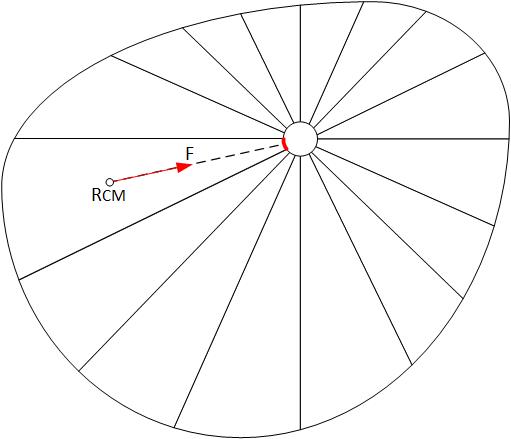
First, I would like to note that unless a rotating object has a circular symmetry and is rotated around the center of that circle, centripetal forces would not be the same in all directions.
This is illustrated below for an asymmetric object. If we wanted to know how centripetal forces are distributed along the perimeter of the axle, we would have to calculate them separately for each segment. These forces would not necessarily be the same for all segments, because the M*Rcm products are not necessarily the same for all segments.
With that in mind, the centripetal forces for a spinning board or a spinning hammer would have to be calculated separately for each segment of a rotating object, i.e., on either side of the axle, and these forces would be the same on both sides only if the center of rotation coincides with the center of mass of the whole object.
Now, when we concentrate on one segment at a time, we can calculate its mass (which would be a fraction of the mass of the whole object) and use the distance from the center of rotation to the CM of that segment as the radius of rotation, which will be used to calculate the centripetal force for that segment.
-
$\begingroup$ So basically you just measure to the center of mass. For example if I am swinging a hammer around with my arms extended and rotating in circles i would measure my distance to center from my center of rotation which would probably be my chest area to the point of where the handle meets the metal part of the hammer(center of mass). That would be my distance to center that I would use? The mass of the hammer would be the entire hammer including the handle? $\endgroup$ Commented Apr 26, 2018 at 14:23
-
$\begingroup$ @Physicsrocks Yes, your understanding is correct. The force you'll calculate will be the force between your hand and the handle of the hammer. To calculate the force at the shoulder, you would have to find CM between the hammer and your arm. I've just extended my answer to cover the cases when a hammer is spun around a random point on the hammer's body. $\endgroup$– V.F.Commented Apr 26, 2018 at 14:43
$\begingroup$
$\endgroup$
It depends what kind of curved motion you are studying: rotating around itself, or rotating around a point that doesn't belong to the object?
If you are studying the object's rotation around a distant point, i.e. that doesn't belong to itself, then it is simpler to reduce your odd-shape object to its gravity centre, noted G. There are formulae and approximations available to do this.
$\begingroup$
$\endgroup$
The centripetal force formula involves the local radius of curvature at a point on its trajectory. This radius of curvature is a measure of the local bending(in terms of path) undergone by the center of mass of the body in motion.
The distance you seek should therefore be from the local center of curvature of the trajectory of the body to its centre of mass(CM). To find the center of curvature, one draws a normal to the path at a cetain point on the path and locates a point that is at a distance equal to the radius of curvature along the normal.
In your example of a fan, center of curvature is clearly the fixed point right at the center of the fan where it is possibly attached to the ceiling. Hence you should consider the distance from this center to the CM of the fan as your radius of curvature in the centripetal force formula for the extended body.
$\begingroup$
$\endgroup$
For a point mass moving in a circle the centripetal force can be calculated as mr$ω^2$. For a rigid body rotating about an internal axis, each point has the same angular velocity (ω). The instantaneous x component for the net centripetal force would be - ${ω^2}ʃ(r_x)$dm (similar for y).
$\begingroup$
$\endgroup$
There are two points that matter. One is the pivot location or the center of rotation and the other is the center of mass.
The centripetal force is based on the distance between these two points.
answered May 28, 2022 at 3:25