0
$\begingroup$

This question is related to a previous question asked here.

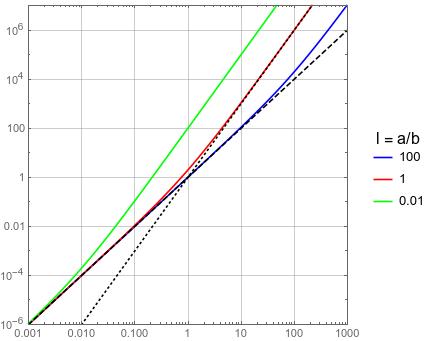
Power laws are scale invariant. They don't have a built-in or characteristic scale associated with them. Exponentials such as $e^{-x/\xi}$ are not scale-invariant. They have a characteristic scale $\xi$. What is the matter with polynomials such as $f(x)=ax^2+bx^3$ (where $x,a,b$ are all dimensional parameters with appropriate dimension)? Like exponentials, they too are not scale-invariant. But is there a natural scale associated with it? If yes, how does one find that hidden scale?

Improve this question
$\endgroup$
6
  • $\begingroup$ I guess the natural scale may be hidden in constants like $h$, for example. - as implied by answer just now by Steven and the other answer $\endgroup$
    – tom
    Commented Mar 21, 2018 at 16:42
  • $\begingroup$ @tom The question is how to identify the hidden scale. $\endgroup$
    – SRS
    Commented Mar 21, 2018 at 16:49
  • $\begingroup$ This seems to be a question about mathematics, not physics. $\endgroup$
    – sammy gerbil
    Commented Mar 21, 2018 at 17:31
  • $\begingroup$ @sammygerbil It's important to understand if one wishes to understand scale invariance in physics. Moreover, mathematicians (as far as I know) is not used to the language of "length scales", "characteristic scales" etc. And It's, of course, related to physics. See my question here physics.stackexchange.com/questions/394732/… $\endgroup$
    – SRS
    Commented Mar 21, 2018 at 17:34
  • $\begingroup$ So I looked at your Landau free energy scale question and I think I understand more about what you are asking. I will comment there. $\endgroup$
    – tom
    Commented Mar 21, 2018 at 17:43

2 Answers 2

Reset to default
3
$\begingroup$

Write your polynomial as $f(x) = ax^2 [1+ x/(a/b)]$. You see that $a/b$ is the scale at which the $x^3$ term takes over.

Take a look at the log-log plot of $f(x)$ for different values of $l=a/b$. The black lines are there to guide the eye. The dashed line corresponds $x^2$ and the dotted one to $x^3$.

enter image description here

Take a look at the red curve. It scales as $x^2$ for small arguments and as $x^3$ when $x$ is large. The cross-over scale is clearly visible in the middle.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Do you mean $a/b$ is the natural scale? I don't understand why. Does your argument hold good for polynomials of the form $f(x)=ax^2+bx^3+cx^4$ or of higher orders? You think there will be a single characteristic scale? @StevenMathey $\endgroup$
    – SRS
    Commented Mar 21, 2018 at 16:47
  • 3
    $\begingroup$ Yes. For $x\ll l$, the $x^3$ term can be neglected and $f \sim x^2$. For $x \gg l$, the $x^3$ term completely overwhelms the other and $f \sim x^3$. For higher order polynomials, you have more scales in the problem, $f(x) = a x^2 [1 + x/l_1 + (x/l_2)^2]$, with $l_1 = a/b$ and $l_2 = \sqrt{a/c}$. $\endgroup$
    – Steven Mathey
    Commented Mar 21, 2018 at 17:21
  • $\begingroup$ Can you slightly edit your answer? I've accidentally downvoted it, and cannot undo the downvote unless you edit it a bit. @StevemMathey $\endgroup$
    – SRS
    Commented Mar 24, 2018 at 17:29
3
$\begingroup$

There's a problem with $$ f(x)= x^2+x^3 $$ in that, if $x$ is not dimensionless, then various powers in your polynomials will have different dimensions and so cannot strictly be added. If of course you make $x$ dimensionless by dividing $x$ by some characteristic scale $a$, then you're back to the same argument as the exponential examples.

[See also this answer.]

Improve this answer
$\endgroup$
2
  • $\begingroup$ I've been careless in asking the question. Now I have modified the question. Is there a way I can identify some natural scale with $f(x)$? @ZeroTheHero $\endgroup$
    – SRS
    Commented Mar 21, 2018 at 16:20
  • $\begingroup$ This looks like a comment rather than an answer. $\endgroup$
    – sammy gerbil
    Commented Mar 21, 2018 at 17:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.