The shape of the Maxwell-Boltzmann distribution

Question

This question is about my misunderstanding of the differences between and shapes of the Boltzmann and Maxwell-Boltzmann distributions.

I understand that the Boltzmann distribution arises as the solution to the constrained maximum entropy optimisation, and that it is commonly written:

$$ p_i =\frac{e^{-E_i/kT}}{\sum_{j=1}^Me^{-E_j/kT}},$$

where $j$ and $i$ index the state of some system with energy levels $E_i$ increasing in $i$. $T$ is the temperature, which was introduced as the reciprocal of a Lagrange multiplier and $k$ is Botlzmann's constant.

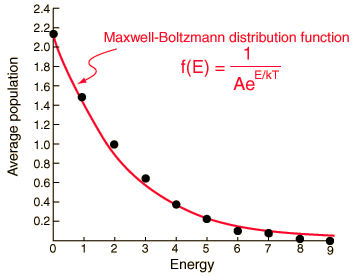
Now if someone were to ask me to draw the probability density function of the states for different energies, I would draw something similar to this:

In particular, the density would take its maximum value at $E=0$. When I read the wikipedia page for the Maxwell-Boltzmann distribution, they start with the distribution I have above and then proceed to find the distribution of momenta. They then transform the distribution back to a distribution of energy, but somehow the distribution now has a different shape. In particular:

$$ f(E) = 2\sqrt{\frac{E}{\pi}}\frac{1}{(kT)^{3/2}}\exp\Big(\frac{-E}{kT}\Big).$$

This expression clearly does not have the maximum value at $E=0$. Why is the new energy distribution different to the first one? Perhaps my failure to understand this is related to the condition they have: $f_E(E)\,dE=f_p(\mathbf{p})d^3\mathbf{p}.$

Answer 1

Your plot corresponds to the probability $p_i$ of occurence of the $i$-th microscopic state whose energy is $E_i$. At the macroscopic level, you cannot distinguish the different microscopic states but you can measure macroscopic quantities as the energy E. The probability to measure an energy $E$ is $$P(E)=\sum_i p_i\delta(E-E_i)=\Omega(E){e^{-\beta E}\over{\cal Z}}$$ where $$\Omega(E)=\sum_i \delta(E-E_i)$$ is the number of microscopic states whose energy is $E$. Usually, you have very few microscopic states with a low energy but this number increases with $E$. Multiplying by the Boltzmann weight which behaves in the opposite way, you get a curve which typically increases as $\Omega(E)$ for $E$ small and then decreases exponentially.

In the particular case of the Maxwell-Boltzmann distribution, i.e. for a classical ideal gas, we have for a single particle $$\eqalign{ P(E)&={1\over z}\int \delta\Big(E-{p^2\over 2m}\Big) e^{-\beta{p^2\over 2m}} {d^3\vec r d^3\vec p\over h_0^3}\cr &={V\over zh_0^3}\int_0^{+\infty} \delta\Big(E-{p^2\over 2m}\Big) e^{-\beta E} 4\pi p^2dp }$$ where $d^3\vec p$ has been written in spherical coordinates. Setting $u=p^2/2m$ and $p=\sqrt{2mu}$ so that $du=pdp/m$, we get $$P(E)={4\pi V\over zh_0^3}e^{-\beta E}\delta(E-u)\sqrt{2mu} \times mdu={4\pi V\over{\cal Z}h_0^3}m^3/2\sqrt Ee^{-\beta E}$$ Plugging now the expression of the partition function, we get $$P(E)={4\pi\over (2\pi k_BT)^{3/2}}\sqrt Ee^{-\beta E}$$

However, this is only for a single particle. The same calculation should be done for a gas of $N$ particles. $$P(E)={1\over {\cal Z}}\int \delta\Big(E-\sum_{i=1}^{3N}{p_i^2\over 2m}\Big)e^{-\beta\sum_{i=1}^{3N}{p^2\over 2m}} {d^{3N}\vec r d^{3N}\vec p\over h_0^{3N}}$$ The equivalent of spherical coordinates in a space of dimension $3N$ leads to ${\rm Cst}\ \!p^{3N-1}dp$ after integrating over the $3N-1$ angles. Therefore, I guess that we should expect something like $$P(E)={\rm Cst}\ \!E^{3N/2-1}e^{-\beta E}$$

Answer 2

The expression which you state as Boltzmann distribution and maximum at zero energy gives number of particles obtained energy of a system. At ground or zero energy level, probability is maximum equals to one. This description doesn't need energy levels, whether continuous or discrete. It means that as more and more particles have energy of a system, their number becoming less and less. Suppose a system has thermal energy of 300 k and there are 1000 particles. As 100 particles have that energy of system, probability to other particles to gain that energy becomes difficult. If 500 particles gain that energy of 300 k, it becomes more difficult to further increase total energy of a system.

The expression for Maxwell-Boltzmann distribution is differ from above in aspect that as previous one deals with population of particles and then multiple of constant energy of a constraint of a system gives total energy. While this expression is about energy obtained by a system per unit of energy. It is increasing then decreasing function so have a maxima which is average energy of a system. While previous is decreasing function and maxima is always at zero energy level.