I understand how moving slowly between equilibrium states allows for a process to be closer to reversibility, but I'm still a little confused why friction contributes to irreversibility. I saw an example of a block on an inclined plane that stated that after the block was pushed down the plane and back up (to it's starting position) it didn't cool back to its initial T which makes sense. However, I'm missing something with these piston examples that involve slowly removing and readding pebbles. In the picture I drew the gas has to do W to move the piston. If net work is W-Ffr then in both cases of going from A to B or back from B to A the net work should be the same (assuming friction doesn't change). If the net work is the same wouldn't U return to its original state and the process be reversible? 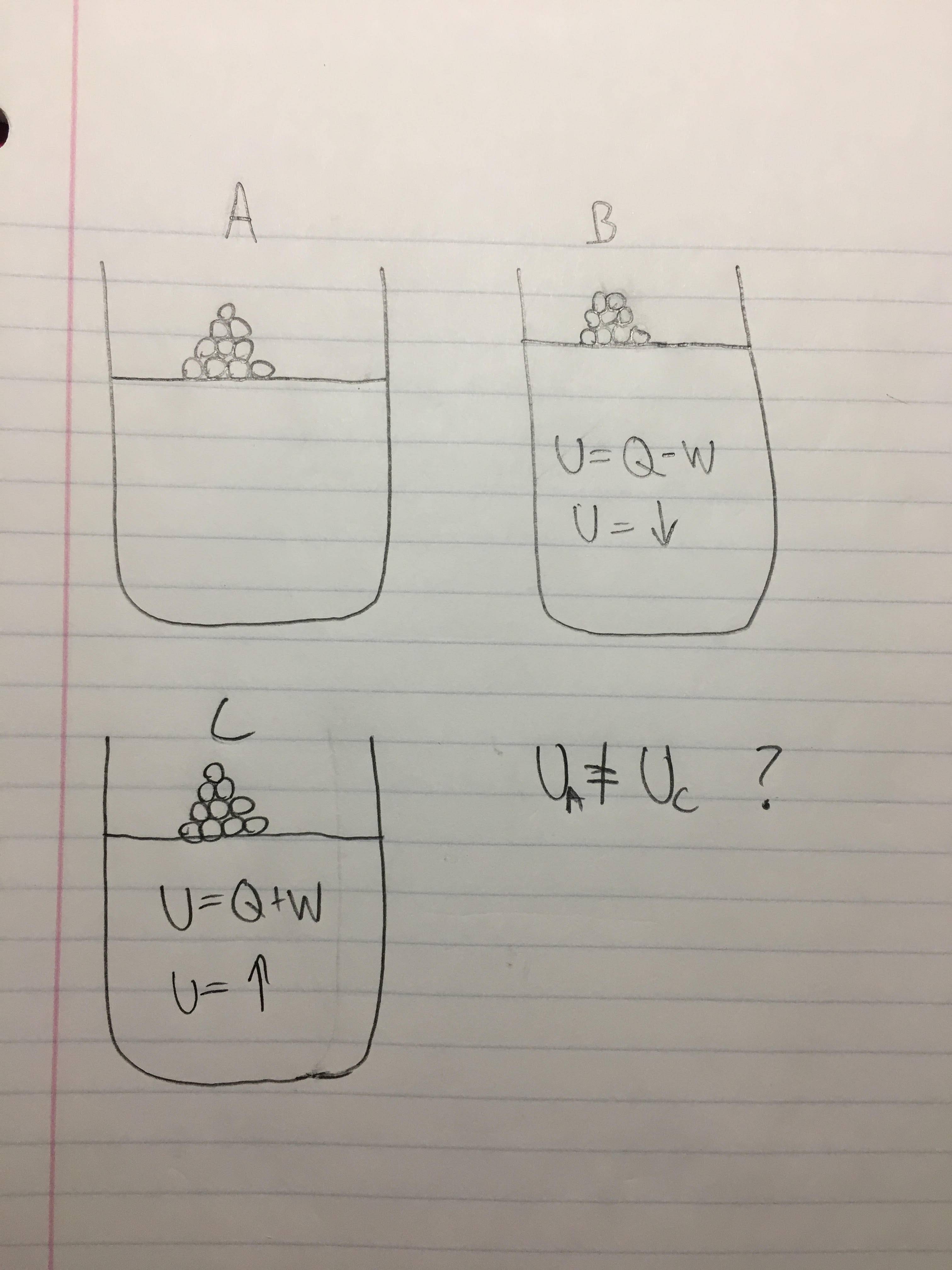
$\begingroup$
$\endgroup$
6
-
$\begingroup$ Actually, friction always does negative work. So, going from $A \to B$ and from $B \to A$, friction does almost the same amount of work both times, even their signs are same. Thus, their is always a net work against friction that can't be reversed. $\endgroup$– Yuzuriha InoriCommented Mar 15, 2018 at 5:54
-
$\begingroup$ My confusion is that when the gas expands it does work hence U=Q-W and when it's compressed work is done on it and U=Q+W. Friction does negative work against the motion of the piston but the net work either done by the gas or on the gas is still positive and equal (if friction is equal) which means U returns to the same value. $\endgroup$– user177470Commented Mar 15, 2018 at 6:07
-
$\begingroup$ I think my answer here will help you. (The question is very similar to yours I think.) $\endgroup$– N. VirgoCommented Mar 15, 2018 at 6:10
-
$\begingroup$ You are missing a friction component in the equations. physics.stackexchange.com/questions/247467/… might help you out $\endgroup$– Yuzuriha InoriCommented Mar 15, 2018 at 6:14
-
$\begingroup$ Did you forget that the friction force changes direction when you go from expansion to compression? $\endgroup$– Chet MillerCommented Mar 15, 2018 at 12:41
|
Show 1 more comment
