Suppose we have two twins travelling away from each other, each twin moving at some speed $v$:
Twin $A$ observes twin $B$’s time to be dilated so his clock runs faster than twin $B$’s clock. But twin $B$ observes twin $A$’s time to be dilated so his clock runs faster than twin $A$’s clock. Each twin thinks their clock is running faster. How can this be? Isn’t this a paradox?
The answer to this is that our twins, $A$ and $B$, are not measuring the same thing on their clocks. Since they are not measuring the same thing there is no paradox in the fact that each twin thinks their clock is running faster.
I’m going to try and give an intuitive feel for what is going on, and to do this I’ll use an analogy. This is going to seem a bit odd at first but bear with me and I hope everything will become clear.
Suppose I, Albert, and my two friends Bill and Charlie are all in cars driving at $1$ metre per second. I am driving due North, Bill is driving at an angle $\theta$ to my right and Charlie is driving at an angle $\theta$ to my left:
Consider how fast we are travelling North, i.e. the component of our velocity in the North direction. I am travelling North at $1$ m/s while my friends are travelling North at $\cos\theta$ m/s, so my friends are travelling North more slowly that I am.
Now it turns out that our compasses have the odd feature that they show North as the direction in which our cars are travelling. That means both Bill and Charlie also consider themselves to be travelling North. Let’s have a look at the situation from Bill’s perspective:
Bill considers himself to be travelling North at $1$ m/s while from his perspective I am travelling North more slowly, at $\cos(\theta)$, and Charlie is travelling North even more slowly, at $\cos(2\theta)$ m/s. And for completeness let’s show Charlie’s view:
Like Bill, Charlie considers himself to be travelling North at $1$ m/s while he considers me to be travelling North more slowly, at $\cos(\theta)$, and Bill to be travelling North even more slowly, at $\cos(2\theta)$ m/s.
So all three of us think they are travelling North faster than the other two. Let me emphasise this because this is the key point in my argument:
Everyone thinks they are travelling North faster than everyone else
Now this isn’t rocket science. The reason we all think we are travelling North fastest is because we have different ideas of what direction North is in. But this is exactly what happens in special relativity if we replace the direction North in our diagrams by the time direction. And the reason everyone thinks everyone else’s time is dilated is because we all disagree about the direction of the time axis.
In special relativity we typically use spacetime diagrams with the time axis vertical and the $x$ axis horizontal (we omit the $y$ and $z$ axes because it’s hard to draw 4D graphs). I’ll get out of my car, so I’m not moving, then if I draw my spacetime diagram it looks like this:
Although I’m no longer in the car I am still moving up the time axis because of course I’m moving through time at one second per second. So we have a diagram much like the one I started with except now the vertical direction is time not North, and I’m moving in the time direction not the North direction.
Bill and Charlie are moving away from me along the $x$ axis at speeds $+v$ and $-v$ just like the twins in the question:
But, and this is the key point, what special relativity tells us is that for a moving observer the time and x axes are rotated relative to mine. Specifically, if the other observer is moving relative to me at a speed $v$ then their time axis is rotated by an angle $\theta$ given by:
$$ \tan\theta = \frac{v}{c} $$
So if I draw Bill and Charlie’s time axes on my graph I get:
Hopefully you can now see the point of my analogy. In Bill and Charlie’s rest frames they are stationary, so as far as they are concerned they are moving up the time axis at $1$ second per second just like me. But because their time axes are rotated relative to me I observe them to be moving in the time direction at less than $1$ second per second i.e. their time is dilated relative to mine.
Bearing in mind my analogy, to find out what Bill observes we rotate everything to the left to make Bill’s time axis vertical, and now Bill considers himself to be moving up the time axis fastest. Likewise we rotate to the right to make Charlie’s time axis vertical, and we find that Charlie considers himself to be moving up the time axis fastest.
And this answers our question. All three of us think we are moving through time fastest, and the other two people’s time is dilated, because when we measure time we are all measuring time in a different direction. Our clocks differ because we are measuring different things.
This effect (B is slower from p.o.v of A and vice versa) does not seem very mysterious and can be observed even in a very simple model. The effect is the direct consequence of Einstein – synchronization of clocks in an observer’s frame of reference.
To demonstrate that, let us consider the behavior of objects that, although slow-moving, nonetheless act in accordance with the laws of the special theory of relativity.
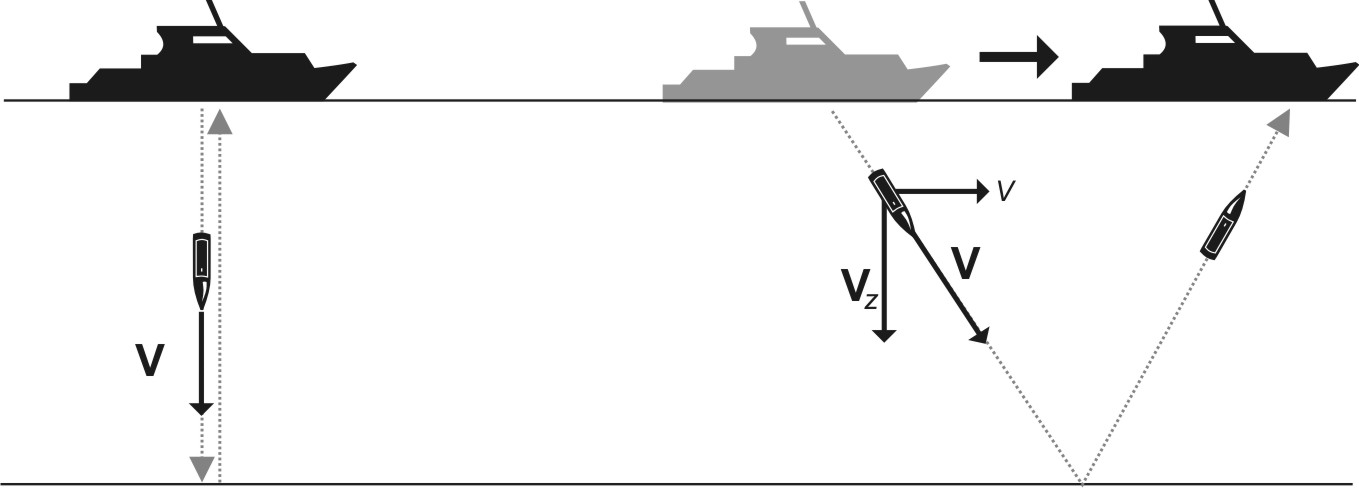Fig. 1. The ship on the left is at rest on the water surface. A shuttle moves at a velocity of $V$ from a ship to the bottom and back. The ship on the right is moving at a velocity of $v$ along the water body surface. The speed of movement of the shuttle equals $V$, the shuttle’s horizontal velocity component equals $v$, and the vertical component, $V_Z$, equals $V \sqrt{1-(v/V)^2}$
Let’s imagine the surface of a flat-bottomed water body with a depth of $h$, filled with still water. A ship equipped with a pendulum clock and with instruments that operate based on signals generated by this clock (in time with this clock) is located on the water body surface. A high-speed shuttle that is in continuous motion along a plumb line (relative to a given ship) between the ship and the bottom performs the function of the clock’s pendulum. Each shuttle trip to the bottom and back requires a time of $Δt = 2h/V_Z$ , where $V_Z$ – rate of descent and ascent of the underwater shuttle, and is accompanied by a change in the clock reading. The shuttle moves at a constant speed of V relative to the water, and if the ship is at rest, the shuttle moves perpendicular to the bottom, and the rate of the shuttle’s descent and ascent, $V_Z$, equals $V$. The time, $Δt$, of a shuttle trip to the bottom and back equals $2h/V$. The $V$ velocity value exceeds the ship’s speed of $v$; i.e., the condition $v < V$ is satisfied.
If a ship is proceeding at a speed of $v$, the clock tick rate and the operating speed of the instruments on the ships are decreased. This occurs due to the fact that when a ship is moving at a speed of $v$, the ascent and descent rate, $V_Z$, of a shuttle making trips in the water between a ship and the bottom of the water body according to the hypotenuses of right triangles happens to equal $V \sqrt{1-(v/V)^2}$ . Time on the ship in motion, which can be called simulated time, $t'$, passes more slowly than time, $t$, on the ship at rest also by $1 \sqrt{1-(v/V)^2}$ times. Thus, the more rapidly a ship proceeds through the water, the less often the pendulum “swings” and the more slowly the operations of the instruments located on this ship are performed, the operating speed of which is proportional to the shuttle pendulum frequency.
It is easy to simulate time dilation using ships of this type.
Let us assume that two ships at rest are located on a water surface at some distance from one another. Let’s imagine that the ships are equipped with speedboats that, like the shuttles, run at a speed of $V$, but only on the water surface. Let us assume that the ship instruments synchronize the clocks using a speedboat to transmit the information, which runs from one ship to the other and back. If the instruments have information that the speed of the boat relative to ships in opposite directions are equal, then using the boat, the instruments synchronize the clocks, as is done using a light signal in the special theory of relativity.
Having synchronized the clocks, the instruments on the ships at rest can compare their clock rate to that of a ship that is moving past them along the line that connects them. Taking the clock readings of the ship in motion at the locations of the ships at rest and comparing them to the readings of the synchronized clocks on their own ships, the instruments record time dilation of the moving ship $1 \sqrt{1-(v/V)^2}$ times.
Now imagine two ships under way one after another at a speed of $v$. Let’s assume that the first ship moves past a ship at rest at some point in time, then the second ship also moves past the ship at rest at some later point in time. Comparing the clock readings of the ship at rest with those of the previously synchronized clocks of their own ships, the instruments of the ships in motion detect a difference in the rate of their clock and that of the clock on the ship in motion. The result of a comparison of the clock on the ship at rest and the clocks on the ships in motion will depend upon the clock synchronization technique.
If the instruments on the ships in motion are able to measure the speed, $v$, of their ships, or if they have information concerning the fact that their ships are moving at a speed of $v$, then by synchronizing their clocks using a boat moving between the ships, they take into account the disparity of the speed of the speedboat they are using relative to their ships in the direction and opposite the direction of their movement. By synchronizing the clocks in this manner, they obtain a true result, according to which time on the ship at rest passes $1\sqrt{1-(v/V)^2}$ times more quickly than their own time.
However, this result can be just the opposite if the instruments on the ships in motion have no information concerning the movement of their ships and no other means of communication between the ships other than a speedboat. The truth of the matter is that by sending a boat that carries the requisite information from ship to ship, the instruments can only record the fact of the movement of the ships relative to one another. Basic calculations reveal that the instruments have no way of determining which ship is in motion and which ship is at rest relative to the water. If the instruments use false information concerning the repose of their ships, then mistaking their ships in motion relative to the water for ships at rest, they mistake the ship at rest in the water for a ship in motion relative to them. Here, they use the false condition of the equality of the boat’s speed relative to their ships in the direction of their movement and opposite it.
In this instance, by synchronizing the clocks using the Einstein technique, the instruments on the ships in motion, strange as it may seem, record a false time dilation on the ship at rest in the water, which in their estimation is moving relative to them.
Some references:
Dorling, J. „Length Contraction and Clock Synchronization: The Empirical Equivalence of the Einsteinian and Lorentzian Theories“, The British Journal for the Philosophy of Science, 19, pp. 67-9
Chapter 3.5.5 The reciprocity of the Lorentz transformation https://www.mpiwg-berlin.mpg.de/litserv/diss/janssen_diss/Chapter3.pdf
Simulation of Kinematics of Special Theory of Relativity by means of classical mechanics https://arxiv.org/abs/1201.1828
Here are some spacetime diagrams that display the symmetry of time dilation.
These diagrams underlie the various analogies one can use to motivate the symmetry.
First, we draw spacetime diagrams on rotated graph paper so that we can more easily visualize the ticks along the inertial observer worldlines.
In our example,
our observers have relative velocity of $v/c=\tanh\theta=(6/10)$,
and the corresponding time-dilation factor is $\gamma=\cosh\theta=(10/8)$,
where $\theta$ is the Minkowski-angle [the "rapidity"] between the timelike-worldlines.
We have drawn the diagram from Alice's frame. Alice regards P and P' as simultaneous, whereas Bob (traveling with velocity (6/10)c with respect to Alice) regards Q and Q' as simultaneous.
Note that:
In my diagram, the "light-clock diamonds" have lightlike edges and equal area. In addition, the diagonals of the "light-clock diamonds" are Minkowski-perpendicular to each other.
By drawing in the hyperbolas with center at the meeting event $O$,
one can see that $PP'$ is tangent to that hyperbola at the event $P$, where "radius vector" $OP$ meets the hyperbola. Similarly, $QQ'$ is tangent to that hyperbola at the event $Q$, where "radius vector" $OQ$ meets the hyperbola.
To see that this "tangent is perpendicular to radius" construction is analogous to the Euclidean construction (and to see the Galilean analogue), play around with my visualization https://www.desmos.com/calculator/wm9jmrqnw2 by tuning the E-parameter.
(In this visualization, time runs to the right [like the standard position-vs-time graphs].)
The first diagram is based on Fig. 17 in my paper "Relativity on Rotated Graph Paper" [American Journal of Physics 84, 344 (2016)] http://dx.doi.org/10.1119/1.4943251
This is often expressed as "Each of the twins thinks his clock is moving faster". However a more precise way to say it would be "Each of the twins thinks his clock is moving faster when observed in his own coordinate system."
The difference is important in that if the travelers understand relativity, they will know that their observation only applies into their own coordinate system. They can also calculate and agree with what the other traveler thinks, so they are not disagreeing.
An analogy can be made with movement. When traveler A looks out of his window and sees distance to B's ship increasing, he might think "I'm moving and he is staying where he is". But B can think exactly the same. And yet, both of them understand that their observations are not in conflict, because movement is always relative. Another example from Wikipedia:
While this seems self-contradictory, a similar oddity occurs in everyday life. If person A sees person B, person B will appear small to them; at the same time, person A will appear small to person B. Being familiar with the effects of perspective, there is no contradiction or paradox in this situation.
Another important part of the different coordinate systems is that there is no direct way to simultaneously measure the clock times when they are not next to each other. Because light speed is the maximum speed of any information, what you see of the other clock is delayed more and more as it goes further away.
However, if traveler B decides to turn his ship around and catch up with A, the situation changes. Traveler B's coordinate system now changes as his speed is changing. This breaks the symmetry. By the time when B catches up with A, both of them will observe that B's clock is lagging behind A's clock.
I'm assuming the ships take off simultaneously from earth, with all three clocks set to 0. Events connected by blue lines are simultaneous according to the observer on earth. Events connected by red lines are simultaneous according to the observer on ship A. Events connected by green lines are simultaneous according to the observer on ship B:
(Note: these times are approximations; to make this fully realistic, I'd have to show events happening at times like 1:47, which I'll have rounded off to 2:00.)
The earthbound observer says things like this:
I see by my clock that it's now 4:00. At this moment both ship clocks say 3:00. They are running slow.
Or
I see by my clock that it's now 8:00. At this moment both ship clocks say 6:00. They are running slow.
The captain on Ship A says things like:
I see by my clock that it is now 4:00. At this moment, the earth clock says 3:00. It is running slow. Also at this moment, the B-clock says 2:00. It is running even slower.
Or:
I see by my clock that it is now 8:00. At this moment, the earth clock says 6:00. It is running slow. Also at this moment, the B-clock says 4:00. It is running even slower.
The captain on Ship B says things like:
I see by my clock that it is now 4:00. At this moment, the earth clock says 3:00. It is running slow. Also at this moment, the A-clock says 2:00. It is running even slower.
Or:
I see by my clock that it is now 8:00. At this moment, the earth clock says 6:00. It is running slow. Also at this moment, the A-clock says 4:00. It is running even slower.
Where's the alleged paradox?
One way to understand relativity is to think of space time as being described by a geometry, in which $x^2+y^2=z^2$, with $x$ and $y$ being two legs of a right triangle, and $z$ as the hypotenuse, is replaced with $x^2-y^2=z^2$ with $x$ representing the distance in space between two events in spacetime, $y$ being the distance in time between two events in spacetime, and $z$ being the distance in spacetime between two events.
The distance in time between two events, if those two events are connected by a world line of an object that is in an inertial reference frame, is the proper time of the world line. So the proper time of a world line that is in an inertial reference frame can be expressed using the equation $\tau^2=-\left(\frac{\Delta_x}{c}\right)^2+{\Delta_t}^2$, with $\tau$ being the proper time, $\Delta_x$ being the objects displacement in space, $c$ being the speed of light, and $\Delta_t$ being the objects displacement in time.
If twin A and B are in inertial reference frames, and B is moving relative to A, then you could draw a right triangle with one of the legs representing the proper time of twin A, the other leg representing the displacement in space of twin B relative to A from the initial time for A to the final time for A and the hypotenuse being the proper time for B so ${\tau_b}^2=-\left(\frac{\Delta_{x_a}}{c}\right)^2+\tau_a^2$. The direction of an objects proper time is also the direction of that objects time axis so A and B also disagree on which direction is time and that is how they can both say that it is the other whose clock has slowed down. While different observers can disagree on the displacement in space, and the displacement in time they can agree on the distance in spacetime between two events.
It is not a paradox.
Consider the case of two poles 100m tall that stand on Earth a thousand km apart. You stand next to one and I stand next to the other. Owing to the curvature of the earth, the vertical direction in your frame of reference is not parallel to mine- the two are angled somewhat. If you measure the height of my pole in your frame of reference, you will say it is less than 100m, because the pole is leaning away from you. Likewise, if I measure the height of your pole in my frame of reference it will be less than 100m because it is leaning away from me. As measured in our respective frames of reference, we each find the height of the other's pole less than our own. There is no paradox- we are simply measuring quantities on a different basis.
Confusion about time dilation arises where people assume it means that time slows down. The effect arises because planes of constant time in one reference frame are all tilted with respect to the planes of constant time in any other reference frame moving relative to it. That means that as you move through a frame, the clocks you pass are progressive out of synchronisation, each being ahead in time of the last clock you passed. Your own clock seems to be running slow only because each of the clocks you are passing is running ahead of the last clock you passed.
To take a concrete example, imagine two trains, A and B, each of an engine and ten carriages, in each unit of which there is a clock. The clocks keep perfect time but have been set so that each clock as you move down the train is running one second behind the next.
Imagine now that the two trains pass head to head, meeting when their lead clocks say the time is 12:00:00. The trains are moving very slowly, so that it takes ten seconds to move past each carriage.
The driver in train A looks at each of the clocks he passes in the other train. The first he passes at 12:00:10, but the clock has been set 1 second ahead, so it reads 12:00:11.
The second clock he passes at 12:00:20, but that clock has been set 2 seconds ahead so it reads 12:00:22.
The next clock he passes shows 12:00:33, although his clock shows only 12:00:30.
His clock appears to be losing a second every ten seconds according the the clocks he is passing, ie to be time dilated, but really his clock is ticking at the normal rate, and the dilation is caused by comparing his clock with others that are out of synch.
The driver in train B has exactly the same experience. He passes the first carriage in train A at 12:00:10, but that clock has been set 1s ahead, so it reads 12:00:11. Likewise the next clock he passes shows 12:00:22, even though his clock shows only 12:00:20, and so on. So the driver in train B believes he is losing time compared with the clocks on train A.
Each driver, therefore, sees that his own clock appears to be losing progressively more time compared with the clocks he is passing on the other train. It is not a paradox. Each drivers clock is keeping the correct time, but they are passing clocks that are progressively out of synch.
This phenomenon follows directly from the principle of time dilation of special relativity:
Proper time = time before time dilation
Observed coordinate time = time after time dilation
That means in this case: When each twin observes his own clock, the observed coordinate time is the proper time (time dilation factor 1, that means absence of any time dilation). When he observes some other clock moving at a relative velocity with respect to himself, the time dilation is not one, it is bigger than one, that means that there is some time dilation.
Everything is clear once you remember that simultaneity is relative.
Let's look at a simple case where frame F' is moving to the right with velocity v with respect to frame F, in another words, F is moving at -v with respect to F'.
The Lorentz transformation in one direction is given by \begin{align} x' &= \gamma (x-vt) \\ t' &= \gamma (t - \frac{v}{c^2}x) \end{align}
If you fix $x'=0$, then $t'$ would be the time of the clock inside F'. Simple substitution shows \begin{align} x=vt &\Rightarrow t'=\gamma(t-\frac{v^2}{c^2}t)\\ &\Rightarrow t'=\frac{t}{\gamma} \end{align}
We usually denote this $t'$ with $\tau$ and call it the proper time because the "clock" is fixed to $x'=0$ in frame F'. We can see the time dilation in this case.
However, at time $\tau$ in frame F', we can ask the question of what does the observer at frame F' see at this moment on the clock sitting at the origin frame F? The key thing here is that at this moment means different things to F' and to F due to the relativity of simultaneity. In the Minkowski diagram, it is tilted.
If we fix $t'=\tau$ for some value of $\tau$, we can figure out what $t$ is at $x=0$. \begin{align} \tau&=\gamma(t-0)\\ \frac{\tau}{\gamma}&= t \end{align} Here, we can denote $t$ with something else such as $\overline{t}$ meaning it is what the F' observer sees on the clock at the origin of F. You can see that both observers think that there is a slowdown and at the root it works because simultaneity is relative.
The twin paradox may help. Before you say “No, that is asymmetrical!”, let me explain.
In the twin paradox one twin stays on earth while the other twin travels on a spaceship to a star and back. The twin who travels ages less than the twin who stayed on earth. Some then ask "But if we switch things around and consider that the twin on the spaceship was at rest and the earth and star did all the moving, don't we then find that the earth twin ages less?" And the answer is no, we don't. We get the exact same result. Whether the earth and star do the moving or the spaceship, the result is always that the twin on the spaceship ages less.
To best see what is happening, consider that the earth and star is a long rod, say 3 ly in length, and that the spaceship is traveling at 0.6c and traversing this rod from end to end.
Scenario 1: The ship is moving at 0.6c and passes the stationary rod. How long does it take the ship to traverse the rod? From both perspectives?
Scenario 2: The rod is moving at 0.6c and passes the stationary ship. How long does it take the ship to traverse the rod? From both perspectives?
Scenario 1 Result: From the rod's perspective the ship is going at 0.6c and the rod is 3 ly long and it takes 5 years. From the ship's perspective, the rod is 2.4 ly long (contracted) and the ship is going 0.6c and it takes 4 years.
Scenario 2 Result: From the ship's perspective, the rod is 2.4 ly long (contracted), the ship is going 0.6c, it takes 4 years. From the rod's perspective, the ship is going 0.6c and the rod is 3 ly long, it takes 5 years.
So you see, unlike what your hear, the twin paradox is quite symmetric in the sense that the principle of relativity applies (as it always should) and you can have the spaceship move or the earth and star move. Ok, now you say "But this is even worse! You showed symmetric scenarios but now the results are asymmetric!”
Well, the twin paradox is symmetric in the sense that it follows the principle of relativity, but there is an important detail, which distinguishes it from your scenario.
When two observers A & B pass each other at some velocity, we often say that A sees B's clock running slow and B sees A's clock running slow. And this is true. But what does "sees" mean? Well, one way to define it is like this. If A pulls out a ruler and times how long it takes B to traverse it, and B times this as well, A's time will be longer than B's time. Likewise, if B pulls out a ruler and times how long it takes A to traverse it, and A times this as well, B's time will be longer than A's time.
But each is using their own ruler and this represents two different scenarios that cannot be compared. Thus, no paradox (since you can’t compare the scenarios anyways). You can only compare the two results if they are based on one ruler.
In the twin paradox, we are only using one ruler (the rod) the whole time. Basically, the distance from the earth to the star. That ruler was ALWAYS in earth’s frame, even though we looked at it with the spaceship stationary and with the spaceship moving.
In your scenario, you have to settle on which way you are going to go. Are you going to use A’s ruler or B’s? Do you want A’s clock to be slower than B’s or the other way around.
Also, if you think through this some more, once you choose a ruler, the other observer sort of has to come back to that reference frame at some point for the different clocks to mean anything. Having the two observers go away from each other forever makes the case of clock differences moot.
How can time dilation be symmetric?
Suppose we have two twins travelling away from each other [...]
The sketch in the OP explicitly names $\mathsf A$ and $\mathsf B$ as main protagonists.
Suitably for discussing basic cases of time dilation, each $\mathsf A$ and $\mathsf B$ are apparently supposed to "move freely" in a flat region; accordingly $\mathsf A$ being a member of an inertial frame$^§$, and $\mathsf B$ being a member of another inertial frame.
To adequatly specify cases of time dilation, and to point out their symmetry, in this setup, the OP sketch can be expanded by explicitly naming one more participant, say $\mathsf P$, who is a member of the same inertial frame as $\mathsf A$ (in other words: who is and remains at rest wrt. $\mathsf A$), and one more participant $\mathsf Q$, who is a member of the same inertial frame as $\mathsf B$ (in other words: who is and remains at rest wrt. $\mathsf B$):
$$ \begin{matrix} \qquad \mathsf P \, ========= & \! \! \mathsf A \! \! & \longrightarrow \, v \qquad \qquad \qquad \qquad \qquad \qquad \cr \, \, \qquad \qquad \qquad v \, \longleftarrow & \! \! \mathsf B \! \! & ================== \, \mathsf Q \end{matrix} $$
Participants $\mathsf P$ and $\mathsf Q$ are selected "along the axis of motion"; such that eventually $\mathsf A$ and $\mathsf Q$ are going to meet and pass each other, and likewise, eventually $\mathsf B$ and $\mathsf P$ are going to meet and pass each other.
Let's denote as $u$ the speed of $\mathsf B$ and of $\mathsf Q$ wrt. ((all members of) the inertial frame of) $\mathsf A$ and $\mathsf P$, which is equally the speed of $\mathsf A$ and of $\mathsf P$ wrt. ((all members of) the inertial frame of) $\mathsf B$ and $\mathsf Q$. (The relation between the value $u$ and the value $v$ is of course pretty simple and easily derived -- key phrase: "velocity addition" -- but that's not necessarily the topic of this question.)
Now, explicit instances of time dilation referring to the sketched setup are:
(a)
$\mathsf A$'s duration from having met $\mathsf B$ until having met $\mathsf Q$ is less than $\mathsf B$'s duration from having met $\mathsf A$ until simultaneous to $\mathsf Q$ having met $\mathsf A$. Or symbolically, and more quantitatively:
$$ \frac{\boldsymbol \tau^{\mathsf A}{[ \, \circ \mathsf B, \circ \mathsf Q \, ]}}{\boldsymbol \tau^{\mathsf B}{[ \, \circ \mathsf A, \text{simultaneous to } \mathsf Q \! \circ \! \mathsf A \, ]}} = \sqrt{ 1 - \left( \frac{u}{c} \right)^2 }.$$
And likewise, or in other words: symmetric to instance (a):
(b)
$\mathsf B$'s duration from having met $\mathsf A$ until having met $\mathsf P$ is less than $\mathsf A$'s duration from having met $\mathsf B$ until simultaneous to $\mathsf P$ having met $\mathsf B$. Or symbolically, and more quantitatively:
$$ \frac{\boldsymbol \tau^{ \mathsf B}[ \, \circ \mathsf A, \circ \mathsf P \, ]}{\boldsymbol \tau^{ \mathsf A}[ \, \circ \mathsf B, \text{simultaneous to } \mathsf P \! \circ \! \mathsf B \, ]} = \sqrt{ 1 - \left( \frac{u}{c} \right)^2 }.$$
(A corresponding quantitative derivation was of course already given by Einstein 1905; specificly there. However, the notation I've used above hopefully makes the relevant symmetry better recognizable. Besides, I recommend the derivation shown here, sec. 2.)
This concludes my answer to the question stated in the OP title.
p.s.
"Twin $\mathsf A$ observes twin $\mathsf A$’s time to be dilated so his clock runs faster than twin $\mathsf B$’s clock. But twin $\mathsf B$ observes twin $\mathsf A$’s time to be dilated so his clock runs faster than twin $\mathsf A$’s clock. [...]"
This quoted description is defective.
Either -- perhaps trying to argue sympathetically -- the two described cases are meant to correspond to the two cases (a), or (b), resp., of my answer above. (Even though in my above answer there was no mentioning at all of "clocks", or even of "one clock running faster than another", or somesuch.)
If so, then the quoted description is poorly worded: it fails to convey the actual symmetry, and it can easily be mistaken for an absurd statement.
Or -- even worse: it is actually meant to be an explicitly absurd statement.
The reason why the twins will think that their clock is running faster or slower than the other twin's is, is because they are both unable to observe the other twin "from above" (like we do in the picture that you provided). They instead, observe each other while considering themselves as stationary!
But that's "wrong"...* Neither of them is stationary... They are both moving in spacetime. But since we have the equivelence principle, we can infact consider ourselves as stationary and think that they other guy is moving in space or in time, either faster or slower... That creates the "paradox" that you are asking about. Its not a paradox, is just shortsightedness...
(Its not really wrong, we are just unable to see spacetime "from above" like in the picture you provided, so we end up seeing all those effects of relativity, like time dilation and length contraction. When we observe both objects from "above" all those effects vanish).