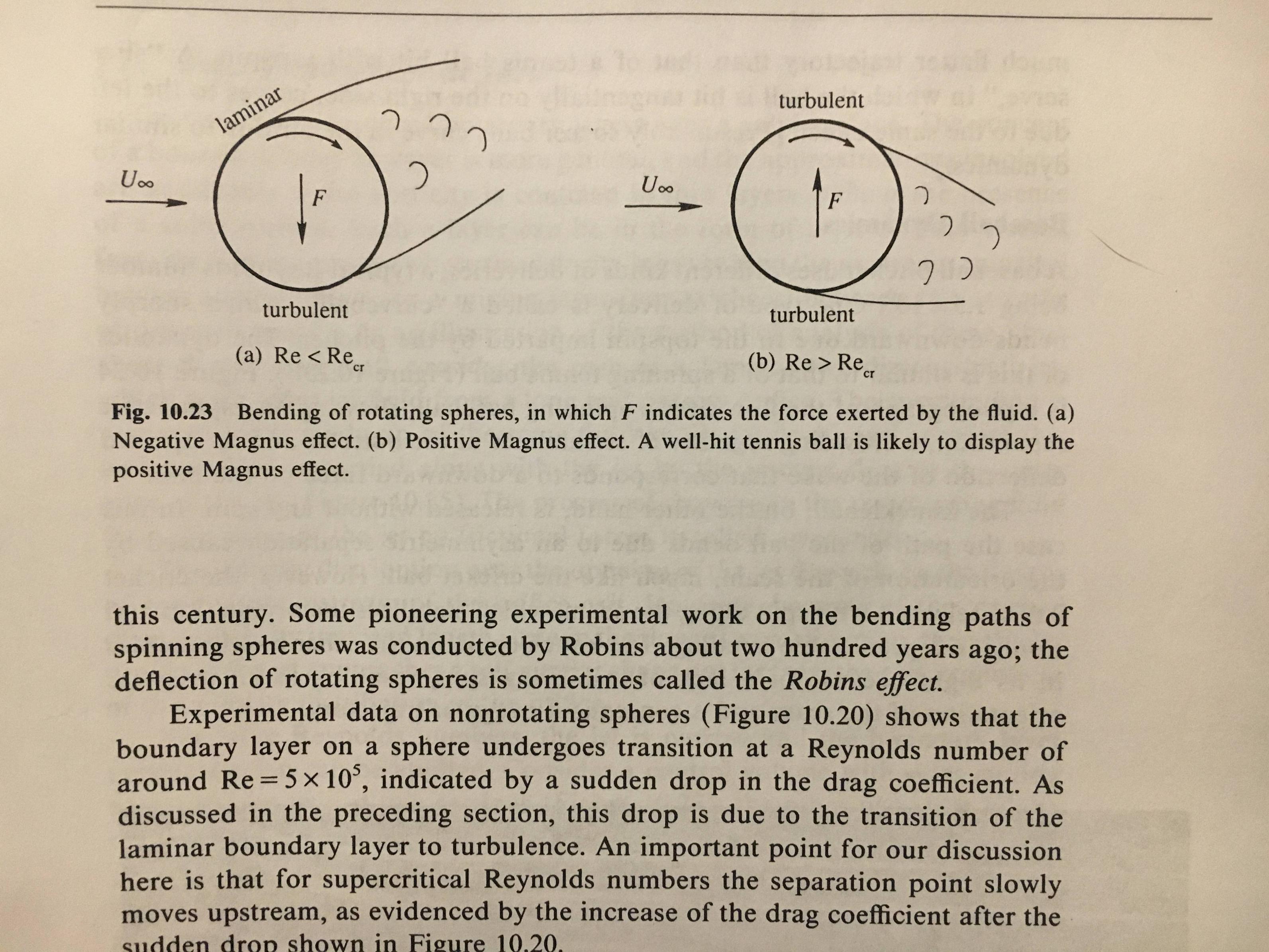
Supposedly, the Magnus effect, which is responsible for the curve of a curveball, and is the reason that tennis players hit their ground strokes with topspin, only acts above a critical Reynolds number ($\mathrm{Re}=Ud/\nu$, where $U$ is the velocity, $d$ is the diameter, and $\nu$ is the kinematic viscosity) of around $5 \times 10^5$. At lower Reynolds numbers, the force is supposed to be in the opposite direction: topspin would create lift, and backspin downforce--it's called the "negative Magnus effect".
My question is, why have I never seen any real life indication that this actually occurs? Further, why do real-life spinning spheres with significantly lower Reynolds numbers exhibit a positive Magnus effect?
For example, taking the kinematic viscosity or air as $1.5 \times 10^{-5}$ m$^2$s$^{-1}$, a ping pong ball, with $d =$ 40 mm and $U=$ 15 m/s, has Re $=4 \times 10^4$. Yet if you watch an elite table tennis match, the positive Magnus effect is very obvious. Similarly, an Airsoft BB, with $d =$ 6 mm and $U =$ 100 m/s, also has Re $=4 \times 10^4$. But Airsoft guns put backspin on the BBs because it flattens the trajectory due to the (positive) Magnus effect (it's called "hop-up" and there's a Wikipedia article on it).
I'm primarily getting this from the textbook from which I learned introductory fluid mechanics, by Kundu. A figure from the relevant section is included below. I love the book and have found it to be quite free of mistakes. What gives? Is this an uncharacteristic error? Or is there something about the real-world scenarios that don't allow the theoretical effect to manifest?