A simple paradox on conservation of energy

Question

Well this problem is challenging me, but seems ridiculously simple: Imagine we have a frictionless hill, and a mass of m which starts slipping down without an initial velocity from the height h. So when it reaches the horizontal ground at the end, it has the horizontal velocity $$v = \sqrt{2gh}$$ to the right. Now let's imagine the observer is moving with the speed of u to the left, so the mass has an initial velocity of u to the right so $$E = mgh + (mu^2)/2$$ On the other hand the mass had the speed of v at the end before, now it has the speed of v+u at the end so $$E = (m(v + u)^2)/2 = (m(\sqrt{2gh} + u)^2)/2 = mgh + (mu^2)/2 + u\sqrt{2gh}$$ so the energy at the beginning of the slipping and the end of it are not equal, and it means that an additional work of $$u\sqrt{2gh}$$ is done on the mass while slipping. But I can't figure out why and what is the force causing it.

Answer 1

According to the observer, the hill is moving to the right. The normal force exerted by the hill on the mass has a component pointing to the right. So in this frame, the normal force does work on the mass.

Answer 2

I just wanted to add the explicit energy balance relation in the moving frame because I found it interesting. The moving frame is not accelerating and so the forces are the same that we have in the fixed frame.

In the moving frame, at $t=0$, the mass moves horizontally with velocity $u$. Let's say the angle of the hill is $\alpha$. Right before hitting the floor, the velocity of the mass is $\vec{v_f} = (u \cos \alpha +\sqrt{2gh})\hat{i} + u \sin \alpha \hat{j}$, where $\hat{i}$ points in the falling direction of the hill and $\hat{j}$ is normal to the surface of the hill, pointing upwards. Notice that the angles in this expression come from the fact that we move horizontally while the hill has an inclination. Also, this velocity is what we would obtain from the regular Galilean transformation of the fixed frame.

The time taken by the mass to fall completely is the same in both frames, namely $t_f = \sqrt{\frac{2h}{g}}\csc \alpha$.

Between $t=0$ and $t=t_f$, the velocity of the mass is given by $\vec{v} = (u \cos \alpha + t g \sin \alpha)\hat{i} + u \sin \alpha \hat{j}$.

The normal is $\vec{N} = m g \cos \alpha \hat{j}$ and the weight looks a bit odd in this basis, $\vec{W}=m g \sin \alpha \hat{i} - m g \cos \alpha \hat{j}$.

The energy balance is then $\frac{1}{2} m u^2 + m g h + \int^{t_f}_0 \vec{N} \cdot \vec{v} \, \mathrm{dt} = \frac{1}{2} m \vec{v_f}^2$. There we see explicitly how the kinetic energy of the mass is changed by the work done by the weight, $\int^{t_f}_0 \vec{W} \cdot \vec{v} \, \mathrm{dt} = mgh$, and the work done by the normal, $\int^{t_f}_0 \vec{N} \cdot \vec{v} \, \mathrm{dt} = m u \cos \alpha \sqrt{2gh}$.

PS. Notice that something very interesting happens if the moving frame moves instead in the direction of $\hat{i}$! In that case, the normal does no work but the weight does an unexpected amount of work.

Answer 3

You can’t do the u+v.
Ignoring mass and 1/2:
$KE1=u^2$
$KE2=v^2$
Total $KE =u^2 + v^2$
Which does not equal $ (u + v)^2$

Answer 4

Let me expand on Ben's answer by giving a quantitative argument.

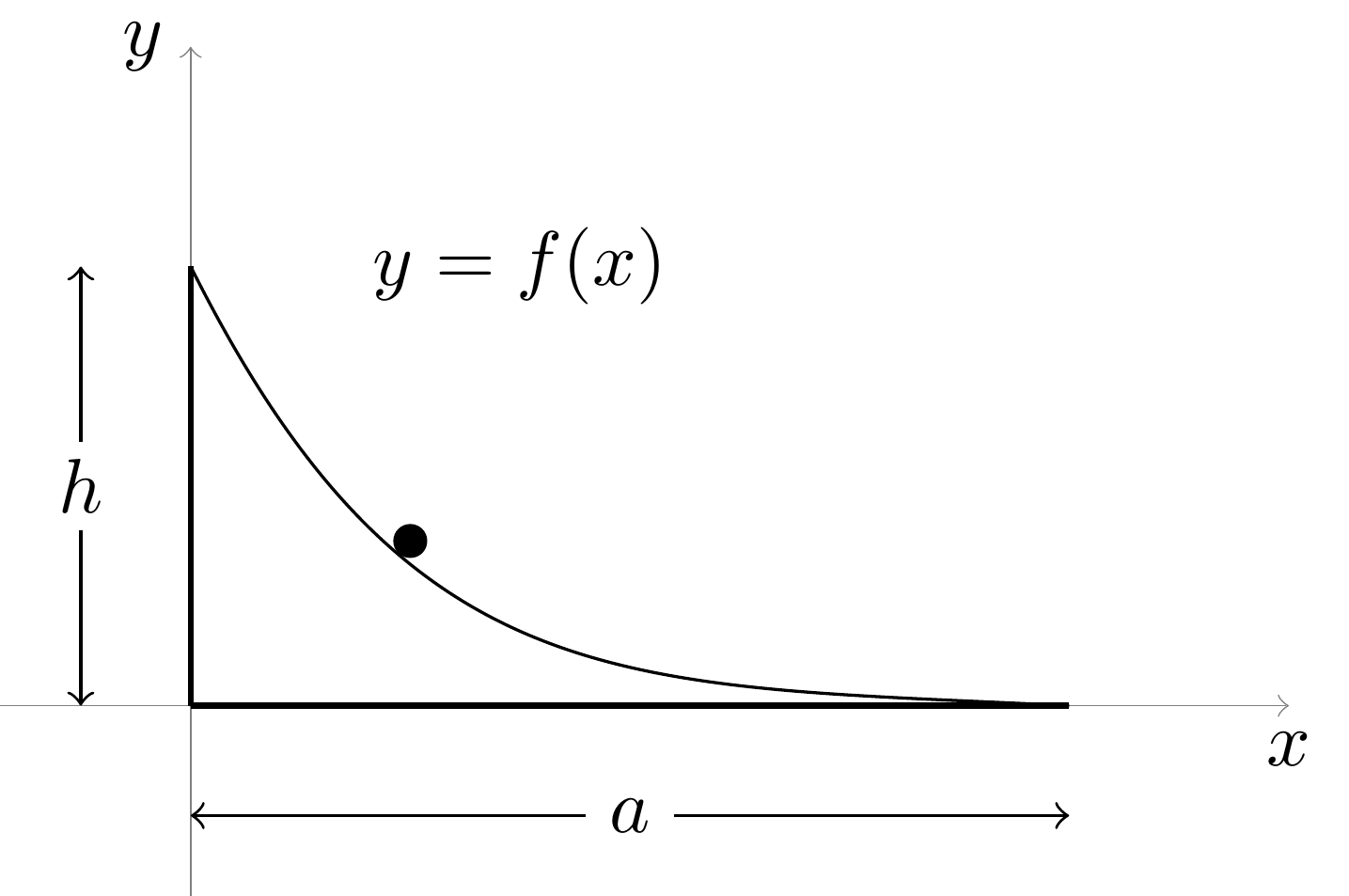
Let $x$ be the horizontal coordinate and $y$ the vertical one, such that, for instance, the gravitational acceleration is $\mathbf g=-g \mathbf{\hat y}$. We consider a profile $y=f(x)$ for a frictionless hill, where a point particle of unit mass is constrained to move. The function $f(x)$ is such that (letting $a$ be the base of the hill) $$ f(0)=h\,,\qquad f(a)=0\,,\qquad f'(a)=0 $$ and $h$ is the maximum of $f$ in the interval $[0,a]$. The gravitational potential energy is $U(y)=g y$.

$\bullet$ In a reference system which is stationary with respect to the hill, the trajectory $\mathbf r(t)=(x(t),y(t))$ of a point particle constrained to its surface satisfies: $$ \begin{aligned} y(t)-f(x(t))&=0\\ \dot y - f' \dot x&=0\\ \ddot y - f' \ddot x - f'' \dot x^2&=0\,. \end{aligned} $$ In particular, by the second equation, the trajectory is always tangent to the surface, whose unit normal to the constraint is $\mathbf n =(n_x, n_y)$ $$ n_x=\frac{-f'}{\sqrt{1+f'^2}}\,,\qquad n_y=\frac{1}{\sqrt{1+f'^2}}. $$ Namely, $$ \mathbf n \cdot \dot{\mathbf{r}}=0 $$ The Newton equation reads $$ \ddot{\mathbf{r}}=-g \mathbf{\hat y} + R \mathbf n $$ where $\mathbf R=R \mathbf n$ is the reaction of the constraint, and it is normal to it because the hill is frictionless. Then, multiplying by $\dot{\mathbf{r}}$, we get te usual conservation of mechanical energy $E$ $$ \dot E(t)=\frac{d}{dt}\left(\frac{1}{2}\dot{\mathbf{r}}^2+gy\right)=R \mathbf n\cdot \dot{\mathbf r}=0\,. $$ If the particle starts with zero velocity, the energy balance $E_0=g h=\frac{1}{2}v^2$ gives $v=\sqrt{2gh}$ for the final velocity.

$\bullet$ If now we choose a reference system which moves with respect to the previous one, with a constant velocity $-u \mathbf{\hat{x}}$, the previous equations get modified as follows. $$ \begin{aligned} y(t)-f(x(t)-ut)&=0\\ \dot y - f' (\dot x-u)&=0\\ \ddot y - f' \ddot x - f'' (\dot x-u)^2&=0\,. \end{aligned} $$ So, $$ \dot E(t)= R \mathbf n \cdot \dot{\mathbf{x}}=-\frac{u f'}{1+f'^2}(g+(\dot x-u)^2 f'')\,. $$ This equation can be integrated by employing the conservation of energy in the stationary frame, since $x-ut$ is indeed the trajectory in that frame by Galileian invariance: $$ dt = \sqrt{\frac{1+f'^2(x)}{2(E_0-U(f(x)))}} dx\,,\qquad \dot x^2=\frac{2(E_0-U(f(x))}{1+f'^2(x)}\,. $$ The result is $$ E(t)=E(0)+u\sqrt{\frac{2(E_0-U(f(x)))}{1+f'^2(x)}}\,\Bigg|_0^a\,. $$ Since $E_0=gh$, $f'(a)=0$ and $U(f(x))=g f(x)$, we finally find $$ E(t)=E(0)+u\sqrt{2gh}\,. $$ Imposing this with an initial velocity $u\mathbf{\hat{x}}$ and a final velocity $(u+v')\mathbf{\hat{x}}$, in the moving frame, yields $$ \frac{1}{2}(u+v')^2=\frac{1}{2}u^2+g h + u \sqrt{2 g h} $$ and this is solved by $v'=\sqrt{2gh}=v$, as desired.