0
$\begingroup$

For a pendulum, a= acceleration, y= displacement, w= angular velocity, T= time period. We have →|a| = yw² →a/y = w² = (2π/T) ² →2π/T = +√(a/y) or -√(a/y) →T= + 2π√(y/a) or -2π√(y/a). Is this correct that time period can be negative?

Improve this question
$\endgroup$
1

2 Answers 2

Reset to default
0
$\begingroup$

As far as I'm aware, The period is just the amount of time it takes for a whole oscillation. You can, for classical mechanics like this, just take the equations of motion and switch the time argument for $-t$, and still have the physics work, but the amount of time between oscillations is still going to be $T,$ and more to the point I'm not really sure why you would want a negative $T$ even if you justify defining one.

Improve this answer
$\endgroup$
0
$\begingroup$

Suppose we write the equation of motion as:

$$ y = A \sin(\omega t) = A \sin\left(\frac{2\pi}{T} t \right) $$

If the period $T$ is a positive number then the variation of $y$ with time looks like this:

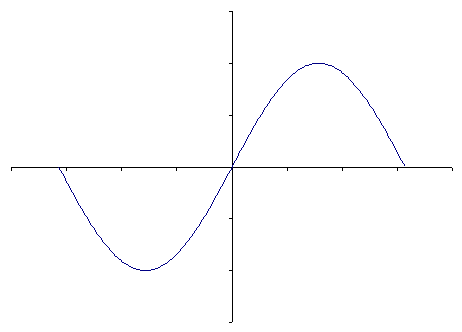
Positive T

If we keep the magnitude of $T$ the same but make it a negative number then we get this:

Negative T

which is the same graph just shifted along the time axis by $\pi$. So yes you can make $T$ negative if you wish. The initial conditions will determine which is correct i.e. is the velocity positive or negative when $t=0$?

Having said this, we wouldn't normally talk about a negative period. If we wanted the second graph we'd probably keep $T$ as a positive number and write the equation with a phase shift i.e.

$$ y = A \sin\left(\frac{2\pi}{T} t + \pi \right) $$

Improve this answer
$\endgroup$
1
  • $\begingroup$ But what is the physical meaning of negative time period? Is it the same as negative time in kinematics when we count the time in reverse? $\endgroup$
    – Mamta Bansal
    Commented Dec 16, 2017 at 5:41

Not the answer you're looking for? Browse other questions tagged or ask your own question.