Resonant frequency in RLC circuit

Question

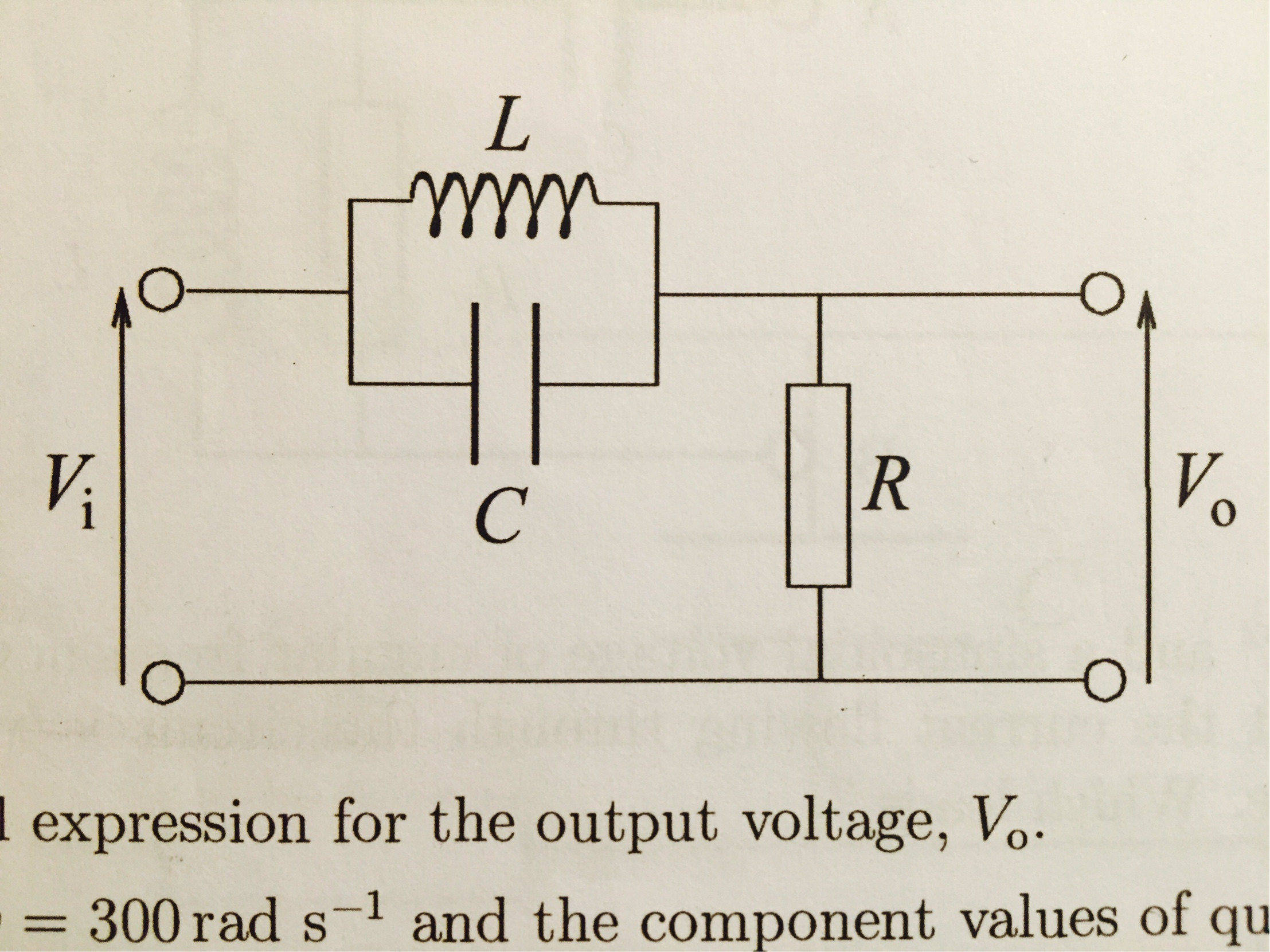
I know that in an RLC circuit the resonant frequency is the one that makes the total impedance purely resistive.

So for this circuit:

The impedance is $$ Z = R + \frac{\omega L}{1-\omega^2 LC}j $$,

So if I want to make purely resistive therefore making the imaginary part I zero, I can't get the $\omega_0 = 1\sqrt{LC}$ which I know is correct!

I assume the $Z$ you show is the open circuit input impedance, i.e, $Z_{11}$, of the two-port network shown? Keep in mind that the impedance of a parallel connected L and C goes to infinity on resonance. It can be argued that the phase is zero there. — Alfred Centauri, Commented Nov 28, 2017 at 21:56
Yes I'm using the open circuit impedance. But I don't understand then, when can I use the purely resistive impedance method and when do I have to do the maths to find the resonant frequency? — SuperCiocia, Commented Nov 28, 2017 at 22:14

Alfred Centauri · Accepted Answer · 2017-11-28 22:32:32Z

Place another resistor of resistance $R'$ in parallel with the inductor and capacitor. The open-circuit input impedance of the two-port is then

$$Z_{11}(\omega) = R + \frac{j\omega L}{(1 - \omega^2LC) + j\omega\frac{L}{R'}}$$

and see that this reduces to your equation for $R' \rightarrow \infty$

Now, see that when $\omega = \omega_0 \equiv \frac{1}{\sqrt{LC}}$, the impedance on resonance is

$$Z_{11}(\omega_0) = R + \frac{j\omega_0 L}{j\omega_0\frac{L}{R'}} = R + R'$$

which is purely resistive (real). Since this holds for any value of $R'$, argue that $Z_{11}(\omega_0)$ remains real for $R' \rightarrow \infty$.

1 Answer 1