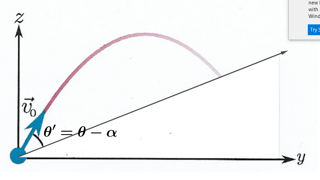
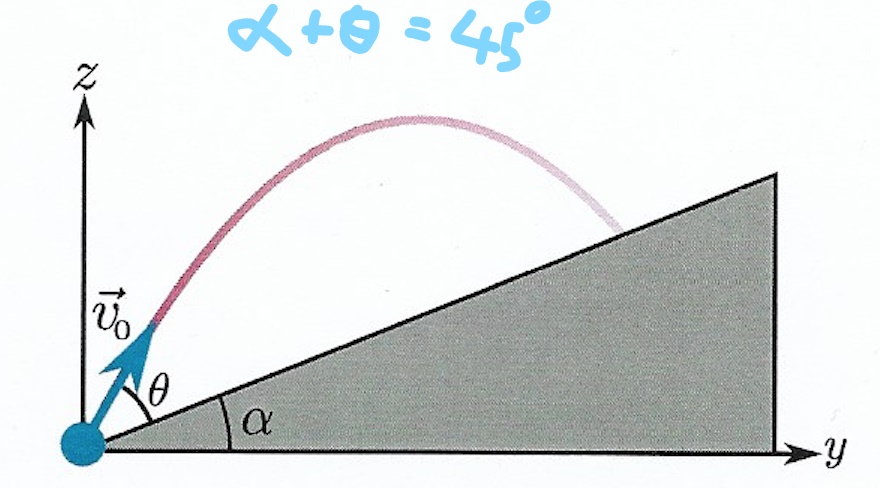
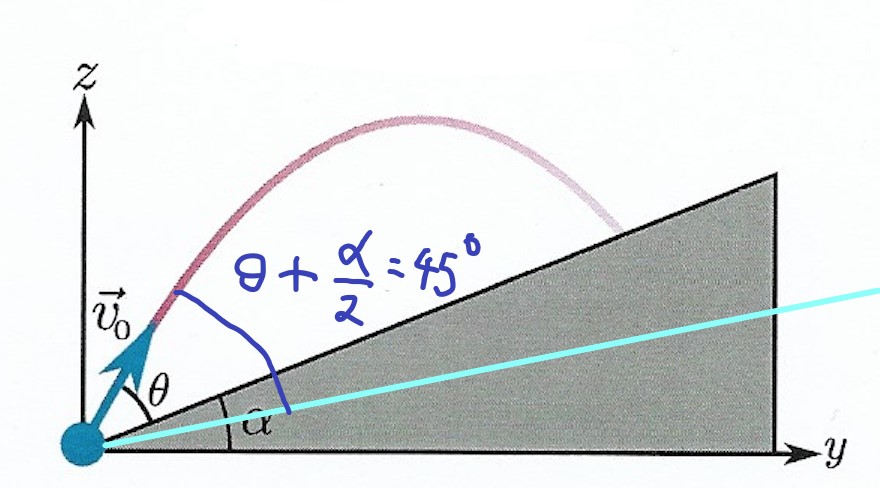
A Ball is thrown up an inclined plane (incline angle $\alpha$) with $\vec{v_0}$ being at an angle of $\theta$ with the inclined plane. I added a picture to show the situation 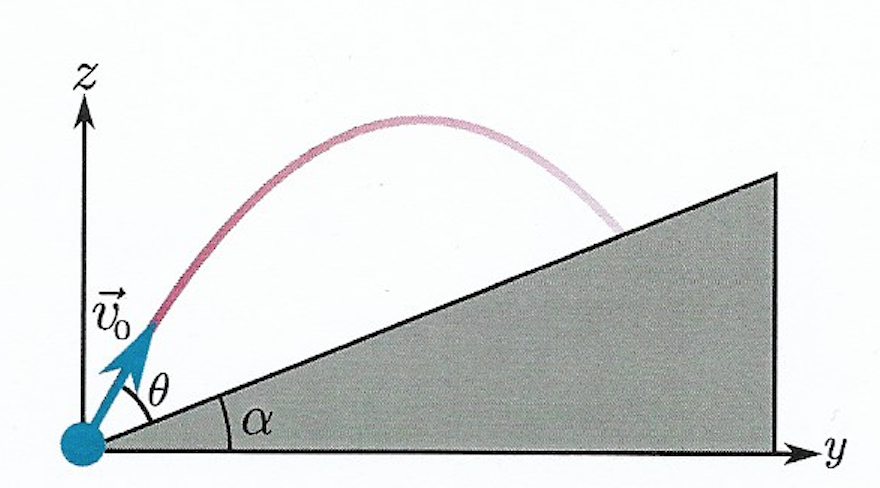
I already derived the angle of $\theta$ which maximises the distance on the inclined plane which turns out to be $\theta = \frac{\pi}{4} - \frac{\alpha}{2}$. This also seems to be correct when I compare it to other results on the internet.
However, is there an intuitive way for why this has to be the case?