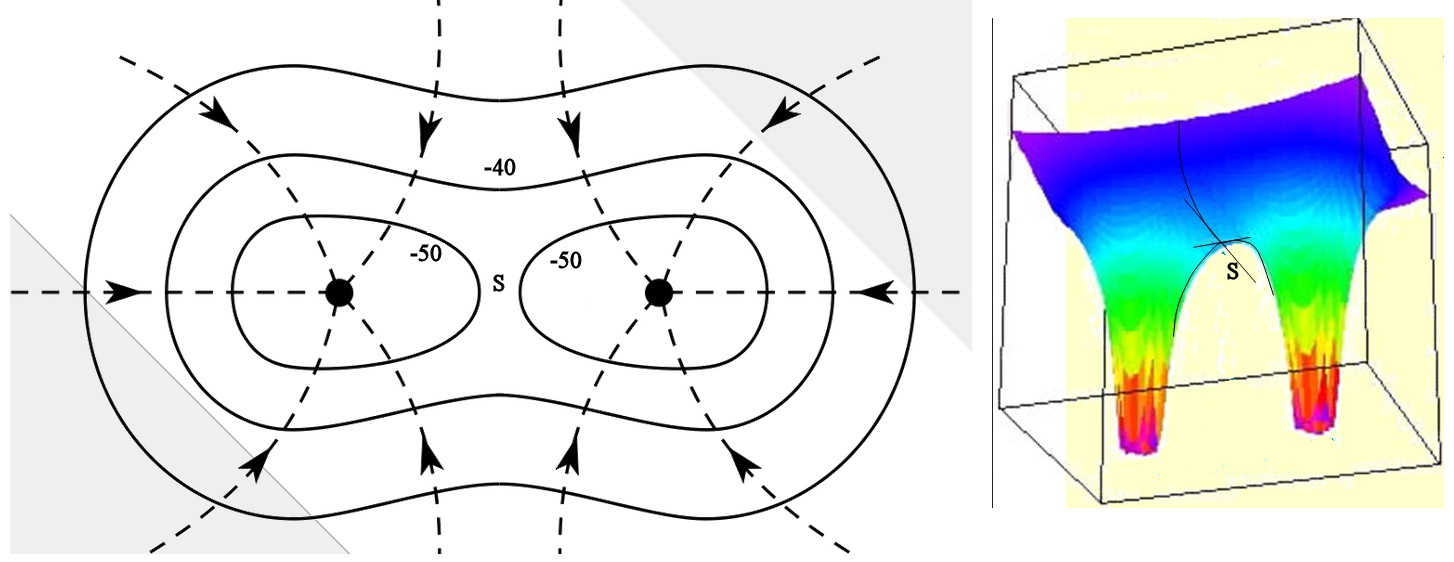
If you have 2 stars of mass $M$ and seperated by a distance $2R$, and a planet of mass $m$ is equidistant between them, the potential energy of the planet is given by:
$$E_p = \frac{-2GMm}{R}$$
This is the sum of the potential energy the planet has due to each star. However, considering that gravitational potential energy is just a measure of how much kinetic energy would be gained were the planet to fall towards the source of the field, shouldn't the potential energy be 0?
The planet is effectively stuck between the 2 stars. It will be at rest on the line joining the centres of the two stars, unable to move because the net force on it would be 0. Therefore, I do not understand why the net potential energy is not also 0.