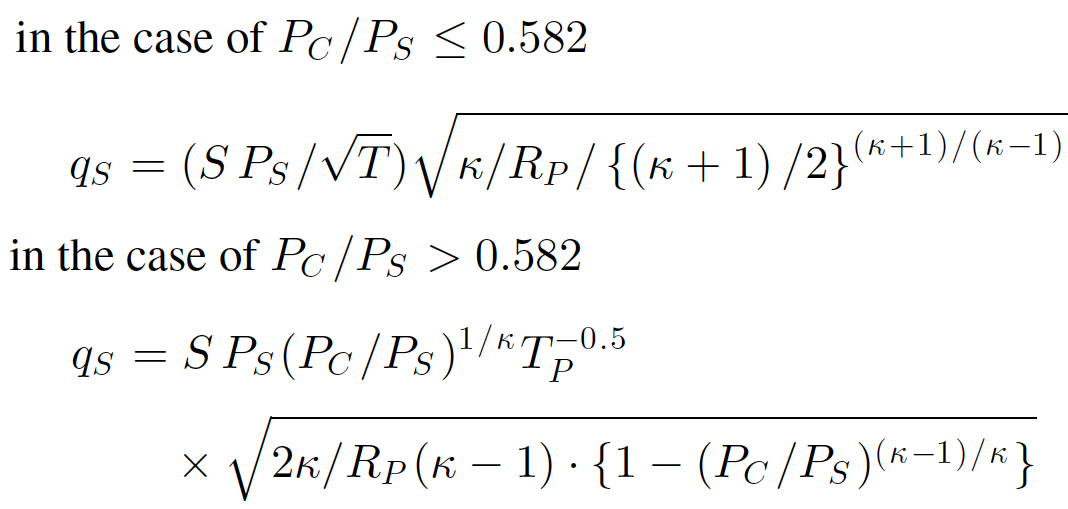Relationship between flow and pressure gradient in a one-dimensional compressible fluid

Question

Consider a one dimensional model (tube with diamter $D$) with a compressible viscose ($\mu$) fluid (e.g. air). further assumptions are

• conduction and radiation are negligible
• gravity's effect is negligible
• flow is one dimensional and uniform
• the flow is not steady-state so it is changing rapidly by time (e.g. Sod shock tube)
• gas is ideal $p=\rho \mathring{R} T$

based on this assumptions it is possible to write conservation equations of mass, momentum and heat. However, solving those PDEs is very difficult. I'm looking for empirical equations to approximate the mass flow dependency on pressure gradient:

$q= \rho \nu =f\left( \frac{\partial p}{\partial x},D,... \right)$

I have looked into the literature but what I have found is mostly for steady state flow in long tubes (e.g. Weymouth and Panhandle Equations). I would appreciate if you could help me find such an approximate solutions for the said assumptions.

P.S.1. I'm not interested in pressure loss. I have Darcy equation and its derivatives for that reason.

P.S.2. I was not sure what tags to attache, so let me know please if they are irrelevant.

P.S.3. I have found these equations from one article I'm reading:

But the paper has provided no reference or explanation where these equation came from, nor any name is mentioned.

Answer 1

I think I have figured it out where the equations above come from. They are not empirical equation but approximations generated directly from conservation law of energy. It is also not for a tube in particular but rather for any 1 dimensional flow like orifice with cross section change (Venturi effect). Please do not consider this as the complete answer to the question but just explanation of how the equations mentioned in the said paper are generated.

We will take a Lagrangian approach, so have a particle in mind. We have these further assumptions:

• process is reversible $\implies dS=\frac{dQ}{T}$, Where $S$ is entropy, and $Q$ is heat
• process is adiabatic so $dQ=0$, from first assumption it implies the process is isentropic too $\implies dS=0$

The energy conservation law implies ( "Fluid Power Control by Blackburn 1960" page 68):

$$h+\frac{v^2}{2}=k_1$$

where $h$ is the specific enthalpy and $v$ is speed. for ideal gas in isentropic process $h=c_P T$ :

$$ \implies c_PT+\frac{v^2}{2}=k_1$$

for adiabatic process in ideal gas the polytropic equation boils down to:

$$\frac{P}{\rho^\gamma}=k_2$$

where $\gamma=\frac{c_P}{c_v}$ is the heat capacity ratio (above noted as $\kappa$) . For dry air is SI units $\gamma=1.4$, $C_p=1004$ and $\mathring{R}=286.9$

$$\implies v=\sqrt{2\left(k_1-\frac{c_P\mathring{R}}{k_2^{\frac{1}{\gamma}}}P^{\left(1-\frac{1}{\gamma}\right)}\right)}$$

$$\implies \dot{m}=\left(\frac{P}{k_2}\right)^{\frac{1}{\gamma}}\sqrt{2\left(k_1-\frac{c_P\mathring{R}}{k_2^{\frac{1}{\gamma}}}P^{\left(1-\frac{1}{\gamma}\right)}\right)}$$

The number 0.528 comes from the equation for choked flow:

$$\frac{P_2}{P_1}=\left(\frac{2}{\gamma+1} \right)^{\left(\frac{\gamma}{\gamma -1} \right)}$$

However I still don't know where this equation comes from. there are two options:

• Considering that the speed of flow can't be more than the speed of sound ($c=\sqrt{\gamma \mathring{R}T}$ for air $c\approx340$)

• from $\frac{\partial \dot{m}}{\partial P}=0$

Answer 2

What you have written down (also in your answer) is a version of the fluid dynamic jump conditions. Those result from integrating the conservation equations (for mass, momentum and energy) over 1D over an arbitrary test volume, which gives a relation of the conserved quantities at the edges of said volume. Once you have those, the relation of the pre-to-postshock quantities (works also without shocks) is just a matter of solving the algebra.

In the fluid dynamics literature you can find this treated quite widely, see for example "Riemann solvers and numerical methods for fluid dynamics" by E. Toro, or you can easily find a number of university lecture by googling "shock jump conditions".

Just to clarify, a few things that you have mentioned, put into context:
* Venturi effect: results from the Bernoulli law, which is a 1D-integrated version of the momentum equation, aka just what you want to do
*e.g. Weymouth and Panhandle Equations: Never heard of, probably more specialized equations with boundary conditions for tubes
* Darcy equation: Darcy's law is used in soil physics, where the fluid is embedded in a matrix and therefore cannot build up a wave equation to communicate state changes. I would argue that using Darcy here is very wrong.