1
$\begingroup$

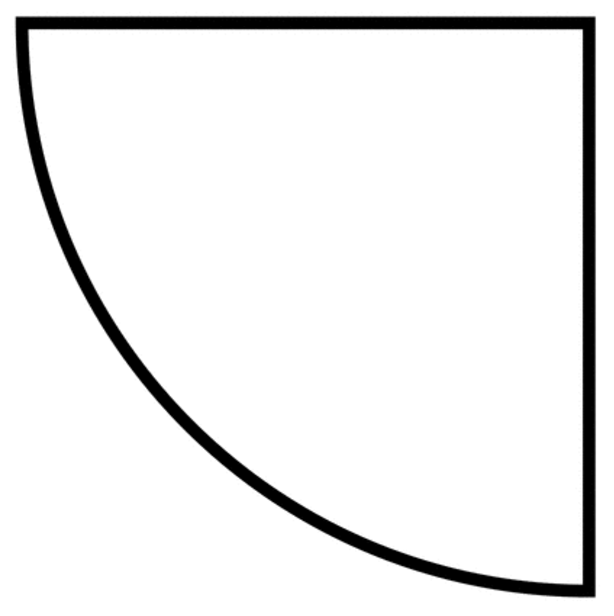
Imagine an object falling along a quarter circle of height $h$ meters ($h$ would also be the radius) and gravity $g$ meters per second squared. How long will it take for the object to travel the distance of the curve in terms of $h$ and $g$? (no friction, no rolling, the object is negligibly small compared to the quarter circle, and the object is stationary until $t = 0$.)

enter image description here

I was able to determine the following answer:

$${t(h, g)=\sqrt{\frac{h}{g}}\cdot1.854074...}$$

The only issue is that I have no idea where the magic 1.854074... number comes from. This was solved with brute force calculation and curve fitting, but I'm sure a proper solution to this problem will better explain the value.

Improve this question
$\endgroup$
0

3 Answers 3

Reset to default
2
$\begingroup$

As John Forkosh said in the comments this can be done in a similar way to the brachistochrone problem problem. But in this case I think this overly complicates things. Instead we can use the fact that we are on a circle to find the speed. From conservation of energy we have: $$\frac{1}{2}mv^2=mgy$$ where $y$ is measured from the top of the circle downwards. Putting this in terms of angular velocity: $$\frac{1}{2}mh^2 \dot \theta^2=mgh \sin(\theta)$$ $$\int \frac{1}{\sqrt{2\sin(\theta)}}d\theta=\sqrt{\frac{g}{h}}\int dt$$

Improve this answer
$\endgroup$
10
  • $\begingroup$ I've looked at the brachistochrone problem and the setup/integration seems complicated and difficult to translate to this problem. Also as a heads up, this is NOT a homework problem. That tag was added by someone else. This was just a curiosity of mine. $\endgroup$
    – Dan
    Commented Aug 13, 2017 at 5:09
  • $\begingroup$ Hey @Dan, what aspects are you having trouble with? Also the 'homework-and-exercise' tag is not just for things that have been set as a homework but for problems you find in e.g. text books instead of conceptual questions. $\endgroup$
    – Quantum spaghettification
    Commented Aug 13, 2017 at 5:13
  • $\begingroup$ I don't know what delta variables mean outside of fractions. Like ds/dt=v makes sense to me, but working with ds or dt outside of that is something I don't understand at all $\endgroup$
    – Dan
    Commented Aug 13, 2017 at 5:18
  • $\begingroup$ @Dan see my edit. $\endgroup$
    – Quantum spaghettification
    Commented Aug 13, 2017 at 5:27
  • $\begingroup$ I'll assume the limits of that first integral should be 0 to pi/2 and sure enough it gives 1.854074..., exactly what I was looking for. Thanks! $\endgroup$
    – Dan
    Commented Aug 13, 2017 at 5:35
0
$\begingroup$

My answer is quite late, but I hope someone will find it helpful.
In fact, we can use Newtonian mech to solve this problem.
We first decompose the gravitational force into two components--tangential and centripetal. The centripetal force does not change the magnitude of the velocity, so let's ignore it. Now we look at the tangential component of the gravity. $$ma_t=mg\cdot\cos\theta$$ we know that $a_t=R\ddot\theta$, so $$R\ddot\theta=g\cos\theta$$ $$\ddot\theta=\frac g l \cos\theta$$ (The equation above is similar to the equation of a SHM pendulum)
Now what we need to do is to solve this ODE. Since this is not easy to do, I will leave the answer like that.

Improve this answer
$\endgroup$
-1
$\begingroup$

I am generally averse to assist with anything resembling a homework problem but, in this instance, I think answering would be appropriate.

The question being asked is technically a wholly other question in disguise: the information presented defines a pendulum and you are being asked to find HALF the period.

The formula to find the complete period is: $$T ≈ 2\pi \sqrt\frac{L}{g}$$

Just remember that, in this instance, L and h are the same and you only want HALF the complete period.

Improve this answer
$\endgroup$
1
  • $\begingroup$ The formula $T\approx 2\pi \sqrt{L/g}$ is only valid in the case of small oscillations. This is far from true here - given that our 'pendulum' goes to the horizontal. $\endgroup$
    – Quantum spaghettification
    Commented Aug 13, 2017 at 5:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.