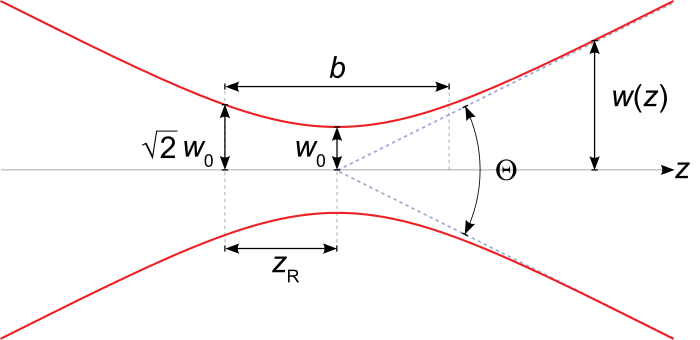
I'm trying to understand how Gaussian beams interact with matter. Maybe I have a conceptual issue, but is the beam in the image not propagating in the z direction? In that case, I want to understand what happens to the beam if it enters a new medium at say z = -2 (where z=0 at beam waist).
Specifically, I'd like to know how to calculate the new beam waist position of a Gaussian beam once it is enters (at right angles) a medium of different refraction index. Say I know width of the beam at the surface of the medium, how do I find out where the new beam waist is?
I've tried to apply the ray transfer matrix [1 0; 0 n] to the beam parameter transformation, but I don't get any new information from that. It seems reasonable to me that the new Rayleigh range would simply be multiplied by n - but why would this be the case?
Any help would be appreciated!
Thank you!