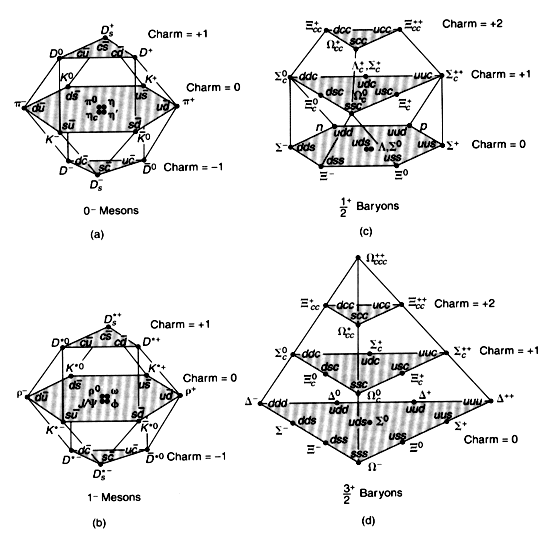
Gell-Mann's $SU(3)$ quark model is extremely successful at describing the bound states of the three light quarks $u,d,s$. The bound states fall neatly into the irreducible representations of $\mathfrak{su}(3)$. With the recent discovery of the doubly charmed baryon $\Xi_{cc}^{++}$ I have been thinking about how this "eightfold way" may be extended to include all six flavors. Is it as simple as extending the flavor symmetry group to $SU(6)$? I am a little rusty on my group theory, but if I remember correctly $SU(3)$ is not isomorphic to a subgroup of $SU(6)$. So how could this extension preserve the highly successful theory of $SU(3)$ for the lighter quarks?
2 Answers
SU(3) is a subgroup of SU(6): its generators span the 3×3 block of the 6×6 generators of the latter.
Yes, the eightfold way trivially extends to a notional 35-way of all six flavors, which is, nevertheless, largely useless.
You may, of course, build classification tables of such hadrons in a 5-dimensional space (rank of SU(6)), but with little logical benefit. People did build 3d pictures of the first 4 quarks' SU(4) when charm was discovered,
However, the whole point of the 8-fold way was that the lightest 3 quarks are lighter than the scale of QCD, ~200 MeV , which binds them together, so their masses could serve as small perturbations to a robust underlying pattern, explicitly broken (corrected) by small SU(3) violations.
For more flavors, the violations are, evidently, huge, and so your proposal might be a fool's errand.
Perversely, the 3 heaviest quarks, c,b,t, form a separate SU(3) of their own, as they are so heavy that you may transcribe across their differences, and treat the much lighter (scale-separated) QCD "glue" as invariant around them: "brown mud", in Bjorken's words, for example.
It is Yang-Mills theory that suggests the extension of QCD to SU(n) dimensions builds real space rotational state energies into the angular momentum quantization of electron, quark, and n-ternion states. That means that the weak decay is in reality a function of angular momentum and there is no neutrino. There is no neutrino. The effect of rotational symmetry group operators moving Hilbert space vectors in their real projection results in the emission of energy. This explains nuclear behavior in large nucleons, asymmetric magic numbered nucleons, and isotopes. Further relativistic corrections provide a dark matter connection to the frame dragging rotation. Again, more relativity implies the SU(n) frames create pseudo-forces. These may be the gluons or binding energies.