Finding eccentricity of orbit given speed, altitude and polar angle

Question

Problem

For a certain satellite the observed velocity and radius at v = 90° is observed to be 45,000 ft/sec and 4,000 n mi, respectively. Find the eccentricity of the orbit.

(Answer: e = 1.581)

How does one solve this problem without knowing what body the satellite is orbiting around or what the flight path angle is or other characteristics?

It is easy to find an example planet and altitude (μ) for which this is a simple circular orbit. If you assume it's a circular orbit, then $$a_c=v^2/r=\mu/r^2$$ $$\mu=v^2r=(45000 ft/s)^2*(4,000 n mi)=4.92*10^{15} ft^3/s^2$$

This makes me think this would have to be around earth, but that isn't clearly stated in the problem.

But even from there, you don't know the flight angle of orbit at 90° which doesn't allow us to find the angular velocity of the orbit. Knowing the answer is 1.581, we know it's a hyperbolic orbit and should either be leaving the planet or be coming into the planet just to leave but we don't know at what angle. It could be coming nearly straight down at earth.

Answer 1

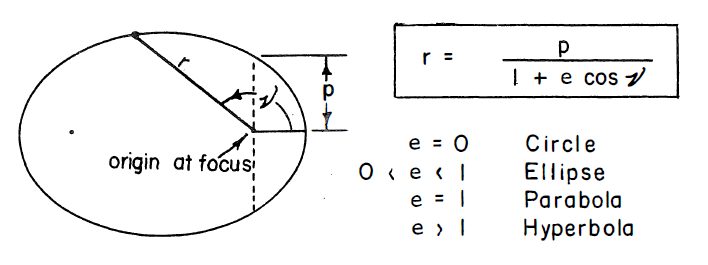Here is a snapshot from Fundamentals of Astrodynamics by Bate, Mueller, and White.

You do have to assume that you are working with the earth, while it is not mentioned in the problem.

You start by calculating the specific energy (ε) of the orbit given the velocities:

$$\epsilon=\frac{V^2}{2}-\frac{\mu}{r}$$

From there you calculate the semi-major axis (a) of the orbit:

$$\epsilon = -\frac{\mu}{2a} \therefore a = -\frac{\mu}{2\epsilon}$$

Next, you find the parameter (p) of the orbit:

$$r = \frac{p}{1+e\cos{v}}=\frac{p}{1+e\cos{90°}} = p$$

Finally, you can throw it all together into an equation from the definition of a conic section:

$$p = a(1-e^2) \therefore e = \sqrt{1-\frac{p}{a}}$$

When numbers are plugged in from the initial problem and from the characterization of earth, I got the final answer.