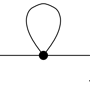
This is somewhat related to an earlier question I asked about the following diagram in $\phi^{4}$ theory:
I've been following these lecture notes by H. Kleinert and V. Schulte-Frohlinde.
Saying we're in $D$-dimensions and going to momentum space, the above diagram corresponds to the following: $$ - \lambda \int \frac{d^{D}p}{(2\pi)^{D}} \frac{1}{p^{2}+m^{2}} = - \lambda \frac{(m^{2})^{D/2}}{(4\pi)^{D/2}} \Gamma\left(1 - \tfrac{D}{2}\right) $$
The above is divergent for $D=4$, so we consider small $\epsilon$ for which $4-D=\epsilon$. We consider an arbitrary mass parameter $\mu$, and introduce a dimensionless coupling constant $g =\lambda \mu^{-\epsilon}$. The above then reads: $$ = - m^{2} \frac{g}{(4\pi)^{2}} \left( \frac{4 \pi \mu^{2}}{m^{2}} \right)^{\epsilon/2} \Gamma\left( \tfrac{\epsilon}{2} - 1 \right) $$ And performing a Taylor expansion about small $\epsilon$, we find that the above becomes ($\psi$ is the digamma function): $$ \approx m^{2} \frac{g}{(4\pi)^{2}} \left[ \frac{2}{\epsilon} + \psi(2) + \log\left( \frac{4 \pi \mu^{2}}{m^{2}} \right) + \mathcal{O}(\epsilon) \right] $$
$\ $
I am interested in getting the contribution from the above in position space, in the massless limit $m \to 0$. I have two questions:
In the lecture notes above, it says that the above diagram is IR-divergent in the limit that $m^{2} \to 0$. What does this mean, precisely?
If we have an incoming momentum $k$, and the the above diagram corresponds to a function $\tilde{F}(k)$ in momentum space, then in position space we have a contribution given by $F(x_{1},x_{2}) = \int \frac{d^{4}k}{(2\pi)^{4}} e^{- k \cdot (x_{1} - x_{2})} \tilde{F}(k) $. How do I do this in the framework of dimensional regularization? Can I even do this? Where is the dependence of $k$ in the above that I can even do the integral, and then how do I complete that integral?
At the end of the day, I am trying to understand the nature of the divergence for this diagram in position space (in the massless case).