What is the direction of the normal acceleration on an inclined plane?

Question

I am very confused about the solution that my Professor has provided to an apparently-simple exercise.

For the following box rotating around in an inclined plane, I am asked to find the minimum velocity at the highest point in the trajectory to have circular motion.

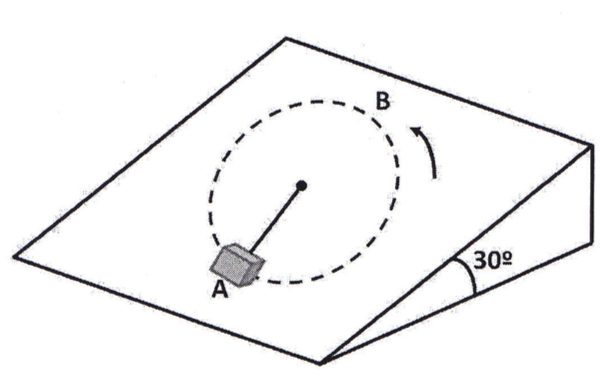
I know this implies that the normal acceleration must be greater than zero, but when I solve this exercise, I come up with the forces equilibriums:

$$\sum F_y = N\cos(30º) - mg - Tsin(30º) = 0$$ $$\sum F_x = T\cos(30º) - mg - Nsin(30º) = m\frac{v^2}R$$This is taking the traditional X and Y axes.

However, my Professor takes the Y axis perpendicular to the plane and the X axis parallel to it, and comes up with:

$$\sum F_x = T + mgsin(30º)= m\frac{v^2}R$$

Shouldn't he be multiplying the normal acceleration by the $sin(30)$ for this to be valid? I do not understand why he takes the direction of the normal acceleration to be parallel to the plane.

Answer 1

It's important to specify what axes you are using for your frame of reference, since choosing and using a different frame can simplify the question immensely.

So, start by defining a frame of reference with origin at the centre of the circular motion, with the positive $Z$ axis perpendicular to the inclined plane, and thus the $X-Y$ plane in the surface of the inclined plane.

Assume that the moving box is located at the point $B$; the point in its circular motion farthest up the inclined plane, and that it is continuing in its circular motion.

Immediately, we know the total force that must be acting on the box from B to the centre; it's just the required centripetal force.

Moreover, we know that the only real forces acting on the box in the plane of the incline are the tension in the string, and the down-slope component of the force of gravity. The normal force (perpendicular to the inclined plane) can be ignored; it does not act in a direction to affect the circular motion.

So just equate the real force acting down the slope to the force needed:$$T+mg \sin(30)=\frac{mv^2}{R}$$ and let $T$ go to zero...