Motion of a mass attached to a rotating spring

Question

Consider a spring of spring constant $k$ and natural length $l_0$. One end of the spring is fixed to the origin $O$ and the other end is attached to a mass $m$ which is free two move frictionlessly in a 2 dimmensional plane. Define $\Omega=\sqrt(k/m)$

Let $(r,\theta)$ be the polar coordinates of the mass. Assume the initial conditions are such that $r(0)=r_0>0, \theta=0, r'(0)=0, \theta'(0)=\omega_0 \ge 0$. That is, the particle starts at some point on the positive $x$-axis and its initial velocity parallel to the $y$-axis.

I would like to study the motion of the mass. Ideally, i would like to express it's motion in polar coordinates, if it's possible. If not, I would like to see if there's still some interesting information we can extract from the problem, for example the existence of closed and closed,periodic orbits.

We already know the special case $\omega_0=0$ is the classic harmonic oscillator. Also, it's easy to show that if $\omega_0=\omega_{0,circ}=\Omega\sqrt{1-l_0/r_0}$ then the mass moves in a circular orbit of radius $r_0$ and angular frequency $\omega_0$. Using the effective potential energy we easily show that this orbit is stable.

In the general case, the orbits of the mass about $O$ are always bounded, since the total energy is positive:

$E_{tot}=\frac{1}{2}mv^2 +\frac{1}{2} k(r-l_0)^2$

There are two cases to distinguish: the spring starts out stretched $r_0>l_0$ (I) and the spring starts out compressed $r<l_0$ (II).

In case I, if $\omega_0<\omega_{0,circ}$ then the spring begins compressing immediatley and mass is pulled towards the origin; the mass moves past the origin and then reaches some maximal distance as the spring stretches, etc. If $\omega_0>\omega_{0,circ}$ then the movement is similar except the spring begins by stretching. I wrote a python simulation of the particle's motion. Below is an image from the an example Case I motion (the origin is the center of the window).

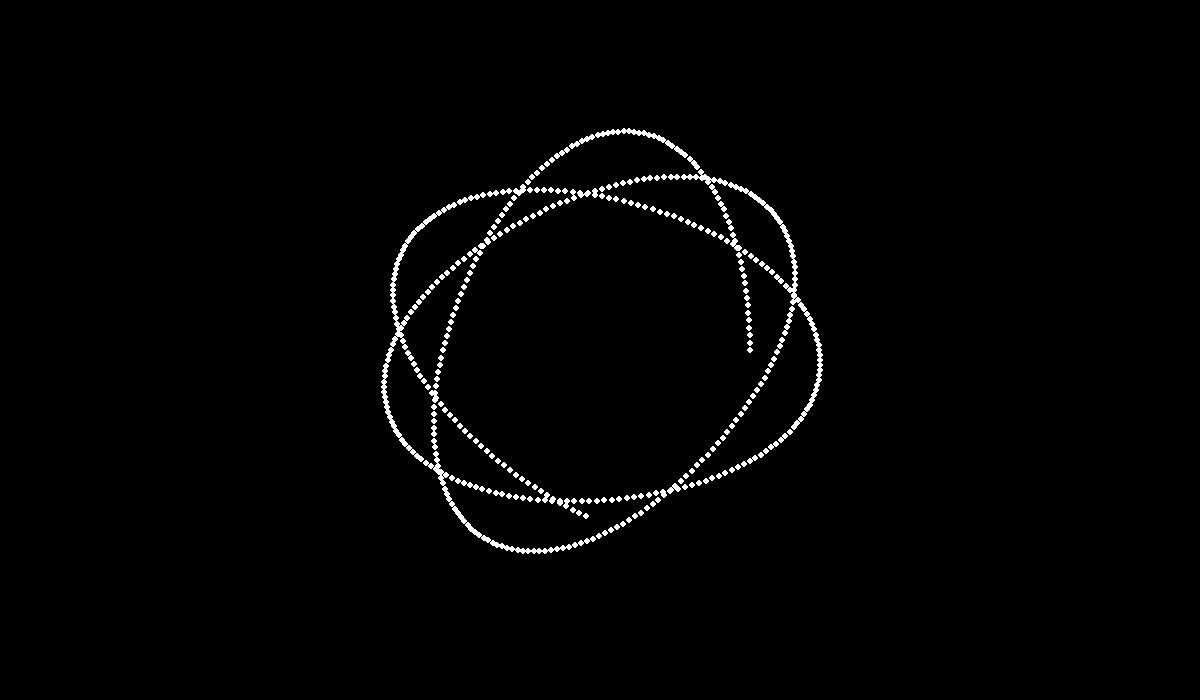
In case II, the spring starts decompressing and the mass moves away from the origin, stretching the spring until some maximal distance is reached; then the spring begins compressing and them ass is pulled back towards the origin, etc. See below:

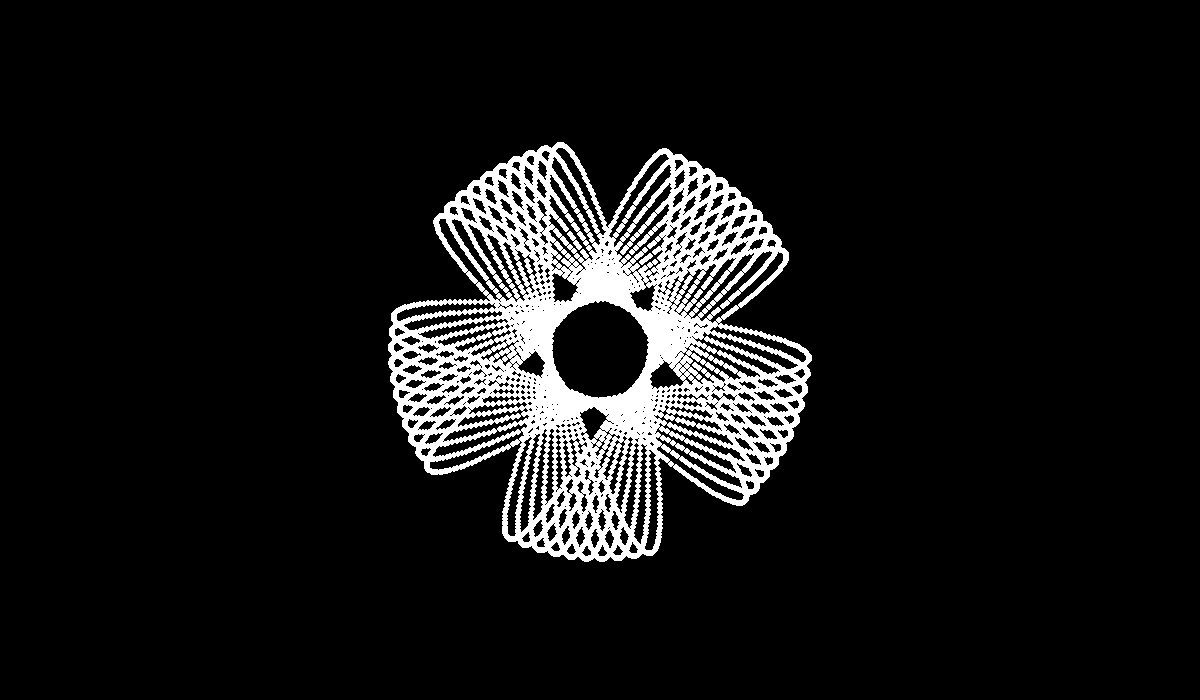
If the motion from the above simulation continues, the orbit is not closed. I don't know is this representative of the real motion or if it's due to inaccuracies in the simulation. In any case, letting the simulation continue gives a pattern which seems to be filling the interior of an annulus (the orbit is bounded between the minimum and maximum radii) so the orbit seems to not be closed. See below:

By standard methods I have derived the following differential equation relating $r$ and $\theta$:

$(\frac{dr}{d\theta})^2=\frac{2mE}{L^2}r^4-r^2-\frac{mk}{L^2}r^4(r-l_0)^2$

where $E$ is the total energy (which is conserved) and $L=mr^2\omega_0$ is the angular momentum (also conserved). I don't whether this is solvable, but it doesn't look to be...

Answer 1

The easiest way to solve this is Lagrangian mechanics. The Lagrangian is defined as $L=T-V$, where $T$ is the kinetic energy and $V$ is the potential.

Here, the only $T$ comes from the mass' motion. $T=\frac{1}{2}mv^2$, and it's easiest to write $v$ in terms of polar coordinates. $v^2=\dot{r}^2+r^2\dot{\theta}^2$

So, $T=\frac{1}{2}m(\dot{r}^2+r^2\dot{\theta}^2)$

Now, all potential energy comes from the spring. $V=\frac{1}{2}k(r-l_0)^2$

The full Lagrangian becomes $$ L=\frac{1}{2}(m(\dot{r}^2+r^2\dot{\theta}^2)-k(r-l_0)^2) $$

Ok, now what? In order to extract useful information from the Lagrangian, we use Lagrange's equations:

$$ \frac{d}{dt}(\frac{\partial L}{\partial \dot{q}})=\frac{\partial L}{\partial q} $$

where $q$ is a "generalized coordinate". Here, we take $q$ to be $r$ and $\theta$ separately, to get 2 equations out of this.

$$ \frac{\partial L}{\partial r}=\frac{1}{2}(2mr\dot{\theta}^2-2k(r-l_0)) $$ $$ \frac{\partial L}{\partial \dot{r}}=m\dot{r} $$ $$ \frac{d}{dt}(\frac{\partial L}{\partial \dot{r}})=m\ddot{r} $$

This gives us the full equation for $q=r$:

$$ m\ddot{r}=\frac{1}{2}(2mr\dot{\theta}^2-2k(r-l_0)). $$

For $q=\theta$, $$ \frac{\partial L}{\partial \theta}=0 $$ $$ \frac{\partial L}{\partial \dot{\theta}}=mr^2\dot{\theta} $$ $$ \frac{d}{dt}(\frac{\partial L}{\partial \dot{\theta}})=m(2r\dot{r}\dot{\theta}+r^2\ddot{\theta}) $$

So, the for the full equation, $$ m(2r\dot{r}\dot{\theta}+r^2\ddot{\theta})=0. $$

With those two equations, we can solve for each for $\ddot{r}$ and $\ddot{\theta}$ respectively, obtaining some equations of motion.

Note that $\frac{\partial L}{\partial \theta}=0$; this is actually a statement that the angular momentum is conserved! This conservation law is a form of Noether's theorem. The symmetry here (that the Lagrangian is invariant in $\theta$) corresponds to a conserved quantity.

Hope this helps!