Calculating focal length for contracting a Gaussian beam

Question

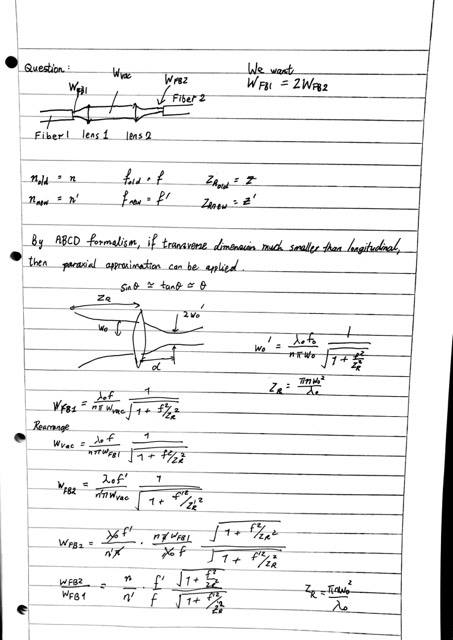
A typical experiment problem, I have to choose two lens such that I can couple laser from one fiber of diameter D1 into another fiber of diameter D2. The fiber one has twice the diameter of the second fiber, i.e. D1 = 2D2. It is clear that two focal lengths must be different to achieve this, I tried to calculate this by meeting the condition that the waist of Gaussian beam in first fiber must be twice of that second fiber. The problem can be seen in the schematics below. I have attempted to calculate it, but i am not sure if I am doing it correctly. My attempt: applied paraxial approximation to Gaussian beam, and use ABCD formalism for both lens. I quoted the formula for focusing a beam using a lens, I rearranged that formula to use it for collimation, and use the same formula for the second lens. And I arrived the steps shown below.

n,n' : refractive index of the medium, f,f' : focal lengths Zr,Zr': Rayleigh range

Download and zoom in if it is not too clear. The formula are quoted from this document http://www.mpl.mpg.de/personal/njoly/pdf/Laser/chap2.pdf

Answer 1

My method is proven to be correct, but too complicated. An easier method is to consider the waist with the beam divergence angle, $\theta=D/f$. Since the collimation beam diameter in between two lens must be the same, Only the the focal length and divergence angle must be different. By comparing the divergence angle on both sides, one angle is twice of the others, so if the beam divergence of the left hand side is twice of the right hand side, the focal length must be twice of the right hand ones.