-1
$\begingroup$

In one exercise I was asked to find the general solution for a free particle but in integral form(!!)

I'm supposed to get 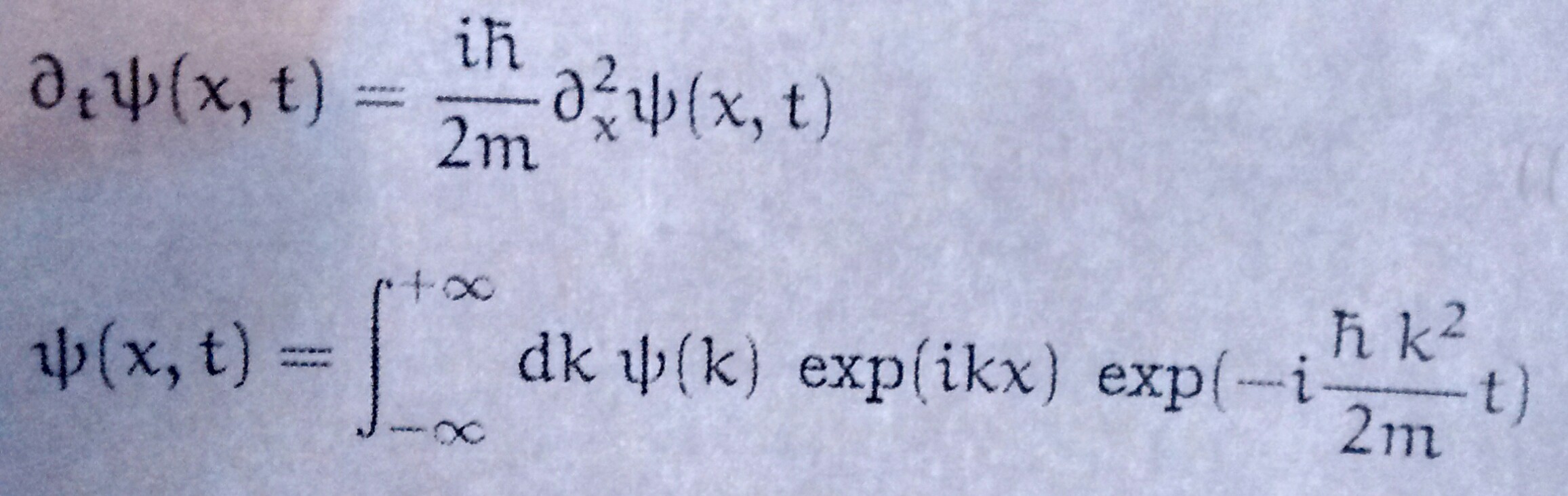this but I have no idea how to get there!

Looking around I found quite a bit of sites/videos/lecture about how to solve the free particle problem but not once I have found this form of the solution :s

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Hint: This integral form is derived by the use of the Fourier transforming this equation by the variable x. A Fourier transformed quantity $\phi(k,t)$ can be transfomed into its original quantity $\psi(x,t)$ by the formula:

$\psi(x,t) = \int_{-\infty}^{\infty} dk \frac{e^{ikx}}{2 \pi} \phi(k,t)$.

Improve this answer
$\endgroup$