Sorry if this is a trivial question.
Why does gravity act at the center of mass?
If we have a solid $E$, shouldn't gravity act on all the points $(x,y,z)$ in $E$? Why then when we do problems we only only consider the weight force from the center of mass?
Suppose I have a collection of n vectors $x_i\quad \forall i\in(1,n), i\in \mathbb{Z}$ such that the corresponding masses at each $x_i$ is $m_i$. This is your body $E$ and if the total mass of your body is $M$, then $$M=\sum_{i=1}^{n}m_i$$ In that case, if $E$ is subjected to a uniform acceleration field $\vec{g}$, as specified in the answer above, then the net force acting on the body is $$F=\sum_{i=1}^{n}m_i \ddot{x}_i$$ But, the force on the entire body would be $F=Mg$. Let there be a point $X$ on the body such that I can say that $\ddot{X}=g$, Then I can write $F= M\ddot{X}=\sum_{i=1}^{n}m_i \ddot{x}_i$.
From this you can interpret that $$\ddot{X}=\frac{\sum_{i=1}^{n}m_i \ddot{x}_i}{\sum_{i=1}^{n}m_i}$$ And the centre of mass is defined as $$\begin{equation}\label{com} x_{com}=\frac{\sum_{i=1}^{n}m_ix_i}{\sum_{i=1}^{n}m_i} \end{equation}$$ Since the body $E$ has constant mass, you can get the definition of center of mass above by simple integration.
This is only true in a uniform field, and this is why: the center of mass is the average mass weighted position of an extended object. Meanwhile, the total gravitational force is the sum over all parts of the object, weighted by mass: the mass weighted integrals for the average and the sum are the same. In reality the center of gravity differs from the center of mass, since a variable gravitational field changes the later sum over parts. If you don't believe me, look at NASA's SRTM data, in which a radar antenna on a 200 ft boom in the Space Shuttle made a radar map of Earth--since the Earth's field is not uniform, the center of gravity was lower than the center of mass, and the thing kept rolling (imagine a dumbbell at 45 degrees)--thrust corrections left the boom oscillating, and that can be seen as a ripple in the map elevation.
Consider two situations as shown below.
One (left diagram) where two masses are in a non-uniform gravitational field and the other (right diagram) where the two masses are in a unifgorm gravitational field.
In both cases the centre of mass is midway between the two masses $M$.
In the non uniform gravitational field the gravitation attraction on the mass closest to the large mass $W$ is bigger than the gravitational attraction on the other mass $w$, so the centre of gravity of the two masses is at position $G$ which is not midway between the two masses.
In the uniform gravitational field the gravitational attraction on the two masses $W$ is the same for the centre of gravity of the two masses is midway between them $G$ which is the same as the position of the centre of mass.
To be sure that the centre of mass and the centre of gravity are the same point the masses must be in a uniform gravitational field.
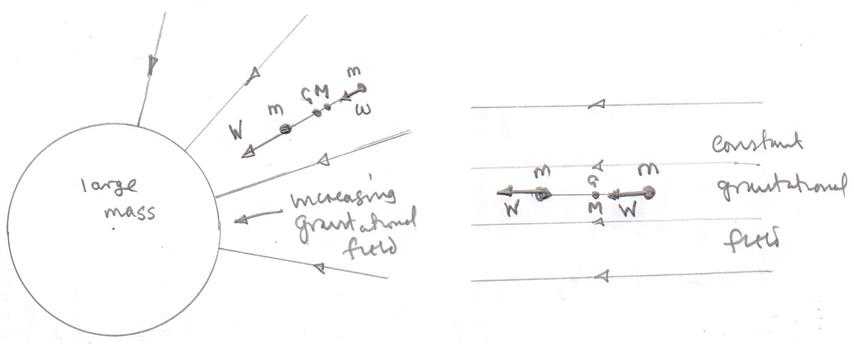
The only time we need to know where a force acts is when we are calculating a torque. For contact forces, it is clear that the force acts at the point of contact. But for a force like gravity, that acts at a distance, it is less clear.
In reality, a rigid object is made up of many particles, and there is a small gravitational force and torque on each of them. When we only care about acceleration we only need the sum of all these forces, which is $\vec{F}_{tot} = \sum_i m_i \vec{g}= M\vec{g}$. But what about the torques?
We would like to pretend that this total gravitational force acts at a single point for the purpose of calculating torque. Is there a point $\vec{x}_{cg}$ such that $\vec{x}_{cg}\times \vec{F}_{tot}$ gives the same total torque as summing up all the small torques?
If we do sum up all the torques we find $\vec{\tau}_{tot} = \sum_i \vec{x}_i\times (m_i\vec{g}) = \left(\frac{1}{M}\sum_i m_i \vec{x}_i\right) \times (M\vec{g})$. This tells us to call $\vec{x}_{cg} = \frac{1}{M}\sum_i m_i \vec{x}_i$ the center of gravity, and if we pretend that the total force of gravity acts at this point, it will always give us the right answer for the gravitational torque. Finally, we notice that it happens to have the same form as the definition of the center of mass!
However! If you do the calculation yourself you might notice that if $\vec{g}$ varies from particle to particle then this derivation does not work. In this case the center of gravity is not actually well defined. There may be no $\vec{x}_{cg}$ that does what we want, and even if there is it is not unique, except in a few special cases.
In actuality gravity does act on all parts of a mass independently. For a solid or liquid the parts then act on each other. And if the size of the mass is small compared to the non-linearity of the gravitational field, the "mass effect" is an average of all the independent effects, hence you can model it as occurring "at the center of gravity".
On the other hand, if the size of the object is large compared to the non-linearity of the gravitation field, one would need to do an integral of the mass across the field to take into account what would be called "tidal effects". A very long object falling into a black hole would be pulled apart as the stress from the different forces across the object interact. This is easier to understand if one thinks of the long object as being a stream of water (very little tensile strength)
Gravity does pull in each and every particle in the object.
If you hold the object in one random point, then gravity pulls in the particles to the left and in the particles to the right of this point. It makes a torque at each of these particles. If there is more total torque in the left side than in the right, then the object rotates counterclockwise.
Choose another point and the torques are spread differently. Only at one specific point do the left and right side torques exactly balance out. We give this point a name: Centre of mass.
A force acting on the Centre of mass does not make the object rotate, since it creates no torque around this already balanced point. Since a free falling object pulled in by gravity only does not rotate, it makes sense to say that the gravitational pull in each particle averages out to be one big gravitational pull in the Centre of mass - especially because we want to/have to simplify the gravitational force into one force at one point to make things easier to work with than many, many tiny forces in each and every particle.
Gravity acts on all parts of the body that have mass not just the COM. However the vector for which you calculate the effect of gravity can be applied between the COM of different bodies because mathematically it reduces to just the calculation between COMs.
