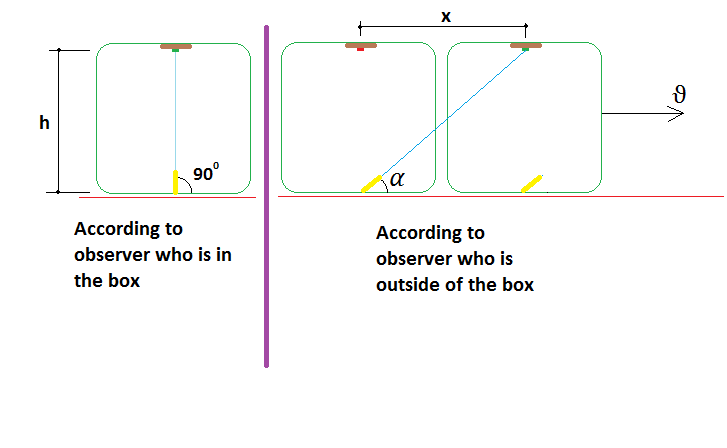
I always see this picture for proof of the time relation between frames that moves relatively constant speed in the special theory of relativity.
The time for the observer who is in the box which travel at constant speed $V$.
$$ t'=\frac{h}{c} \tag 1$$
The time for observer who is outside of the box.
$$ t=\frac{\sqrt{x^2+h^2}}{c} \tag 2$$
$$ t=\frac{x}{V} \tag 3$$ and the time relation between observers is $$ t'=t \sqrt {1-\frac{V^2}{c^2}} \tag 4$$ Everything is Ok till here.
According to outside observer, the person who adjusts the laser gun (yellow drawn in picture) angle in the box must change the angle ($\alpha$) as shown in right side of the picture if the light is not affected from the speed of the box as the special theory of relativity claims. However, According to inside observer who adjust the laser angle, the angle should be exactly $90^0$ because the inside observer will not understand that the box moves.
How does the special theory of relativity explain this dilemma?
(Please assume that the sensor on top is very small and the laser has very focused slim light beam and the box is quite speedy otherwise the angle always would be very near $90^0$ and the angle cannot be detected for low speeds. )